Analogous to the tides of Earths oceans, do the Moon and Sun cause our atmosphere to bulge in what could be described as a low and high tide?
-
3$\begingroup$ possible duplicate of Does the moon affect the Earth's climate? $\endgroup$– user10851Commented Aug 22, 2015 at 20:26
-
8$\begingroup$ @ChrisWhite The answers posted to that question are vague and completely lacking in physical analysis. I would like to hear what a true expert has to teach us about this subject. Also, that question is improperly titled or asks more than one item. Mine is focused and will be search engine friendly. $\endgroup$– AlexCommented Aug 22, 2015 at 20:51
-
$\begingroup$ Very nice question Alex, best of luck with it, does a solar eclipse enhance the effect? $\endgroup$– user81619Commented Aug 22, 2015 at 21:05
-
4$\begingroup$ This is not a duplicate question. The supposed duplicate asks about the Moon; this question asks about the Moon and the Sun. The Sun is dominates over the Moon by more than an order of magnitude in terms of contribution to atmospheric tides. $\endgroup$– David HammenCommented Aug 22, 2015 at 22:45
3 Answers
The differential force of gravity on the atmosphere works the same as it does for the rest of the earth (the oceans etc). However, moving the equipotential surface by a few m will be almost undetectable on the atmosphere, since the density of the atmosphere decreases so gradually – over many km. Contrast this with the surface of the ocean, which is crisp.
So while it might be theoretically possible to look for small changes in the height of an isobar of, say, $10^4\,\mathrm{Pa}$, I don't think that it will be possible to measure such a change in practice.
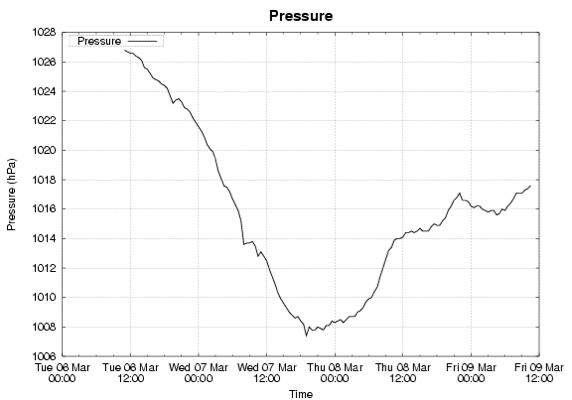
See for example this graph from the Australian weather service showing pressure changes over four days. The units on the left are $\mathrm{hPa}$ – you expect tidal variations to be much smaller. It may take a while (many cycles) to pick out the lunar variations - although I am sure it has been done.
There is a thing called "lunar atmospheric tides" - see Wikipedia which describes the math behind this. And it describes it as "weak".
So the short answer is "yes".
For a good (27 page) review of the subject, see this 1979 article by Lindzen
The introduction of that article states:
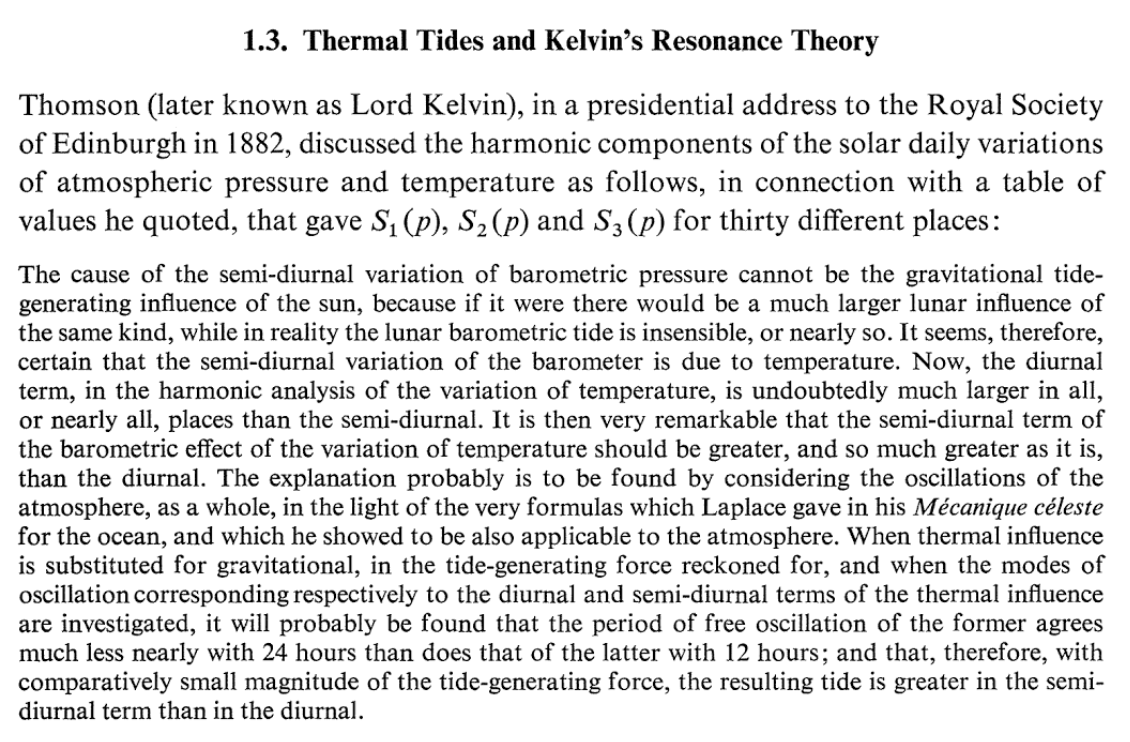
1 INTRODUCTION
Atmospheric tides refer to those oscillations in the atmosphere whose periods are integral fractions of a lunar or solar day. The 24-hour Fourier component is referred to as a diurnal tide, the 12-hour component as a semidiurmal tide. The total tidal variation is referrred to as the daily variation. Although atmospheric tides are, in small measure, gravitationally forced, they are primarily forced by daily variations in solar insolation.
So – the main cause of daily variation is solar heating. There is a (much) smaller component due to gravity:
... atmospheric tides are, in small measure, gravitationally forced...
-
5$\begingroup$ I don't own the latest gadgets, but according to Randall Munroe, "If your phone has a barometer in it, as a lot of new Android phones do, you can download an app and actually see the pressure difference between your head and your feet." So apparently ~20 Pa sensitivity has become commonplace. $\endgroup$– user10851Commented Aug 22, 2015 at 21:09
-
6$\begingroup$ @ChrisWhite of course you can measure a tiny pressure change - the question is how you distinguish lunar variations from all the other factors that influence pressure - there is a lot of churn in the atmosphere. $\endgroup$– FlorisCommented Aug 22, 2015 at 21:17
-
3$\begingroup$ I would still suspect that atmospheric motion caused by the moons gravity differential would be much larger (in volume) than that of the oceans. But is it clear that such motion would necessarily show as a pressure change? After all, the extra air above a given point on the surface is partially "supported" by Moon's gravitational pull. $\endgroup$ Commented Aug 22, 2015 at 21:59
-
1$\begingroup$ The very first line of your answer seems wrong to me. Since water is a far denser fluid than air, the tide height difference of air shouldn't be a few meters but far more. And if the equipotential surface in atmosphere is not moving significantly enough, then simply the tide is not caused by the moon. $\endgroup$– user124734Commented Jul 28, 2016 at 4:59
-
1$\begingroup$ @Floris: I didn't say anywhere that EPS is subjected to different laws. Can you please elaborate though on how the EPS distortion of water and air will be comparable in presence of same gravitational force? $\endgroup$– user124734Commented Jul 29, 2016 at 0:29
Analogous to the tides of Earths oceans, do the Moon and Sun cause our atmosphere to bulge in what could be described as a low and high tide?
The answer is yes, if you generalize beyond gravitation. Sunlight heats the atmosphere, and this causes atmospheric tides. The two dominant effects are absorption of visible and near infrared sunlight by water vapor in the troposphere and absorption of ultraviolet by ozone in the stratosphere.
The article by Lindzen cited in Floris' answer says exactly that. Atmospheric tides are caused primarily by solar heating rather than by gravitation. They are still "tides", however.
-
1$\begingroup$ Absolutely correct that variation due to solar heating exists - not sure that is a "tide" in the sense the OP intended. The article I linked mentions that gravitational tides exist (with a roughly 12-hour period), but they are MUCH weaker than the (diurnal - 24 hour cycle) variation due to solar heating. I pulled out the relevant quote and added it to my answer $\endgroup$– FlorisCommented Aug 23, 2015 at 2:34
-
$\begingroup$ @Floris You would think that, being forced by solar heating, the atmospheric tides would be diurnal. But they are semidurnal. The solar heating signal is basically a truncated sinusoid, being zero all night, and thus has a large semidurnal component. And in terms of the effect on atmospheric pressure, especially in the tropics, this semidiurnal component dominates. $\endgroup$– Ben51Commented Jan 23, 2018 at 18:26
-
$\begingroup$ @Ben51 are you referring to the fact that from a Fourier transform perspective a sinusoid has a second harmonic? $\endgroup$– FlorisCommented Jan 24, 2018 at 0:00
-
$\begingroup$ @Floris Yes! (Well, not a full sinusoid, of course, but rather a truncated sinusoid, with all the negative lobes chopped off). There is quite a lot of energy in the 12-hour harmonic, and that is what the tropical atmosphere responds to. Fair-weather barometric pressure records from the tropics are mostly just clean 12-hour oscillations. $\endgroup$– Ben51Commented Jan 24, 2018 at 3:44
-
$\begingroup$ @Floris I added a temporary answer below to show you what I mean. $\endgroup$– Ben51Commented Jan 24, 2018 at 4:05
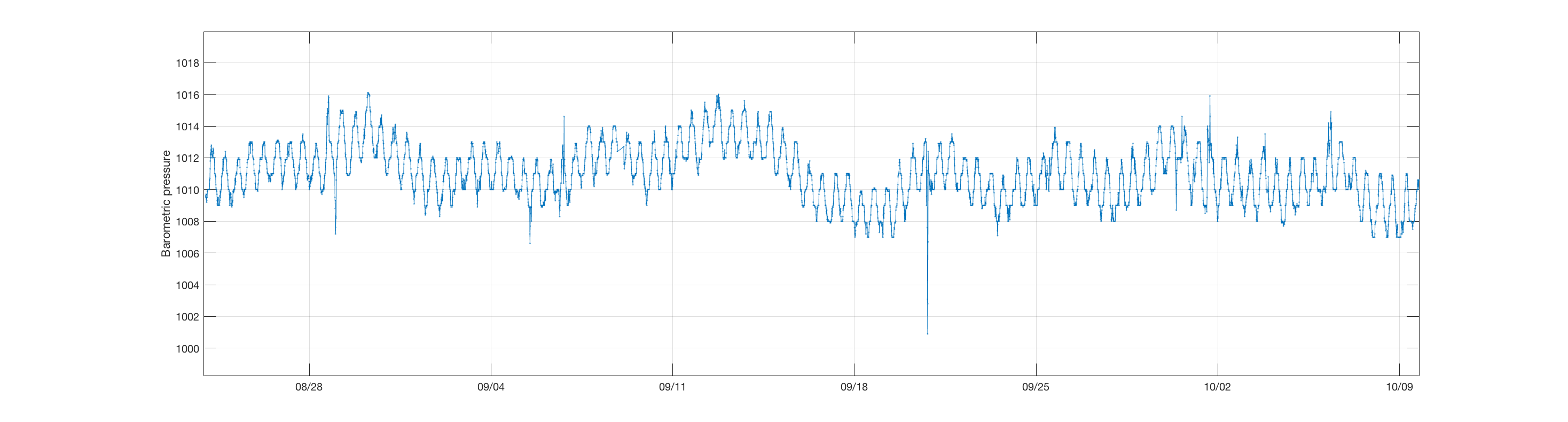
This isn't really an answer, and I will take it down shortly, just want to put it here as a demonstration of the clear dominance of the 12-hour constituent in the atmospheric tides in the tropics (can't put images in comments). The pressure record comes from last year in the Eastern tropical Pacific. There's a fair amount of variability on weekly timescales, and the 12-hour oscillation stands out, but you can't really make out a 24-hour component at all.
So even though the tides are driven by solar heating, which certainly sounds like it would mean they'd follow a daily cycle, they actually have a half-day cycle.
-
$\begingroup$ This is very surprising. Do you have a link to the source of this material? For one thing, the lunar tidal force is stronger than the solar one - yet the cycles shown here are exactly 12 hours long, not 12 hours and 25 minutes. Does a rapid cooling/heating at sunrise/sunset account for this (changing the density of the atmosphere due to humidity?) $\endgroup$– FlorisCommented Jan 24, 2018 at 4:19
-
$\begingroup$ Honestly I don't fully understand the mechanism. I had it beaten into my head that it was a heating effect, not a gravitational one, which I don't question. As for why there's a stronger response to the 12-hour forcing than the 24-hour, I always figured it was a resonance thing. The data is mine, there's no link to it, but I have other examples, and it shouldn't be hard to find comparable ones online. $\endgroup$– Ben51Commented Jan 24, 2018 at 4:24
-
$\begingroup$ Does the wind pick up mornings and evenings? The flatness of the over all curve (total pressure range) is astonishing. Where did you measure this? I know many islands have wind in the morning and evening (because of the heating/cooling effect of the water vs the land) - Bernoulli provides the corresponding pressure change. $\endgroup$– FlorisCommented Jan 24, 2018 at 5:14
-
$\begingroup$ This is at 10 N, 125 W, in the middle of the ocean sort of halfway between Hawaii and Central America. There are maybe barely discernible peaks in the spectrum of wind speed at day and half day periods, but you certainly don't see anything jump out at you when you look at a timeseries. I chose a pretty quiet period to emphasize the tides. There are bigger pressure fluctuations sometimes. $\endgroup$– Ben51Commented Jan 24, 2018 at 5:20
-
$\begingroup$ OK - so no effects due to differential heating as there is no land nearby?. Interesting - I can’t explain it. $\endgroup$– FlorisCommented Jan 24, 2018 at 5:31