Mathematical demonstration
It's straightforward to see why this happens if you use a bit of linear response theory.
Consider a generic damped harmonic oscillator.
There are three forces, the restoring force $F_\text{restoring} = - k x(t)$, the friction force $F_\text{friction} = - \mu \dot{x}(t)$, and the driving force $F_\text{drive}(t)$.
Newton's law says $F(t) = m \ddot{x}(t)$ which gives
$$-k x(t) - \mu \dot{x}(t) + F_\text{drive}(t) = m \ddot{x}(t) \, .$$
Dividing through by $m$ and defining $\phi(t) \equiv x(t)/m$, $\omega_0^2 \equiv k/m$, $2 \beta \equiv \mu/m$, and $J(t) \equiv F_\text{drive}(t)/m$, we get
$$ \ddot{\phi}(t) + 2\beta \dot{\phi}(t) + \omega_0^2 \phi(t) = J(t) \, .$$
This is a nice general form of the damped-driven harmonic oscillator.
Writing $\phi(t)$ as a Fourier transform
$$\phi(t) = \int_{-\infty}^\infty \frac{d\omega}{2\pi} \, \tilde{\phi}(\omega) e^{i \omega t}$$
and plugging into the equation of motion, we find
$$\left( - \omega^2 + i 2 \beta \omega + \omega_0^2 \right) \tilde{\phi}(\omega) = \tilde{J}(\omega)$$
which can be rewritten as
$$\tilde{\phi}(\omega) = - \frac{\tilde{J}(\omega)}{\left( \omega^2 - i 2 \beta \omega - \omega_0^2 \right)} \, .$$
Let's take the case where the drive is a cosine, i.e. $J(t) = A \cos(\Omega t)$.
In this case $\tilde{J}(\omega) = (1/2)\left(\delta(\omega - \Omega) + \delta(\omega + \Omega) \right)$ so if you work it all out you find
$$\phi(t) = \Re \left[ - \frac{A e^{i \Omega t}}{\Omega^2 - i 2 \beta \Omega - \omega_0^2} \right] \, .$$
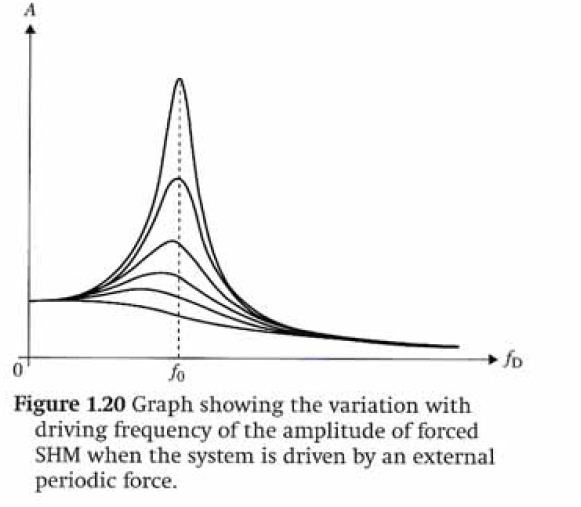
It's easy to check that $\phi(t)$ has the largest amplitude when $\Omega = \omega_0 \sqrt{1 - 2 (\beta / \omega_0)^2}$, which decreases as $\beta$ increases.
Remember that $\beta$ is just proportional to the coefficient of friction $\mu$ so we've shown that more friction makes the peak move to lower frequency.
Resonance
We've shown that the amplitude of the oscillator depends on the damping coefficient.
However, this does not mean that the resonance moves to a lower frequency.
Resonance is a condition defined by the unidirectional flow of energy from the drive to the system.
It turns out (easy to show with the math we already did) that this happens when $\Omega = \omega_0$, i.e. the drive is at the same frequency as the undamped oscillation frequency.
There's already a nice post on this issue which I recommend reading.
Original questions
Why does this happen?
Well, we showed why mathematically.
Intuitively it's because the friction takes away kinetic energy so the oscillator doesn't make it as far from equilibrium on each cycle.
And does this imply that at higher damping levels one cannot achieve a higher amplitude by setting the period of the forced oscillation to be equal to the natural frequency?
Assuming a constant amplitude of the drive, yes.
This seems strange because the highest amplitude is achieved when the natural frequency is equal to the driving frequency. But, I guess that the rules are somehow different for the damping case.
Indeed, damping changes things a bit.
Other reading