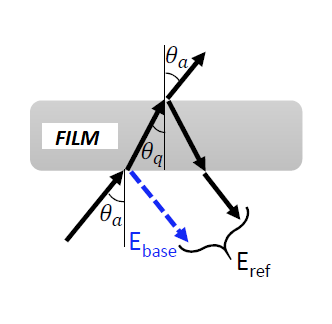
I have analysed a thin-film sample on a reflective setup, using a setup similar to the one represented in the figure below. I also know the thickness of the sample d to be considerably smaller than the wavelength of the signal used.
How can I obtain the refractive index of such a sample?
- I thought of calculating the sample-to-reference ratio in terms of the Fresnel coefficient of the air-sample, and sample-air interfaces, but because we have air on both sides, this would result in simply 1, and thus not work.