0
$\begingroup$

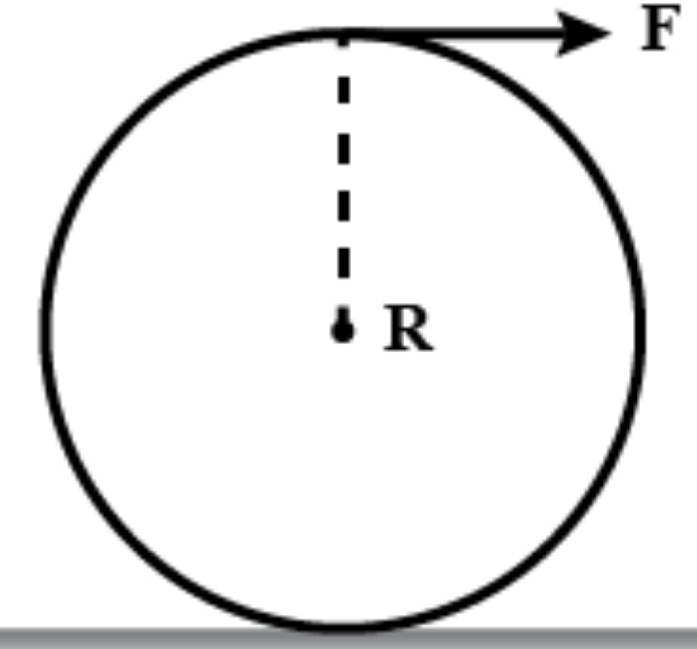
Consider a body like a ball on a frictionless horizontal surface. I apply some force F tangent on it, at the top. Will it translate? I was confused if it can translate or not because I imagined there's only a tangential force, which would provide torque. But now it doesn't follow F=ma as it isn't translating. So it must translate not just rotate. But why? enter image description here

Shouldn't the bottom of the ball keep on slipping backwards making it unable to move forward?

Improve this question
$\endgroup$
1

6 Answers 6

Reset to default
1
$\begingroup$

It is always true for a system of particles (rigid body or not) that $F_{net \enspace external} = Ma_{CM}$ where $F_{net \enspace external}$ is the net external force, $M$ is the total mass, and $a_{CM}$ is the acceleration of the center of mass. For your case, the force moves the center of mass.

Improve this answer
$\endgroup$
1
$\begingroup$

So it must translate not just rotate. But why?

It must translate because the only external force acting on the ball is $F$ in the horizontal direction, or $F_x$. Thus the COM of the ball will have translational motion with acceleration of $a_{x}=F_{x}/M$.

Hope this helpsl

Improve this answer
$\endgroup$
0
1
$\begingroup$

If the force were directly in line with the COM it would cause translation without rotation. When the force is not directly in line with the center of mass it will cause rotation and translation. If you had the force applied on top of the ball, as in your diagram, and you also apply an equal and opposite force on the bottom diametrically opposed to the top force, you will have rotation without translation. This is because the top force to the right and the bottom force to the left are both acting equally to rotate the ball, but the opposing forces cancel out translation of the COM.

Improve this answer
$\endgroup$
4
  • $\begingroup$ But consider this. If I apply a tangential force, the bottom part will have to keep slipping back, which would make the ball unable to go ahead. $\endgroup$
    – Adi
    Commented Dec 20, 2020 at 5:07
  • $\begingroup$ @Adi So in the presence of an net external force the center of mass will remain stationary is what you imply. This will not happen. Imagine you had a yoyo in space if you pull the string when it's winded it will always come to you while rotating unless someone has been pulling it in the opposite direction with the same force. $\endgroup$
    – Linkin
    Commented Dec 20, 2020 at 5:58
  • $\begingroup$ Got it Thanks a lot : $\endgroup$
    – Adi
    Commented Dec 20, 2020 at 8:32
  • $\begingroup$ @Adi Though the bottom part has no friction in this case, it still has inertial resistance to the angular acceleration (per Newton's first law) which will cause some of the force to go to translation of the COM. $\endgroup$
    – Adrian Howard
    Commented Dec 20, 2020 at 16:01
1
$\begingroup$

A way of answering your question is to add two extra forces which are equal in magnitude and opposite in direction (ie net force zero) at the centre of mass as shown in the diagram below.

enter image description here

You now have a force (black $F$) acting at the centre of mass which produces a translational acceleration of the centre of mass of the body and a couple (red $FR$) which produces an angular acceleration of the body.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Such a nice trick. Very helpful! $\endgroup$
    – Kashmiri
    Commented Jun 9, 2023 at 13:48
1
$\begingroup$

I guess it will then both translate and rotate:

$$F = m \ddot{x}$$

in the horizontal direction and

$$Fr = I \ddot{\phi}$$

about the CM.

Or, by statics, the equivalent system of forces about the CM is $F$ pointing toward it and a torque with value $Fr$.

Improve this answer
$\endgroup$
0
$\begingroup$

There is a conservation of energy in play here. The more spin the force generates, the less it can transform to forward motion and vice versa.

In essence, as the r increases, the more of the force is transformed into rotational momentum. With shorter r values, the more it will translate to forward acceleration.

I guess this is the same what Petrini was referring to.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.