Orbital angular momentum of a electron

Question

I am a high school student. My query is that I have read somewhere that orbital angular momentum is related to the motion of the electron or any subatomic particle (I know that this is not the same as in classical mechanics). I want to ask the following: In $s$ subshell i.e for azimuthal quantum no. $l=0$, the orbital angular momentum of an electron is $0$, does it means that it is not moving at all? If it is true then why doesn't it falls towards the nucleus? Some people say that $l=0$ only means that it has no angular dependency. Is it true? Please explain it intuitively not mathematically.

Answer 1

Mapping classical and quantum-mechanical properties one onto the other can be very tricky. Usually, the mapping relies on resemblance that is valid at classical scales (large systems) but some of our intuition may break down when going down to the atomic level.

The classical angular momentum indeed describes motion, that is change in position with respect to time. And specifically circular motion. In quantum mechanics the notion of motion is a bit murkier, as you probably know the position of a particle may be not well defined, with different probabilities to find it in different positions. So change in that position may be murkier still. In fact, the entire idea of dynamics is slightly changed, and when analyzing the orbitals of an atom we are actually describing stationary solutions. That is - states that do not change over time (for the toy-system that consists only the nucleus and the surrounding electron).

Then the entire idea of "electron moving around the atom" needs to be abandoned if we want to describe the quantum-mechanical notion of it. However, there is still a mapping of classical angular momentum onto a quantum one, and it retains the idea of angular dependence. So the quantum angular momentum describes how the probability distribution to find the electron at certain positions (that is what replaces in quantum mechanics the classical position of the electron), is "skewed angularly" about some center. In atomic context, the $s$ orbitals have zero angular momentum, which means that they don't have any angular dependency, and the probability to find the electron when it is in this shell is uniform in any direction. The states with non-zero angular momentum will have a probability distribution that prefers some directions to others.

One feature that can be taken directly from classical angular momentum to angular one, is that only electrons with no angular momentum (that is $l=0$, or $s$ orbitals), have any probability to be found right in the center. For any orbital with $l>0$ the electron has zero probability to be found at the center of the atom, which indeed sits well with out classical intuition of something that revolves around the center.

Answer 2

$J=0$ means that all motion is radial. For a wave function, in this case, $\frac{\hbar}{i}\vec \nabla \psi$ is radially oriented everywhere.

Answer 3

The fact that electrons don't fall into the nucleus has puzzled scientists for many years and is one of the reasons that quantum mechanics was developed. In quantum mechanics particles are described using wave functions. To understand what wave functions are ask yourself the question "when I drop something in the water and it makes a wave, at what location is that wave?". You can argue that the wave has no location but a valid answer is a little bit here and a little bit there. The same is true for electrons. They are spread out in space. The way this electron wave moves is described by the Schrödinger equation. It is hard to understand but what we can take away from it is that the wave equation is constrained. Not every wave function solves this equation.

Physically this means that physical quantities like energy and angular momentum can only take on discrete values. Below are some of these solutions for the hydrogen atom. 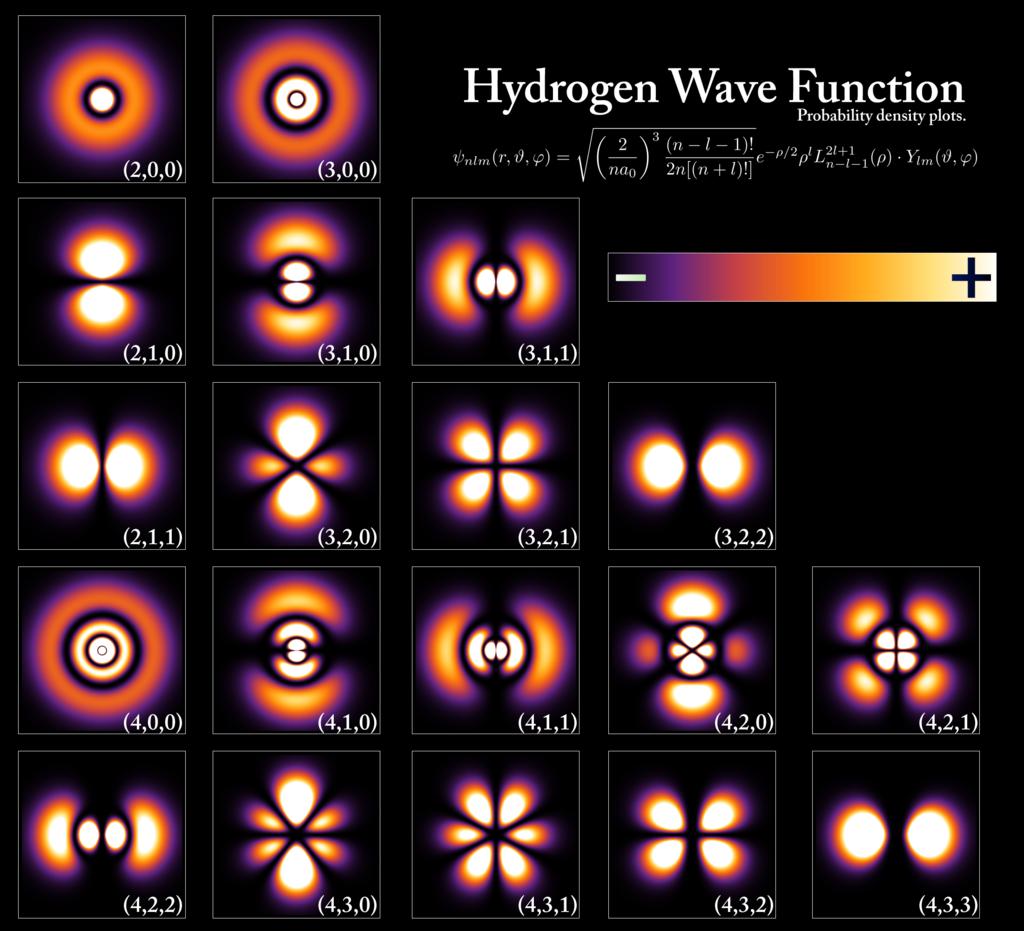The first value ($n$) corresponds to energy and the second value ($l$) corresponds to angular momentum. We see that when $l=0$ the function is spherically symmetric (no angle dependence). But this state doesn't correspond to the electron falling towards the nucleus. It just happily exists as a spherical cloud. The electrons don't fall towards because quantum mechanics doesn't allow such a state.

A final note: the $l$ does correspond to angular momentum. If you were to see the states with $l>0$ evolve in time they would rotate around the atom. Also you can add these solutions together to make more complicated solutions, but over time the electrons take on the lowest energy state. That's why these solutions in the picture are important.