2
$\begingroup$

So, I was am having a hard time understanding the location of an orbital inside an atom.

I read that:

Within each subshell, there are orbitals. This gives the final location of where electrons reside

In this video it looks something like this:

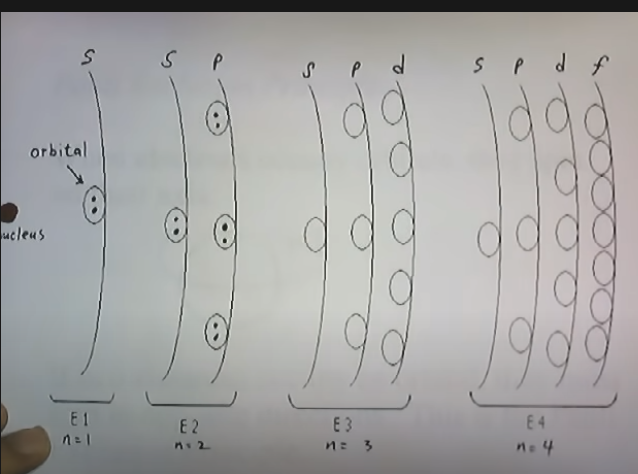
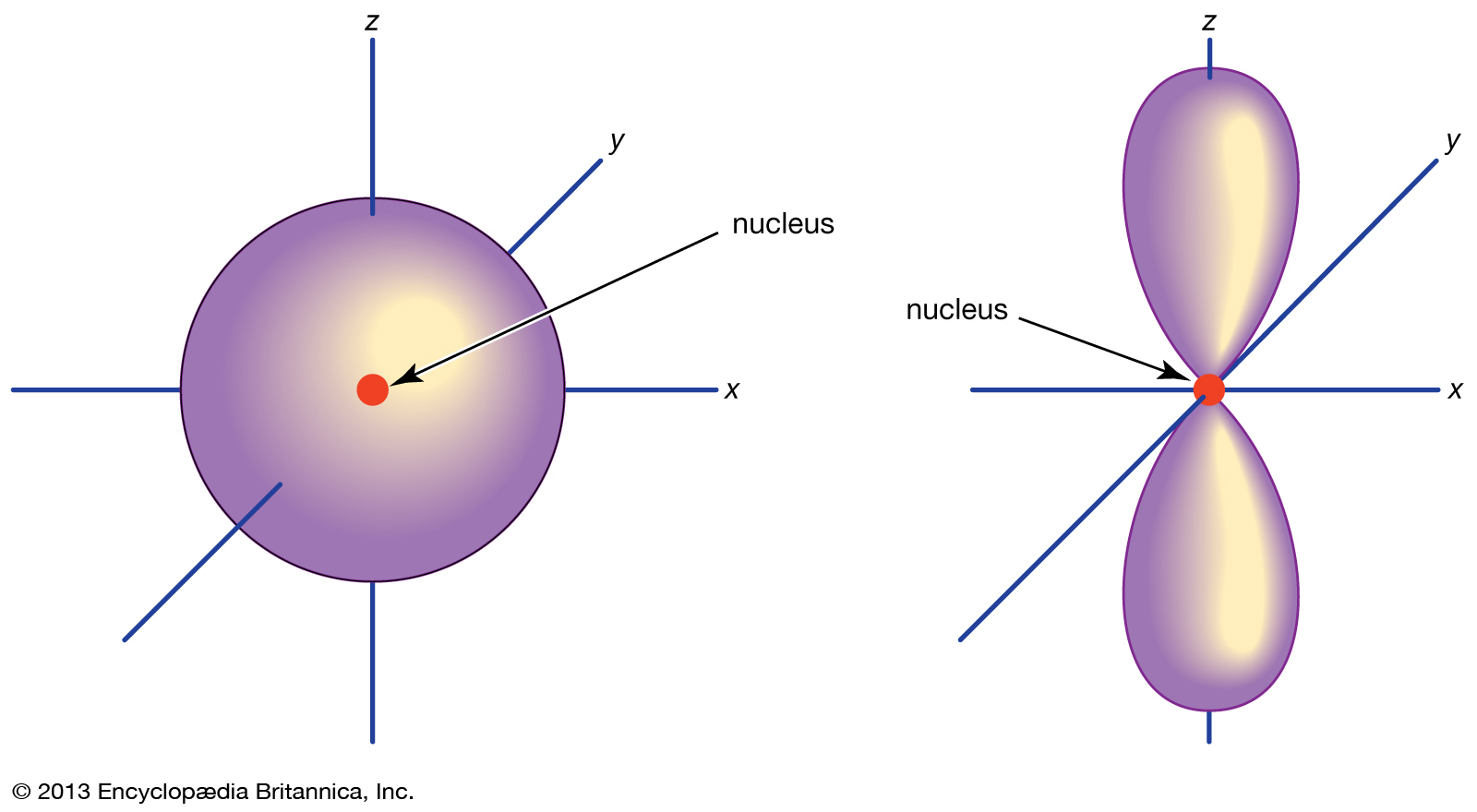
enter image description here

But from other diagrams I have seen it looks like a large 3 dimensional space around the nucleus where there is a probability of finding the electron. I.e:

enter image description here

So are the orbitals really around the nucleus or are they subparts of the subshells? And how are they related to the principal quantam no. and the angular momentum quantum no.

Improve this question
$\endgroup$
1
  • $\begingroup$ Looks like the drawings in the video are meant to be some sort of a schematic representation of the different energy levels of an atom. I don't think it is intended to tell you anything about the geometry. Roughly speaking; energy levels are comprised of groups of orbitals that all have similar binding energy, and "shell" and "subshell" are more or less synonyms for the same idea. All of those words come from a time before quantum mechanics explained any of it. $\endgroup$
    – Solomon Slow
    Commented Aug 5, 2021 at 14:41

1 Answer 1

Reset to default
1
$\begingroup$

But from other diagrams I have seen it looks like a large 3 dimensional space around the nucleus where there is a probability of finding the electron

That's exactly what orbitals are. The shape they have is actually a probability distribution that shows the most likely location of the electrons.

So are the orbitals really around the nucleus or are they subparts of the subshells?

The orbitals are distributed "around" the nucleus, but their shape is to be considered strictly a probability distribution and not some distinct “shape” that houses the electrons. Orbitals that have the same value for $l$ form a subshell (see below).

And how are they related to the principal quantam no. and the angular momentum quantum no.

The principle quantum numbers have an integer value $n$, and generally speaking, the energy of an electron in an atom increases for increasing values of $n$.

The angular momentum quantum number denoted by $l$, is also an integer that defines the shape, as you have above, $s,p,d,\cdots$

This number is allowed to take on the values $l = 0, 1, 2,\cdots, (n – 1)$. Also, the higher the value of the angular momentum quantum number, the higher is the value of the angular momentum of the electron.

Improve this answer
$\endgroup$
1
  • $\begingroup$ No worries. Good luck with your studies. Cheers. $\endgroup$
    – joseph h
    Commented Aug 5, 2021 at 8:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.