Intuitive explanation for why blue light is refracted more than red light? [duplicate]

Question

I understand the equation explaining angles of refraction related to wavelengths. But I'm looking for a more intuitive approach/visual explanation? Perhaps related to QM?

Answer 1

Snell's law tells us that the angle of refraction depends on the index of refraction, $n_1 \sin{\alpha_1} = n_2 \sin{\alpha_2}$. However, the question remains, why $n_{\text{blue}} > n_{\text{red}}$. In order to address this, we need a model for the refractive index.

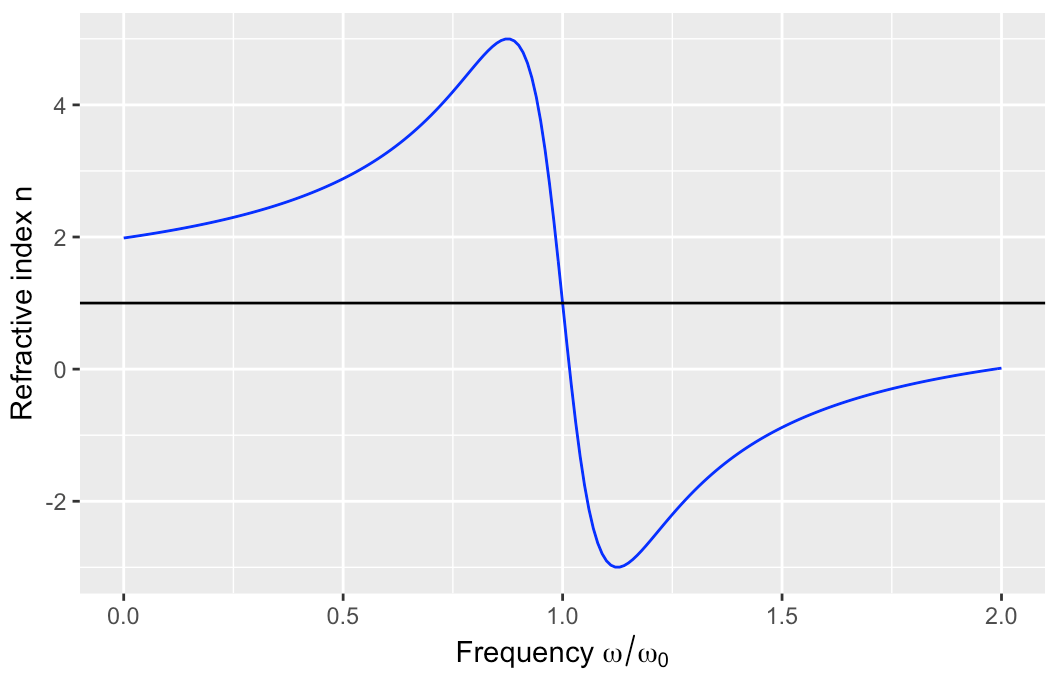
The refractive index $n$ of a material is related to the atomic transitions of the material. To a good approximation the atomic transition can be modelled as an harmonic oscillator, with eigenfrequency $\omega_0$. This leads to $$ n = 1 + \frac{\text{pre factor}}{\omega_0} \cdot\frac{\omega_0- \omega}{(\omega_0- \omega)^2 + (\gamma/2)^2} $$ where the pre factor is not dimensionless. Plotting the refractive index over the frequency yields

If we consider glass, the atomic transitions are at higher frequencies compared to the frequency in the visible range. Thus, the refractive index of visible light corresponds to the left side of the plot. Here we see, that the refractive index increases with increasing frequency. Hence, the plot "explains" why $n_{\text{blue}} > n_{\text{red}}$.

Answer 2

To explain this, we need to use the Fermat's principle of least time. According to the principle of least time, a light ray always takes the path which is the quickest. We also need to know another fact that the speed of all different colours of light in vacumm is the same, but in any other medium like glass, water, etc. their speed is different. And it has been observed that in all those other media, violet light is the slowest and red light is the fastest. The speed gradually decreases from red light to violet light.

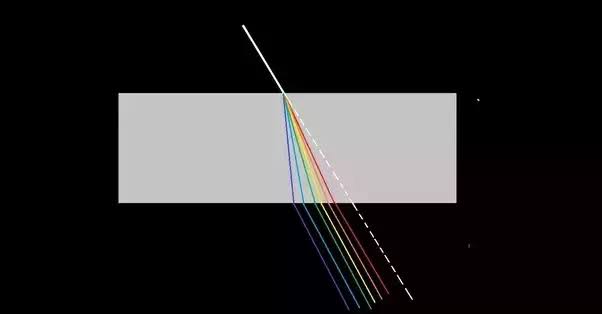
Now let's get to your main question. I would like you to see the diagram below.

^{Image source}

Now imagine that you are one of the components/colours of the white light and your aim is to reach the other end of the glass slab and come out from the glass slab into the air. So now, when you are in air (which is practically equivalent to vacuum when talking about optical phenomena), you and the other colours have the same speed. But as you cross the air-glass interface, your speed reduces. The speed of all other colours also reduce, however the final speed of all the colours is different as the change in speed is different. And then you again come back out of the glass which and now all the colours again have the same speed.

Now if you are a violet coloured ray, then you will be the slowest among all the other colours and thus you would try to take a shorter path through the glass slab (for which you'd have to bend more) because you don't want to waste time by going slow over a longer distance. Why so? Because you are obliged to follow the Fermat's principle of least time. However, if you had been a ray of red light, you'd be comparatively faster in the glass slab and thus you wouldn't have to worry much about taking a shorter path and thus you would bend less.

The reason why all the rays just won't take the straight path, perpendicular to the edge, from one edge to another is because it is not only the time in glass slab to be minimized but also the time taken after refracting out of the glass slab, into the air. Thus we have to make a compromise between a perpendicular path and the original path. The slower a ray's speed, the more closer its path will be to the perpendicular path.

Note: In this answer, I have just walked you through the intuition behind any normal refraction. The bending of colours trivially follows from the fact that different colours have different speeds. So this answer gives more of an intuition of Fermat's principle of least time, which is then simply used to make conclusions about dispersion of light into its constituent colours.

Answer 3

Unfortunately, there's no easy explanation for this. It involves some basic atomic physics, so you will need a picture of how electromagnetic fields interact with matter. Consequently, some of this may be difficult to understand, but hopefully you can ask about (or read up) whatever you don't understand.

Semoi's answer is correct, but a lot of details have been left out. I will try to fill in some of the gaps. Semoi's description is the modern semi-classical understanding of the process, but we can step back to the older Lorentz-Lorenz model to understand the essential idea. I'll explain all this in what follows.

What is happening when light refracts is that when the electromagnetic wave (the light ray) hits a material, it induces the charges in the material to move. Basically, the electron cloud of the atoms are pulled one way (because it is negatively charged) and the nucleus is pushed in the opposite direction (because it is positively charged). This creates a dipole field that opposes the incident field of the electromagnetic wave, and reduces the perpendicular component of the electromagnetic field, and this changes the direction of the wave.

Fermilab have a good video explaining this, which is not too detailed. They don't talk about the atoms, though -- they just say the charges are randomly placed in the material. But these are the details they have skipped over.

Most undergraduate electromagnetic courses will also cover this in detail. David Tong's lecture notes are quite good. You want chapter 7, Electromagnetism in Matter. You can also search online for any other set of notes that you like better: if they are posted, they will usually be open for anyone to read.

Now, this explains why light refracts, but why does blue light refract more than red? The light ray is composed of oscillating electric and magnetic fields. So the charges in the atoms are not just moved in one direction and that's it, they are being oscillated because the field itself is oscillating. As such, the induced dipole field acts like a (damped) harmonic oscillator that is being driven by the external field (the light ray). And like all damped harmonic oscillators, the response of the oscillator depends on what the driving frequency is with respect to the natural frequency of the oscillator. Going back to Semoi's answer, for glass, for example, the natural frequency of the atomic dipole is much higher than the frequency of visible light, so the closer the driving frequency (the frequency of the light ray) gets to it, the more in phase the incident field (the light ray) will be with the induced dipole field. And the more in phase these two fields are, the stronger they will cancel. And the stronger this cancelling is, the more the ray will be refracted, as per the explanation above. If this talk about damped oscillators is a bit confusing, then look online for a good description of forced/driven harmonic oscillators, or forced/driven simple harmonic motion. A cursory search shows what looks like a few university videos: again, pick whichever seems easiest to you to understand.

Where Semoi talks about transitions, this is the modern semiclassical understanding of the process, whereby rather than shifting the position of the electron cloud, the electrons are instead excited into higher orbits. The higher-energy orbits are located further away from the nucleus, so effectively this is like moving the electron cloud. The only difference here is a more accurate description of the atomic dynamics -- the end result is basically the same.

This is a page from The Physics of Laser-Atom Interactions, by Suter. It has a good description of the basic physics, but, again, it may be too advanced. It will cover all the concepts though. If you can get your hands on a copy, then I recommend giving it a read.

Answer 4

So your question is what is the microscopic reason for material dispersion in optics, meaning $n=n(\lambda)$ ("dispersion relation"), where $n$ is the refractive index of the medium and $\lambda$ the wavelength. Because by snells law $\frac{\sin \theta_{2}}{\sin \theta _{1}}=\frac{n_{1}}{n_{2}}$ (see FakeMod's answer) this means a different refraction for different wavelengths. You're specifically asking about the case of normal dispersion, where a decrease in index of refraction leads to an increasing wavelength, which is the case for most transparent media.

On a microscopic level an incoming electromagnetic wave displaces particles of different charge and thus are creating dipole moments (polarization). The strength of this effect is characterized by the electric susceptibility of the material, which again depends on the wavelength.

Maybe think of it in this way. The particles are inert, they don't want to be oscillated. So they take a bit of time to move, after the EM-wave passed. If you're now applying light of different wavelengths and thus different frequencies, they will move faster or slower, depending on how fast the EM-wave oscillates.

Edit1: Misspelling

Edit2: It is important to notice that the velocity of a light-source can't affect the speed of light in vacuum, because of special relativity. The slowing of the speed in the material is due to the Ewald-Oseen extinction.

Answer 5

If an analogy helps:

Picture walking towards a plowed field, which you meet at an angle to the edge of the field. The furrows run parallel to the edge of the field at which they meet.

The furrows make it harder to walk over the furrows at an angle, and you compensate by turning into the field. However, longer legs mitigate this effect so that you don't need to turn as much.

In this analogy, you are a photon, the plowed field is a substance with a higher index of refraction, and the length of your legs is the wavelength of the photon.