In any area of mathematics or physics, we can introduce diagrams as a way of exactly representing certain facts or properties, if we carefully define how the diagram is to be interpreted. In this respect such precisely-defined diagrams are like algebraic symbols. Indeed, some areas of maths are handled by developing a notation where the symbols are like little diagrams.
In the case of spacetime we are dealing with manifolds. The most natural way to allow a diagram to give information about a manifold depends on the type of manifold. Here are examples.
Euclidean manifold in 2D. This is the easiest: an ordinary flat diagram on a paper or screen maps naturally to such a manifold. No special interpretation is needed; the interpretation is obvious.
Minkowskian manifold in 2D. This is the kind of manifold you encounter in Special Relativity. It is flat (the Riemann curvature is everywhere zero) but it is not quite the same as a flat piece of paper, because the latter has Euclidean geometry. In the Minkowski space the invariant is $-\Delta t^2 + \Delta x^2$ but in the Euclidean space the invariant is $+\Delta z^2 + \Delta x^2$. This means that when the Euclidean diagram is used to visualize and Minkowski spacetime, some interpretation is needed. For example, the length of a line in some arbitrary direction on the diagram is not related in any simple way to the length of the corresponding spacetime interval (i.e. $(\Delta z^2 + \Delta x^2)^{1/2}$ does not behave like $(-\Delta t^2 + \Delta x^2)^{1/2}$). Lines that are orthogonal in the spacetime sense are mostly not at right angles to one another on the diagram. However the situation is not too bad: lines forming a pair whose angle is bisected by a photon worldline are spacetime-orthogonal, and distances parallel to any give time- or distance-axis are proportional to time intervals and spatial intervals in the corresponding reference frame, with the proportionality constant indicated by a hyperbola of constant interval from the origin.
Curved 2D space with positive signature. This is the kind of manifold which can represent space (not spacetime) around a gravitating object such as a star or a black hole, especially when there is spherical symmetry so we can use a 2D slice to tell us about the whole space. In this case it is often possible to use an
embedding diagram. Here we go up one dimension, to 3D, such that the 3D space is Euclidean. Then we draw a surface in this 3D space. The surface is 2D, but it need not be flat. For many cases it is possible to construct this surface in such a way that distances along the surface embedded in Euclidean 3D space (so the distances we naturally see when we look at the diagram) exactly match the distances in the curved 2D space. This kind of diagram is widely used to help us get intuition about the Schwarzschild-Droste spacetime. We treat the region outside the horizon, and take a slice through spacetime at some given time. We then have a 3D space which with spherical symmetry. We take a slice through that space, thus getting a 2D manifold with positive signature. The embedding diagram looks like this
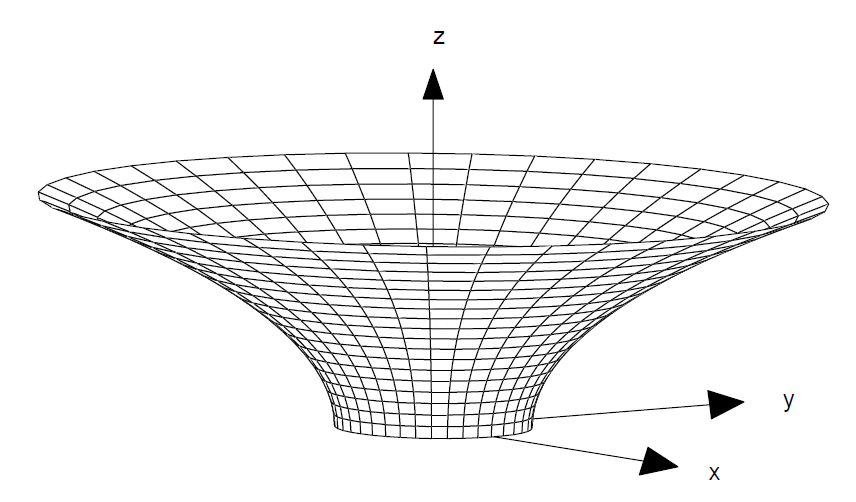
This is a precise diagram; it is called Flamm's paraboloid. Here are a few things you can deduce. (i) Each small region has the shape of a saddle, therefore the Gaussian curvature is negative. (ii) If you obtain the Gaussian curvature at each point, the value matches that of the Schwarzschild-Droste space. (iii) The distance from the horizon to any point is finite. (iv) As you walk outwards, increasing your distance from the horizon by $\Delta r$, the circumference of the circle about $z$ grows by less than $2\pi\Delta r$, and furthermore the diagram indicates exactly by how much it grows.
- Curved spacetime. The case of a curved space with a Minkowski signature is more difficult. There is, in principle, a Euclidean space of higher dimension in which the manifold can be embedded, but the number of dimensions will be larger than 3 so such a diagram is itself hard for us to visualize.
Finally, another remark on case (3), the curved 2D manifold with positive signature. Another example occurs in cosmology. Space on a very large scale may be flat, or it may have constant non-zero curvature. If the curvature is positive then you get a 3-sphere, and a cross-section through it gives a 2-sphere. That is a 2D manifold whose curvature and topology exactly matches that of the surface of an ordinary sphere in Euclidean space. So the widely used 'balloon model' of the cosmos, where an expanding balloon represents the expanding space of the cosmos, is a correct and exact model if it is interpreted correctly. Note especially that it is the surface of the sphere that represents a slice through the space of the cosmos.

