1
$\begingroup$

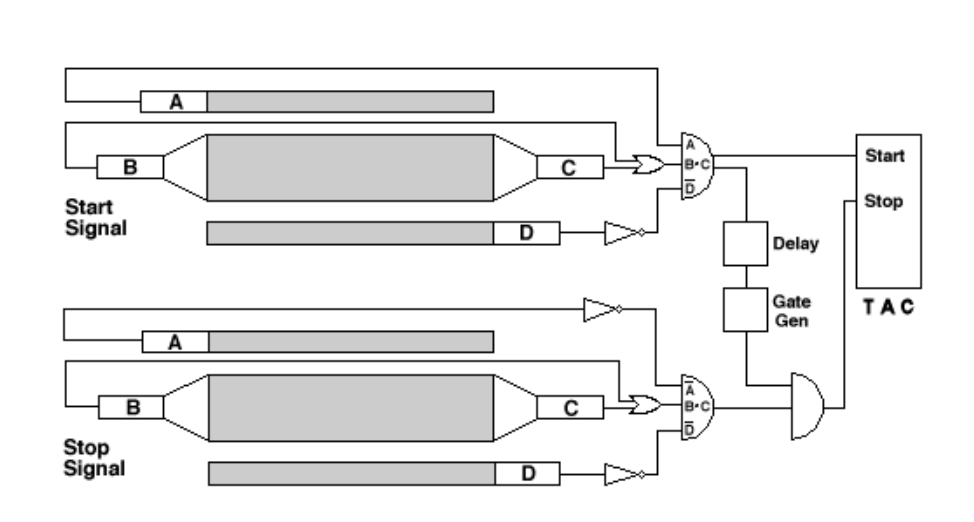
I am trying to measure muon's mean lifetime using 3 scintillators. The logic of the experiment is shown below: enter image description here

I know that accidental coincidences could happen because of unrelated muons that could go through the middle scintillator (skipping the top and bottom one) which triggers a stop that might interfere with another muon that has already triggered a start. Is there a way to estimate the accidental coincidences of this setup?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Accidentals can be due to independent muons, but they can also be due to random firing of the phototubes.

You can’t calculate the accidental rate without a measurement of the individual tubes’ singles rates and knowing the length of the gate.

Since you have to measure those anyway, the easiest way to get the accidental rate it to move the tubes into a non-coincident alignment and measure the rate.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Could you explain what you mean by singles rate? $\endgroup$
    – NegativeTension
    Commented Mar 30, 2019 at 3:57
  • $\begingroup$ If a tube is just sitting there, it randomly fires at some rate that’s typically much larger than the rate of real muons. This is called the singles rate. $\endgroup$
    – Bob Jacobsen
    Commented Mar 30, 2019 at 4:04
  • $\begingroup$ I have set up the discriminator thresholds to minimize this, so would it make sense to just assume the accidental counts as due mostly to muons? and then the accidental counts would be $r^2$t where t is the gate generator window and r is the rate of incident muons. $\endgroup$
    – NegativeTension
    Commented Mar 30, 2019 at 4:18
  • $\begingroup$ Source of random pulses doesn’t matter, just their rate. If you know that’s $f$ and the gate time $T$, you can calculate the Poisson probability of 0, 1 or more in that window. $\endgroup$
    – Bob Jacobsen
    Commented Mar 30, 2019 at 4:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.