The electric field of an oscillating point charge consists of a Coulombic component and a radiative component:
- the Coulombic part is generally directed away from the charge, and it goes down as $1/r^2$ with the distance from the center of the oscillations.
- the radiative part is generally transversely polarized, and it goes down as $1/r$ with the distance from the charge.
When we're considering radiation, we keep the $1/r$ component, because it dominates completely over the $1/r^2$ Coulombic near-field when you're far away from the charge. This explains the discrepancy you observe.
As to how the direction of the electric field is determined - that obviously depends on the situation. Plane waves are a model, and nothing more, and they are generally a terrible model for the field radiated by a point charge. (Instead, you normally use spherical EM waves.) Different situations call for different models, and different characteristics of the radiation within those models.
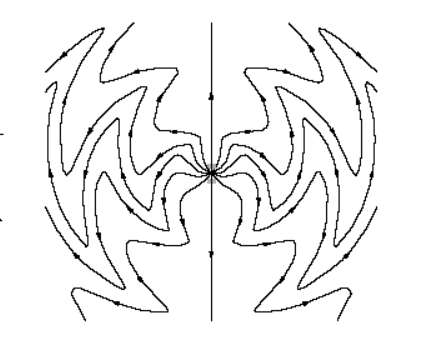 http://physics.weber.edu/schroeder/mrr/MRRtalk.html
http://physics.weber.edu/schroeder/mrr/MRRtalk.html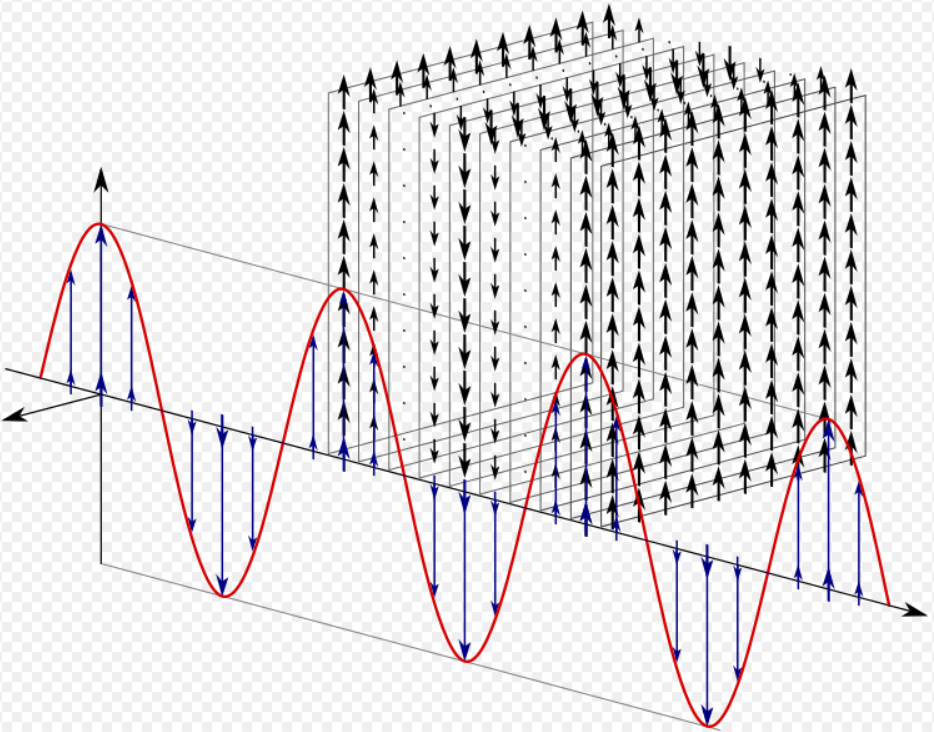 https://en.wikipedia.org/wiki/Plane_wave
https://en.wikipedia.org/wiki/Plane_wave