3 repelling parallel line charges

Question

Addendum 1 (Illustration added at the beginning):

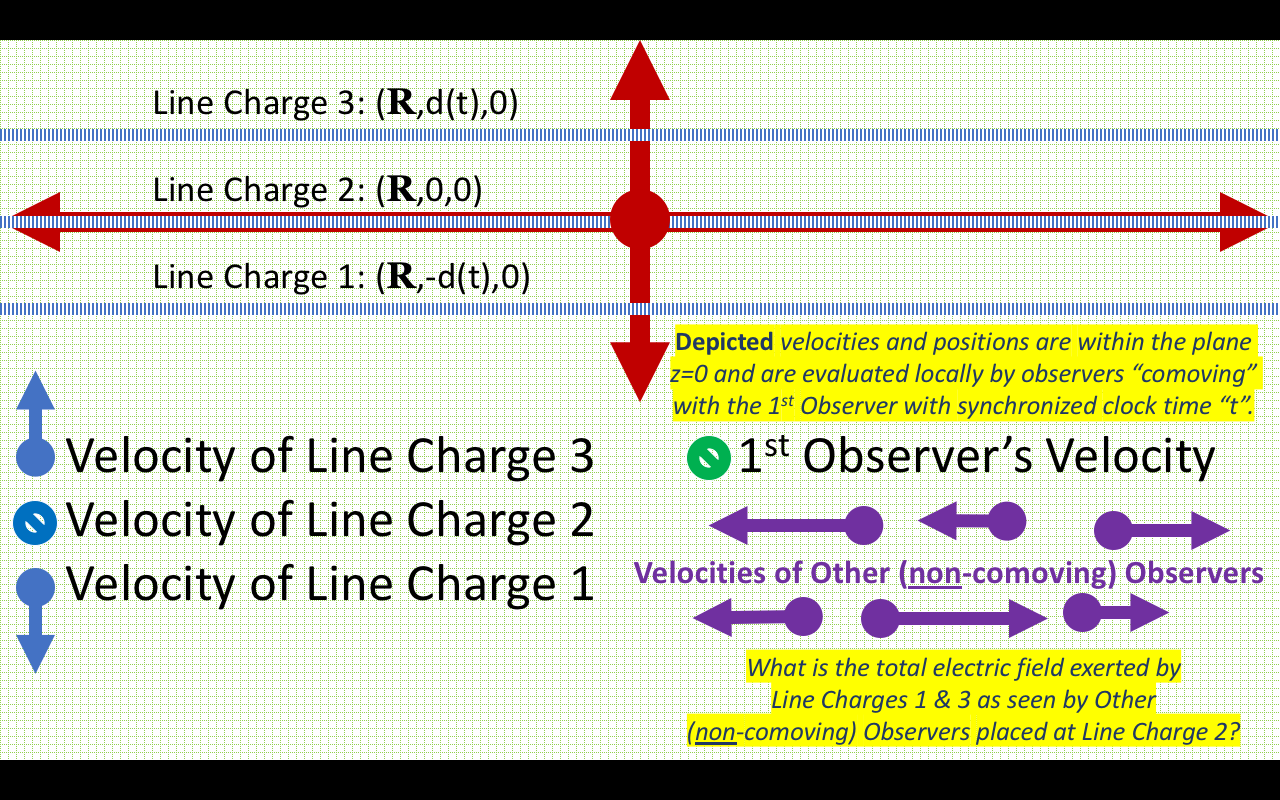
Suppose I had 3 identical parallel line charges parallel to the x-axis of infinite length in the same geometric plane.

The first and third line charges are equidistant from the second.

Now suppose I have a series of observers that have different velocities which vary only along the x-axis (i.e. differences in the x-component of velocity only).

For our first observer, the "center of charge" is stationary, and for the rest of the observers the "center of charge" is moving at some velocity directed along the x-axis. The first observer would observe no component of the electric field directed along the x-axis.

However, the others would observe such electric fields directed along the x-axis.

How do we ensure there is no acceleration of the second line charge despite the electric field exerted on it in the x-direction? Is its momentum affected due to the varying "electric potential" therefore "mass" in some compensating way, ensuring no acceleration, as in (mv)' - m'v = mv' = 0?

Addendum 2 (Added complementary information):

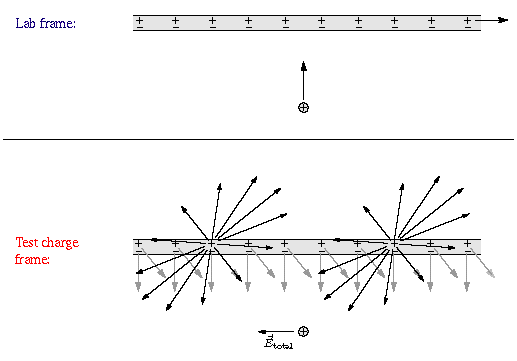
One should consider how a neutral wire with an electric current can be represented by the superposition of two line charges with equal and opposite linear charge densities while having different velocities. Notice that in the bottom half of the illustration below labeled "Test charge frame:" (in red) it shows an electric field with a component parallel to the wire that exists because the positive charges contribute to it and yet the negative charges do not negate that contribution. From http://physics.weber.edu/schroeder/mrr/mrrtalk.html

I've included information about this because some may doubt that electric field components in the x-direction are seen by observers having different component velocities in the x-direction than those of the line charges.

Answer 1

Firstly we need to understand that electric field has inertia. See this: https://www.physicsforums.com/threads/energy-density-in-an-e-field-does-it-contribute-to-inertial-mass.1015388/

Secondly we need to understand that electric field around moving line of charges has momentum pointing to the direction of the motion.

Thirdly, if two identical parallel lines of charges are moving away from each other, then the electric field around the lines loses inertia, and also momentum if it has any, because inertia is a factor of momentum. The charges absorb the lost inertia and the lost momentum. This is the reason that the charges experience a force along the x-axis.

The reason that the charges don't accelerate along the x-axis is that they don't gain so much momentum that they would accelerate, they only gain so much momentum that they can continue at the same speed and increased inertia, inertia of kinetic energy being the extra inertia.

If a line of charges happens to collide with some inelastic barrier so that its y-speed decreases, then we will notice a x-acceleration, as the extra inertia disappears. Anybody doubting this can do the experiment, but the change of x-speed is equal to the change of time-dilation factor, so it may be difficult. : )

(The reason that the ratio of x-speeds before and after the y-speed change is the same as the ratio of time-dilation factors before and after the y-speed change should be obvious)

Answer 2

As I said in my comment, you need special relativity to transform the components of the $\mathbf E$ and $\mathbf B$ fields. They transform according to $$ \begin{align} \mathbf E'_{||} &= \mathbf E_{||} \\ \mathbf B'_{||} &= \mathbf B_{||} \\ \mathbf E'_{\perp} &= \gamma (\mathbf E_\perp + \mathbf v \times \mathbf B_\perp) \\ \mathbf B'_{\perp} &= \gamma \left ( \mathbf B_\perp -\frac{1}{c^2} \mathbf v \times\mathbf E_\perp \right ) \end{align} $$ where the primed quantities denote the (total) fields at a frame moving with velocity $\mathbf v$ relative to your original frame ($\mathbf v$ is the velocity of any observer), $\gamma$ is the Lorentz factor and the subscript $||$ denotes the component parallel to $\mathbf v$ and $\perp$ the perpendicular component. As you can see, the parallel components remain the same, so no acceleration in the $x$-direction will be measured by any observer. The cool stuff happens in the second figure, where a magnetic field is created whose force exactly counterbalances the electric force in order to don't accelerate the charge.