Does this body tilt if we push it with F force?

Question

The question exactly is:

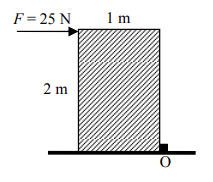
A $20 \text{ kg}$ uniform density wooden crate of height $2 \text{ m}$ and width $1 \text{ m}$ is pushed at one of its edges at its top by a $25 \text{ N}$ force as shown in the figure. The force is horizontal and perpendicular to the edge of the crate. Will the crate be tilted? (The obstacle at O hinders the slipping of the block.)

So here's my train of thought:

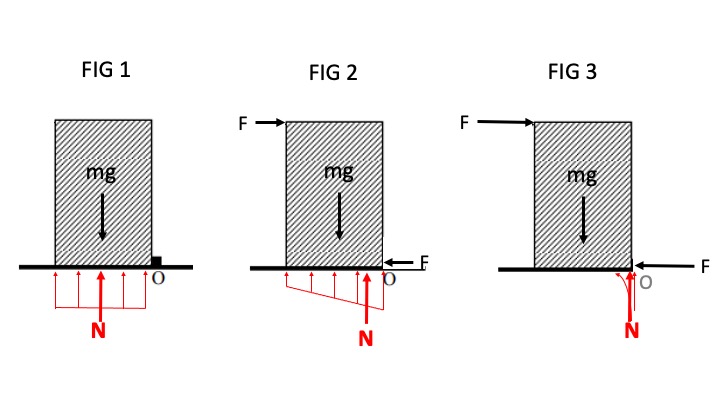
G (200N) pulls down the body, N (-G = -200N) is the constraint force. If this wasn't there the body would fall down which doesn't happen. F' (= -F = 25N) prevents the block from slipping.

If the body was at rest, the sum of forces should be 0 plus the sum of torques should also be 0. The sum of forces is 0, that is for sure.

The sum of torques is what I have problems with. I choose point O as the pivot point. But then the torque of F is the only torque that would act on the body (since G is counterclockwise and N is clockwise and their magnitude is equal). But then that would mean that no matter how little force I exert on the body, it would tilt no matter what which is obviously not the case.

Could you tell me what I get wrong?

Answer 1

Could you tell me what I get wrong?

You are not taking into account that the torque due to the normal force $N$ does not cancel the torque due to the gravitational force $mg$ when the force $F$ is applied.

Without an applied force $F$, the normal force $N$ is a uniformly distributed force on the bottom of the body which can be replaced by a single force acting through the center of gravity (COG) as shown in FIG 1 below.

But once you start applying the force $F$ the distributed normal force is redistributed, moving the location of the single equivalent normal force towards the right of the COG as shown in FIG 2. This produces a clockwise force-couple between the normal force $N$ and gravitational force $mg$ that counteracts the counter-clockwise force couple due to the the applied $F$ and reaction $F$ at $O$.

But the location of the normal force cannot shift to the right beyond $O$ (where there is no contact) as shown in FIG 3, which places an upper limit to the clockwise force couple that can counter the applied force-couple. In the limit, the normal force $N$ acts at $O$ together with the reaction force $F$ where they produce no torque about $O$. Then the only torques about $O$ are the weight, which is 100 Nm clockwise, and the counterclockwise torque due to the applied force $F$, which is 50 Nm. Since the clockwise torque is greater than the counterclockwise, the body will not tilt (it will remain stable)

Hope this helps.

Answer 2

Could you tell me what I get wrong?

You are forgetting what happens with the position where the vertical normal force acts as you push the block horizontally.

In the most extreme case, the very moment that the block "starts rotating" all the vertical normal force $\vec N$ is concentrated at the pivot point $O$ and produces no torque. In that case you have only the weight $\vec G$ working against the horizontal force $\vec F$. In other words, the vertical normal force is redistributed toward the pivot, and to start rotating the block the horizontal force needs to be

$$F \cdot 2 \geq G \cdot 0.5$$

For horizontal force less than that, the position where the vertical normal force acts is redistributed towards the pivot point such that

$$(F \cdot 2) - (G \cdot 0.5) + (N \cdot r) = 0$$

where $r$ is the distance from the pivot. Obviously, for $F = 0$ the distance is $r = 0.5$.