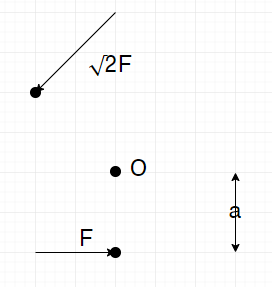0
$\begingroup$

Varignon's theorem states that

"If many coplanar forces are acting on a body, then the algebraic sum of torques of all the forces about a point in the plane of the forces is equal to the torque of their resultant about the same point."

Consider the following case:

enter image description here

Let's consider net moment about the point O.

Algebraic sum of torques gives us √2F*√2a + F*a = 3Fa

But resultant of the two forces = F in downward direction gives us the torque Fa

Clearly Varignon's theorem breaks down in this case. I've read elsewhere that Varignon's thorem doesn't apply for cases where a couple of forces is involved. And that's obvious. Because resultant of couple is 0 force which gives 0 torque - and that is wrong.

But in this case, the two forces don't seem to form a couple. The horizontal component of √2F force does form a couple with the bottom force, but that way, in a generalised problem with n forces, many components can form a couple. So how do we know when not to apply varignon's theorem?

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ The forces have to be concurrent (see e.g. Varignon's theorem (mechanics) ). They are not concurrent in your case, so the theorem does not apply. $\endgroup$
    – NickD
    Commented Jul 17, 2018 at 16:53
  • $\begingroup$ @NickD concurrency is a necessary condition. However, I have paraphrased an alternate statement of the theorem from the same wiki link, and this doesn't talk about concurrency. Can you please confirm wiki is wrong? $\endgroup$
    – yathish
    Commented Jul 18, 2018 at 9:18
  • $\begingroup$ I don't know which wiki you quoted (I don't see a link) but the "coplanar" part above should probably read "concurrent". Also your statement about the couple is wrong: the resultant force may be 0 but the torque is not. $\endgroup$
    – NickD
    Commented Jul 18, 2018 at 12:52
  • $\begingroup$ @NickD I'm quoting the wiki link you added in your first comment. You're misreading the 0 torque statement. I'll edit the phrasing so it's clearer $\endgroup$
    – yathish
    Commented Jul 18, 2018 at 14:25
  • 1
    $\begingroup$ I checked the reference: the "coplanar" part is a mistake (which I fixed). $\endgroup$
    – NickD
    Commented Jul 18, 2018 at 16:47

2 Answers 2

Reset to default
1
$\begingroup$

Varignon's theorem is applicable only for concurrent forces. The quoted part is incorrect and has been fixed (). For non-concurrent forces, the point of application of resultant force will change according to the moment.

In fact, the principle based on which we find the new resultant force's point of application is based on the fact that resultant moment should be the same as earlier. For couples, Moment has to be considered separately as their resultant could have no point of application which will yield the same moment (this is because resultant becomes 0 for a couple).

Improve this answer
$\endgroup$
-1
$\begingroup$

"But resultant of the two forces = F in downward direction gives us the torque Fa" That is wrong!!!!

You must find the point where you can apply the sum of the force : here it is (-3a,-a) from the point O! So you find the same result 3aF for the moment at the point O :-)

You have misunderstood the application force explanation, you can't sum the force than choose a point of application wich is one of the 2 forces, it's impossible. Regards

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.