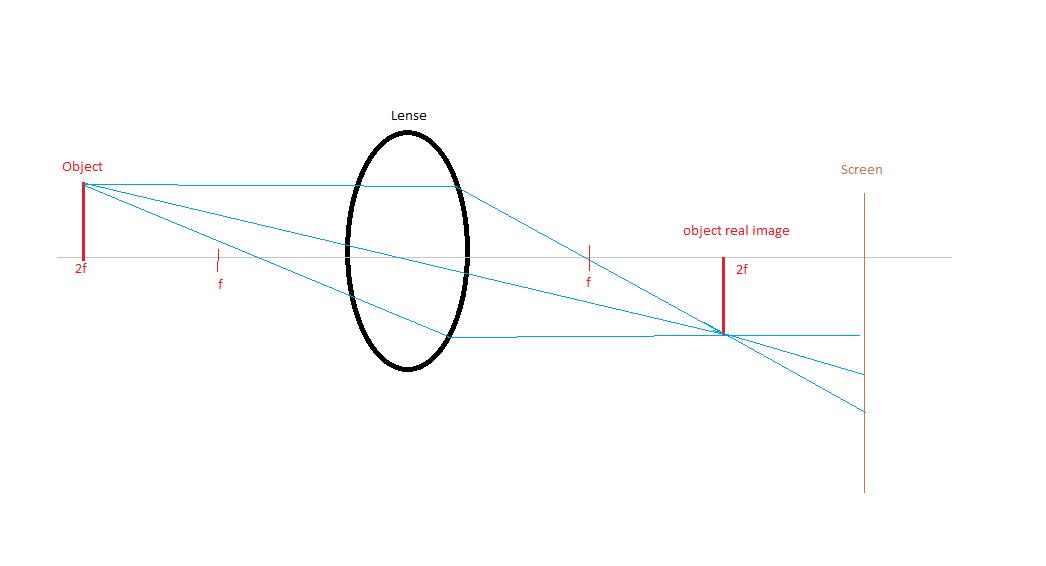
In the paraxial model of geometrical optics a lens can make all the infinitely many rays from an object point hitting the lens converge to a single infinitely small geometrical point. This is the definition of the image point in the world of paraxial optics. We can find the position of the image point by computing where the rays cross. Since all paraxial rays from the object point cross at this point, we can find the position of the image point by choosing only two arbitrary rays out of all the infinitely many rays and compute where these two rays cross. Two paraxial rays are enough to find the position of the image point, but they are not enough to make an image. To make the image itself, we need to have a crossing of all the infinitely many paraxial rays from the object point. In real life we may see images at other places, but this can only be studied using the model of wave-optics.
If a receiving plane is displaced a little from the sharp image point, all the infinitely many paraxial rays go on, spreading out from the image point, filling a cone of light, making a circular or elliptical patch of illumination in the displaced receiving plane.
An extended object consists of infintely many object points. If we consider two points close to each other, we now see two circular patches in the displaced receiving plane, each patch contributing its own illumination. At the places where these patches overlap, the resulting illumination is the sum of the illumination due to each patch. The overlap of the patches is given by the distance between the two points in the object. If the two points are very close to each other, the patches will overlap almost completely, and may seem to be one patch instead of two. We may therefore think that there is only one object point instead of two close points. In the displaced receiving plane we have therefore lost the ability to detect small detail, namely that there are really two close points instead of one, and we would feel that the image in the displaced receiving plane is blurry. If the intensity distributions of the patches are known, it is possible to use computer image processing techniques to retrieve the sharp image from the blurry picture.