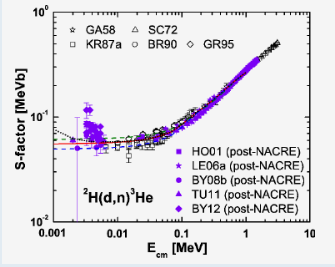
The title is a bit sloppy (easier), but it is a very good question when you mention cross section.
First - it is more convenient to speak not about cross section, but so called astrophysical S-factor. The cross section goes down like hell ($\exp$) with decreasing the energy. This S-factor removes problems of Coulomb barrier and a natural energetic dependence and one can draw almost flat graphs (sometimes).
$$S(E) = \sigma(E) \exp(2\pi\eta) E$$
See - the d-t reaction is 100x more probable (at low energies) than $t-t$ or $d-d$, as you claimed.
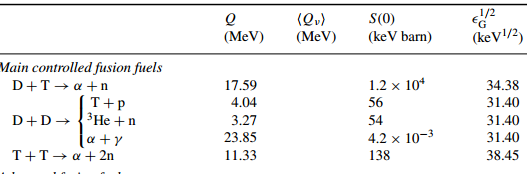
In $^3H - ^{3}H$ scattering, the system feels itself as being $^6He$ in some not well defined state and it has tendency to either just scatter or to "mutate" into some $^6He$ existing state. Energetically, it is possible just to go to (3) lower energy states and waste the remaining energy arbitrarily. Look.
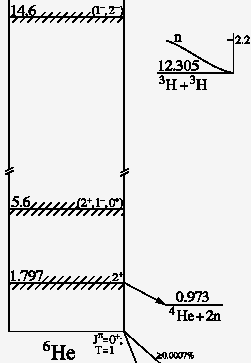
The same happens to $^3H - d$ system, that thinks it is actual $^5He$, but the situation is a little bit different:
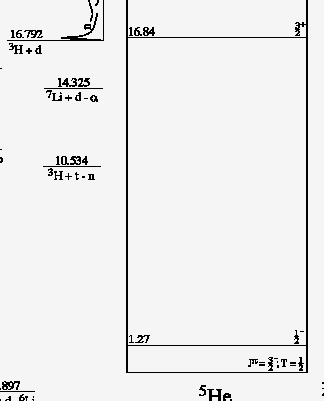
The system appears just around the existing level (resonance) 16.84 MeV and this enhances tremendously the time the system stays there. And this way also the probability of a transfer to another state or of irradiation of a neutron. Look at the S-factor:
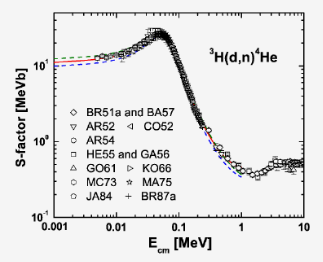
It peaks at about 50 keV by two orders of magnitude, when compared to $d-d$: