Motion of a particle falling along a rough circular rail

Question

I’ve recently been reminded of an old disagreement I had with a very well-respected professor at my university back in my student days, I was tutoring for one of his courses and had been assigned the following exercise to solve:

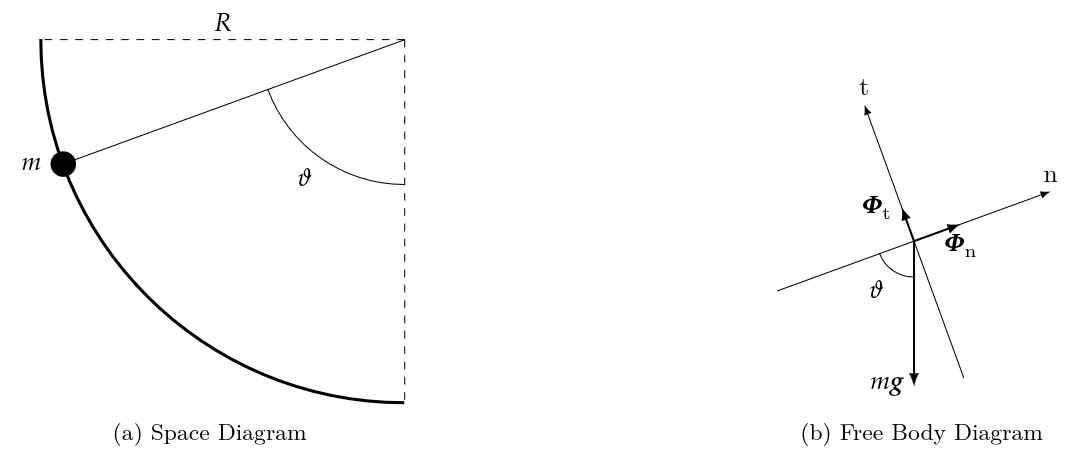
Consider an object of mass $m$ constrained to a quarter-circular rail with radius $R$. The rail is not smooth and the friction coefficient between it and the object is $\mu$. The object is initially placed at the top of the quarter circle ($\vartheta_0 = \frac{\pi}{2}$) and allowed to fall under its own weight ($\dot{\vartheta}_0 = 0$). In the free body diagram here I’m using $\Phi$ to denote the tangent and normal constraint reactions.

My attempt at a solution was to write out Newton’s law along the normal as $\Phi_\mathrm{n} - mg \cos \vartheta = m R \dot{\vartheta}^2$, because I expect that a centripetal force is required to maintain the object on a curved trajectory, while the professor argued that $\Phi_\mathrm{n} - mg \cos \vartheta = 0$ because there is no motion along the normal.

Using my approach, one ends up with a rather messy differential equation, which is why I argued the problem was not appropriate for first-years. Using his approach the equation was a lot more manageable.

At the time I just acquiesced and showed the students his way of solving the problem, but I’m curious to know if I was actually right. Even now I can’t really find any flaws with my solution, I’m pretty sure that if the sum of forces along the normal was zero, the body would just fall vertically.

Answer 1

I believe you are right. Newton's second law in vectorial from might help here. $$\vec{F}=m\vec{a}. \tag 1$$ Working with polar coordinates $\{r,\theta\}$, where $r$ is the radial distance from the origin and $\theta$ is measured counterclockwise from the positive x-axis, you can picture the problem in the third quadrant. So, the ball is moving counterclockwise, and your angle $\vartheta$ decreases as the angle $\theta$ increases.

To be precise, the relation between $\vartheta$ and $\theta$ is $$\boxed{\theta=\frac{3\pi}{2}-\vartheta~}~~\Rightarrow~~ \boxed{\dot{\theta}=-\dot{\vartheta}~} ~~ \Rightarrow ~~ \boxed{\ddot{\theta}=-\ddot{\vartheta},}~~\mathrm{and}~~\boxed{\hat{\theta}=-\hat{\vartheta.}} \tag2$$

The general expression for the acceleration vector in polar coordinates is $$\vec{a}=\frac{d^2 \vec{x}}{dt^2}=\left(\ddot{r}-r\dot{\theta}^2\right)\hat{r}+\left(r\ddot{\theta}+2\dot{r}\dot{\theta}\right)\hat{\theta}. \tag3$$ See https://proofwiki.org/wiki/Acceleration_Vector_in_Polar_Coordinates for a proof of equation (3).

Or, in terms of $\vartheta$, using equation (2), we have $$\vec{a}=\frac{d^2 \vec{x}}{dt^2}=\left(\ddot{r}-r\dot{\vartheta}^2\right)\hat{r}+\left(r\ddot{\vartheta}+2\dot{r}\dot{\vartheta}\right)\hat{\vartheta}. \tag4$$ Now, the left-hand side of equation (1) is $$\vec{F}=\left(mg\cos{\vartheta}-F_N\right)\hat{r}+\left(\mu F_N-mg\sin{\vartheta}\right)\hat{\vartheta}, \tag5$$ where $F_N\equiv\Phi_n$ in your notation, and $\mu F_N\equiv \Phi_t$ is the friction force. Substituting equations (4) and (5) into equation (1), we get $$\left(mg\cos{\vartheta}-F_N\right)\hat{r}+\left(\mu F_N-mg\sin{\vartheta}\right)\hat{\vartheta}=m\left(\ddot{r}-r\dot{\vartheta}^2\right)\hat{r}+m\left(r\ddot{\vartheta}+2\dot{r}\dot{\vartheta}\right)\hat{\vartheta}. \tag6$$ The statement that the ball is moving at a constant radius $r=R$ translates to $$\dot{r}=0=\ddot{r}. \tag7$$ Thus, equation(6) gives $$\left(mg\cos{\vartheta}-F_N\right)\hat{r}+\left(\mu F_N-mg\sin{\vartheta}\right)\hat{\vartheta}=-mR\dot{\vartheta}^2\hat{r}+mR\ddot{\vartheta}\hat{\vartheta}, \tag8$$ which gives $$\boxed{mg\cos{\vartheta}-F_N=-mR\dot{\vartheta}^2,} \tag9$$ and $$\mu F_N-mg\sin{\vartheta}=mR\ddot{\vartheta}. \tag{10}$$ Equation (9) is exactly what you concluded.

Main point:

Motion along a constant radius does not mean $a_r$=0; as you can see from equation (3), $$\vec{a}=-R\dot{\theta}^2\hat{r}+R\ddot{\theta}\hat{\theta}.$$