0
$\begingroup$

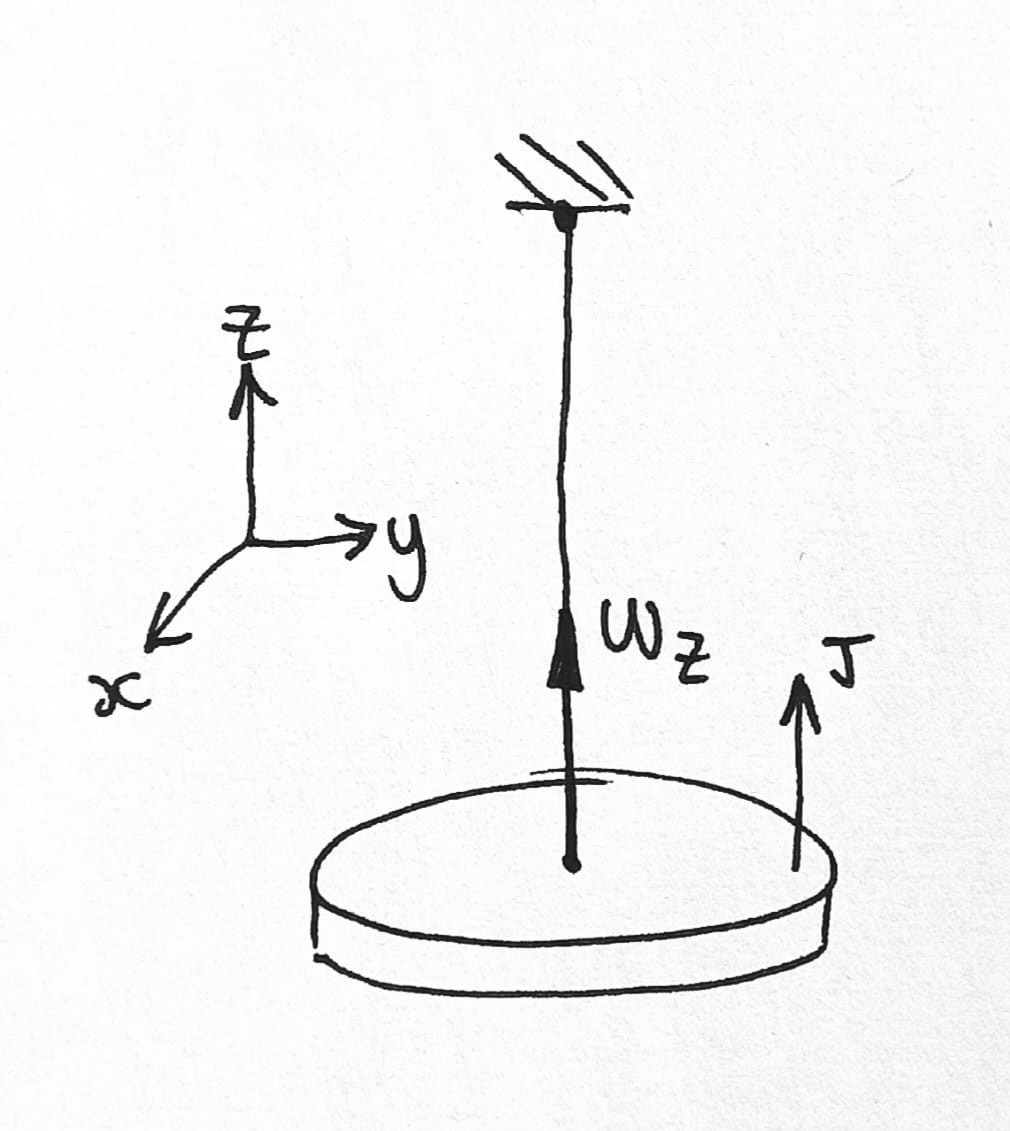
The figure shows a disk spinning with an angular velocity $\omega_z$. The disk is suspended from a long string.

I would like to know the following:

  • How does the disk respond to an impulse $J$?
  • What are the differential equations that describe this response?
Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Let us assume that hitting the disc doesn't cause any loss of energy due to friction. We can conserve angular momentum about the center: $$JR=I_x\omega_x$$ where $I_x=mR^2/4$ So our disc can be thought of as independently rotating about $z$ and $x$ axes. We can find the component of $\omega_x,\omega_z$ to get $\omega_{net}$ along which will be actual axes of rotation of our disc.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for your answer. Can one say that angular momentum is conserved? Does the angular momentum not change because of the impulse? $\endgroup$
    – wSmit
    Commented Nov 22, 2022 at 7:45
  • 1
    $\begingroup$ @wSmit Indeed torque is acted by impulse, but after the impulse is acted, there is no torque. After that, we can conserve the angular momentum $\endgroup$
    – Kshitij Kumar
    Commented Nov 22, 2022 at 7:50

Not the answer you're looking for? Browse other questions tagged or ask your own question.