Going through resonance without damping by controlling the coupling between the oscillator and the generator

Question

To introduce resonance, we usually find in elementary Physics lectures the following system"

The solution of which is: $$\begin{cases}x(t)= \frac{A}{ \omega^2-\omega^2_{0}}sin(\omega~t) ~~~~\omega \neq \omega_{0} \\x(t)= \frac{A}{2m \omega_{0}}.t.sin(\omega_{0}~t) ~~~~\omega =\omega_{0}\end{cases}$$

One generally invokes damping to overcome the diverging amplitude of the solution. But if we model the external force generator by a spring-mass oscillator, the unbounded amplitude of the solution disappears.

My claim is that the classical explanation for resonance does not cover all cases. It is perfectly possible to have a mass-spring system driven at the resonance frequency by a generator, without the system exhibiting unbounded amplitude. The determining factor is not only the frequencies of the generator and system, but also the coupling between the two systems.

There are two possible couplings. The rigid and the soft one.

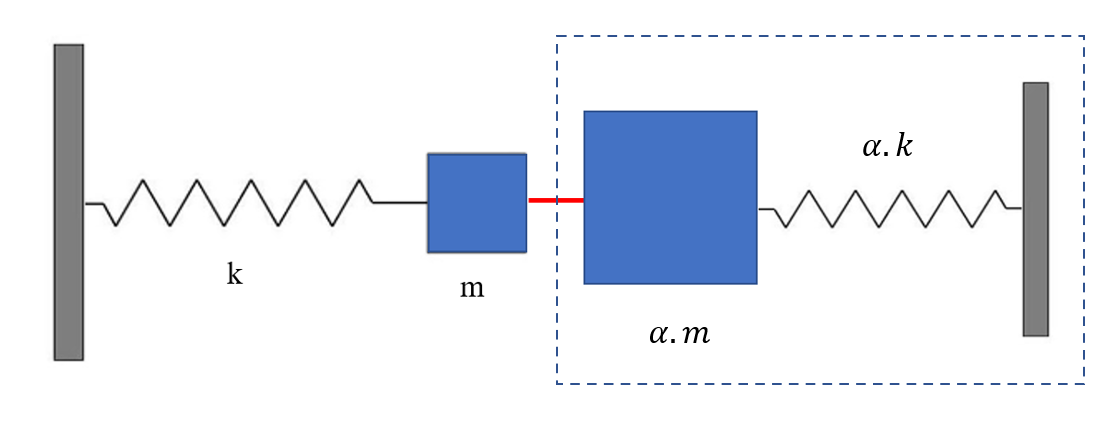
Rigid coupling

The generator is the mass-spring system in the dashed box on the right. It is connected to the oscillating mass-spring system on the left by a rigid rod.

We chose the coefficient $\alpha$ to be very large to insure an ample amount of energy in our generator and minimize any feedback effects. This generator oscillates at the eigen frequency $\omega_{0}=\sqrt{ \frac{k}{m} } $ of the mass-spring system. So the oscillations of the mass m should exhibit the resonance as in the previous case. But it does not.

This system is equivalent to a mass coupled to two different springs in parallel.

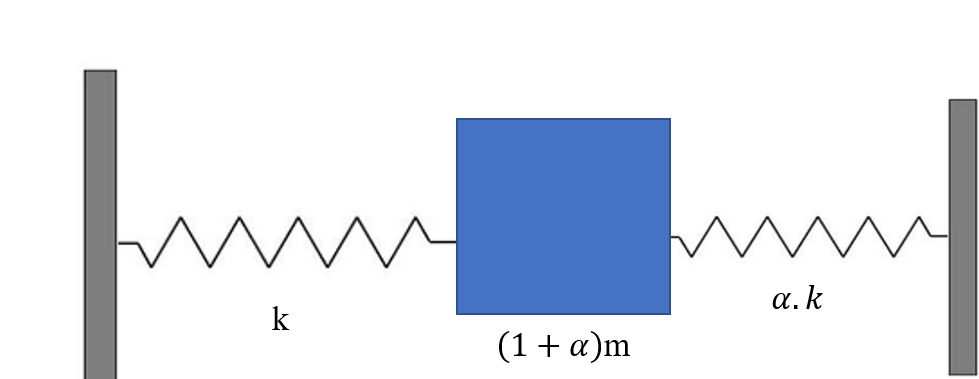
The solution is well known and is non resonant. For the mass m, one gets:

$$ x(t)=A~cos(\omega_{0}~t+ \varphi )$$

This generator oscillates at $\omega_{0}$ both unloaded and loaded and can drive the spring-mass (k, m) without having the amplitude diverging. The result is independent of $\alpha$.

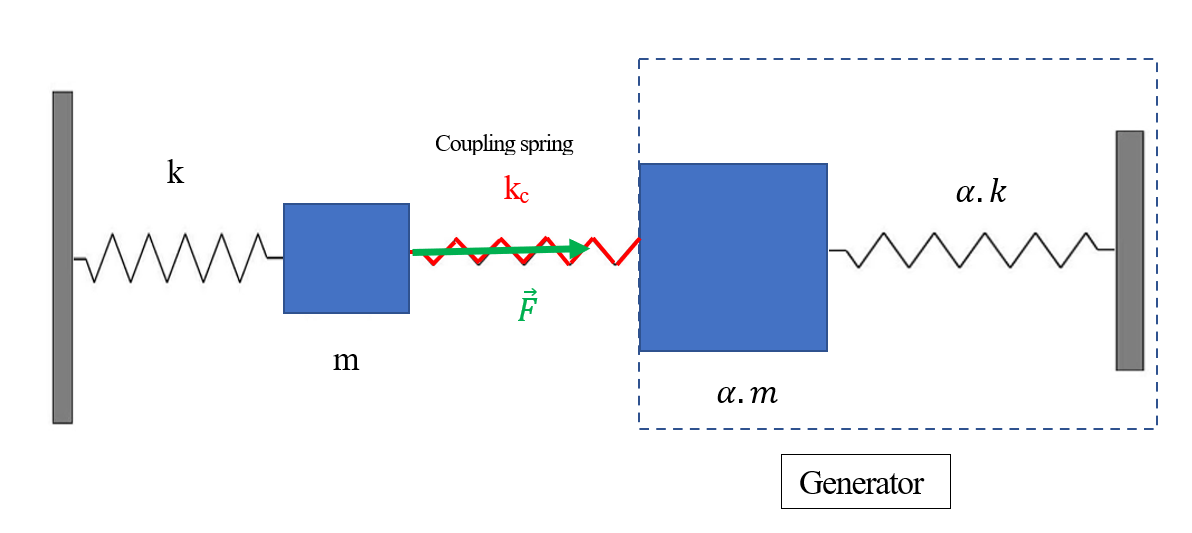
For the second model, the spring-mass generator is connected by a coupling spring $k_{c}$ to the oscillating mass.

Soft coupling

Again, the unloaded generator oscillates at $\omega_{0}$ and has sufficient energy and inertia both controlled by $\alpha$. The solution is again well known. it reads:

$$ \beta = \frac{k_c}{m} $$

$$ x_{m}(t)= \frac{{x_M}(0) \alpha }{ \alpha +1} \big(cos( \omega _{0}~t) -cos( \sqrt{\frac{ \alpha~\omega^{2} _{0}+ \alpha . \beta + \beta }{ \alpha } } t)\big) $$

And for the generator:

$$ x_{M}(t)= \frac{{x_M}(0) \alpha }{ \alpha +1} \big( cos( \omega _{0}~t) - \frac{1}{\alpha} cos( \sqrt{\frac{ \alpha~\omega^{2} _{0}+ \alpha . \beta + \beta }{ \alpha } } t)\big) $$

A series expansion in terms of $\beta$ and $\alpha$ gives:

$$\begin{cases} \beta \mapsto 0 \\\alpha \longrightarrow \infty\end{cases} \Longrightarrow ~~x_{m}(t)= \frac{ \beta x_{M}(0)}{2 \omega _{0}} t.sin(\omega _{0}~t)+O( \frac{1}{ \alpha })O( \beta^{2} )$$

So we recover the ever growing amplitude of the classical resonance. However, it is just an approximation, the full solution does not grow for ever. A closer analysis shows a two frequencies beat response, the ever growing amplitude of the solution is just an approximation at the origin of the envelope by its tangent. It is noticeable that in this case, the frequency of the generator changes, indicating that the energy is flowing back into the generator.

Conclusion

The frequency of the generator matching the frequency of the oscillator is necessary but not sufficient for the resonance to occur. The controlling factor is the coupling between the two systems.

My question:

1. Did I go wrong somewhere?
2. What would be the equivalent of a hard/ soft coupling in electricity?
3. Any references on the subject would be welcome.

Answer 1

1. The main problem I see in your (not)equivalent models is that you start with a forced model and try to find an equivalent model with no external force.

   Comparison between the original system and the free system with hard-coupling. Let's write the dynamical equations of the original system,

   $m \ddot{x} + k x = F(t)$

   and the system with the hard coupling,

   $m \ddot{x} + k x = F(t)$
   $\alpha m \ddot{x}_{\alpha} + \alpha k x_{\alpha} = -F(t)$,

   with the rigid constraint, forcing the displacement of the two masses to be equal $x(t) = x_{\alpha}(t)$. Summing these two equations, we get

   $(1+\alpha)m \ddot{x} + (1+\alpha)k x = 0$ $\qquad$ and thus $\qquad$ $m \ddot{x} + k x = 0$.

   This is a free oscillator and thus oscillates with its natural frequency, if the initial conditions are not zero. The (non)equivalent problem is not forced, so we can't even study its frequency response, without an external force.

   Model of the generator. You could provide an external force if you move the right wall where the "generator spring" is clamped. This way, you could go from a zero-order model of the generator (you directly prescribe the output force), to a dynamic model of the generator (as a second order spring-mass model and damping, if needed) where you controlled the prescribed displacement of the right wall to match the desired force on the system. The dynamical equations of this system read

   $m \ddot{x} + k x = F(t)$
   $\alpha m \ddot{x}_{\alpha} + \alpha k (x_{\alpha} - x_{wall}) = -F(t)$,

   and summing, with the rigid constraint,

   $(1+\alpha)m \ddot{x} + (1+\alpha)k x = \alpha k x_{wall}$ $\qquad$ and thus $\qquad$ $m \ddot{x} + k x = \dfrac{\alpha}{1+\alpha}k x_{wall}(t)$.

   Now we can talk about matching conditions to get the desired force from the input displacement of the wall, i.e.

   $F(t) = \dfrac{\alpha}{1+\alpha}k x_{wall}(t)$

2. For any formal similarity between the mass-damper-spring mechanical system and an electric one, I'd rely on the RLC circuit with a resistor, a capacitor, and a inductor in series with a tension generator with prescribed tension $e(t)$

   $L \ddot{i} + R \dot{i} + \dfrac{i}{C} = \dot{e} $.

3. I have no peculiar reference, but I hope we've found out the main problem of your (non)equivalence.

   For mechanical systems, I'd suggest you to look at experimental activities for structure identification through modal analysis, and the choice of the actuators.

   For a kind of Bible of experimental activities and sensors in engineering, I'd suggest Doebelin, Measurement systems, application and design, McGraw-Hill Publishing Company.

TLDR

The main problem is that you go from a forced system to a free system. If you recover the equivalence by introducing forcing in the system, as I did with the motion of the right wall, now we can interpret the equivalent model as a more detailed model of the force generator and talk about the matching conditions between the characteristic of the generator and the system you need to force, to get the desired force introduced into the system from the displacement of our displacement input.