Why is the formula of (secondary) maxima of single slit diffraction the same as the minima of double slit interference?

Question

We say that, in the Young's Double Slit Experiment -

destructive interference (dark fringe) occurs at every $(n+{1\over 2}){\lambda D \over d}$. However, in the single slit diffraction experiment -

Secondary maxima (bright fringe) occurs at $(n+{1\over 2}) {\lambda \over a}$ where a is the distance between $NP$ and $LP$. Also, the central maxima is twice as wide as the other secondary maxima.

I have 3 doubts -

• Why is it the opposite in both experiment? (i.e. maxima occurs for multiple of $\lambda /2$ in single slit diffraction but for YDSE multiples of $\lambda /2$ are where dark fringes occur).
• Why is the central maximum in diffraction is twice as wide as the other secondary maxima?
• Why is diffraction measured in radian while fringe width in mm?

I need help because my school textbook does not give appropriate/enough explanation on the questions stated above.

Answer 1

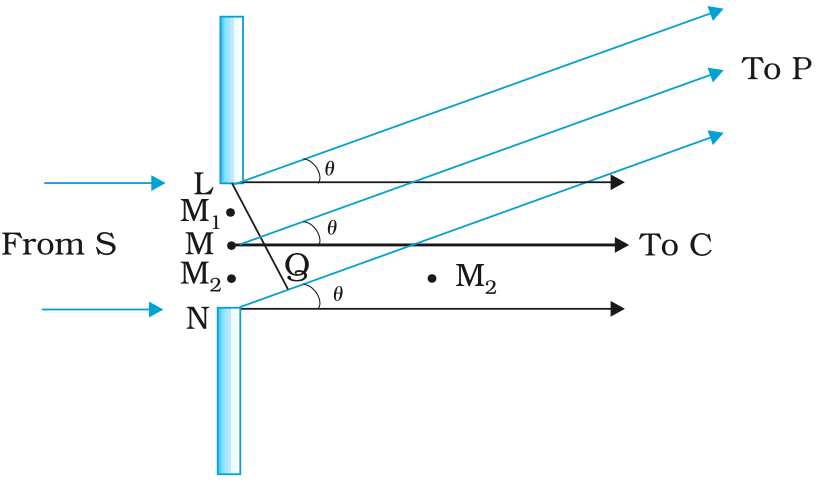
Why is it the opposite in both experiment? (i.e. maxima occurs for multiple of λ/2 in single slit diffraction but for YDSE multiples of λ/2 are where dark fringes occur).

When two point sources separated by some distance $d$ produce waves in phase, they will produce constructive interference at any point $P$ for which the path difference from the sources is $n \lambda$. I believe this is more or less clear, do you see it? Since two very narrow slits may be considered, for this purpose, as point sources, this results also applies for Young's (very narrow)double-slit experiment.

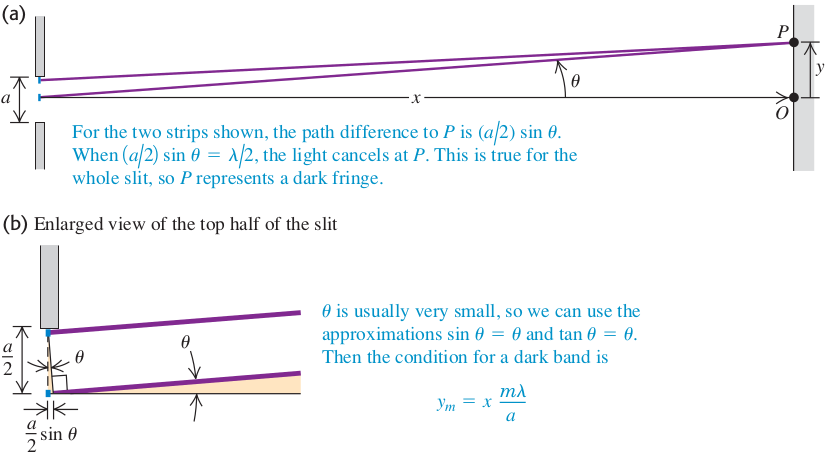
When you have 1 slit with finite width, the usual approach is to consider the slit to be divided into several tiny strips (point sources) each of which produce waves. The following image is taken from Young and Freedman's book, in which the topic is nicely explained), considering 2 little strips in the slit, but the same reasoning applies considering 4, 6, 8,... strips yielding that you get dark bands at $y_m = x \frac{m \lambda}{a}$. In the book you may find a more detailed explanation.

Why is the central maximum in diffraction is twice as wide as the other secondary maxima?

You may look at this using the formula. Basically it is because the central maxima goes from $m = -1$ to $m = 1$ (it jumps two values of $m$) while the secondary maxima jumps only 1 value in $m$ (e.g. from $m=1$ to $m=2$.)

Why is diffraction measured in radian while fringe width in mm?

I don't know what you mean by this. You may measure in radians the angular position of some maxima/minima from the normal line coming from the slits. You can do something similar to measure the width of the lobes in the diffraction pattern. Equivalently, you may measure the locations or the widths of the lobes in mm as distances over the screen.

Answer 2

1. If light from the two slits is in phase (or if their phase differs by an exact number of $\lambda$) then they interfere constructively and it is bright. If the light from the two edges of the single slit differs by an exact number of $\lambda$ then the integrated contributions across the slit cover a complete number of cycles and sum to zero so it is dark.
2. The single slit diffraction formula contains a term $sin \theta / \theta$. (Called the sinc function) This has zeros whenever $\theta = n \pi$ as then $\sin \theta=0$ and gives dark fringes EXCEPT for the central peak where it is $\sin 0 / 0$ as both numerator and denominator are zero. Taking the limit shows that this gives 1: a bright fringe.
3. You can use radians or mm whichever is convenient - they're just connected by trignometry. In figures like the one you show then angles in radians are easy to visualise. If you stick up a screen and look at the fringes it's easiest to measure their visible positions in mm.