Double slit diffraction and interference patterns

Question

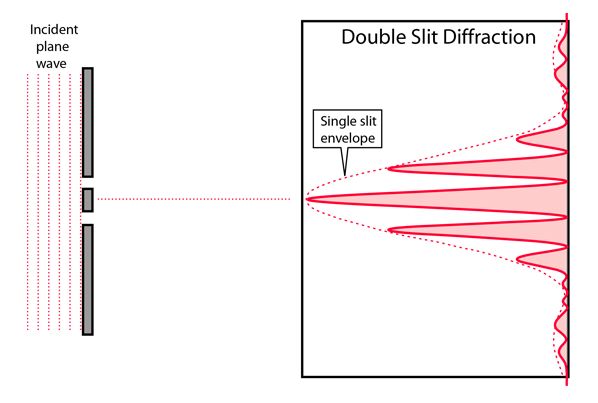
i'm a highschool physics student trying to understand diffraction and interference etc.

I'm confused about this (in the context of the double slit experiment): if an underlying interference pattern occurs, e.g there are alternating maxima and minima all with the same intensity, how come when the single slit modulating envelope is applied and the interference pattern is subsequently shaped to the envelope, the intensity of subsidiary maxima in the double slit experiment decrease? e.g the bright dots that we see with our eyes fade as you go further out from the principle maxima. Additionally, in the attached image, are the maxima that are under the main part of the single slit envelope seen distinctly or do they blur together to give the bright central maxima that the double slit experiment produces.

Forgive my writing, it's just horribly difficult to express this question.

-Thank you

Answer 1

I think confusing arises from your diagram. The two plots are not to scale.

The single slit diffraction pattern is the smooth envelope shown (dashed line). The intensity is unmodulated.

When you add the second slit, the interference pattern appears (as a modulation of the waveform within the envelope). That is the solid line.

But: the envelope has to get "bigger", and by bigger I do NOT mean wider, rather, it needs to have more intensity (duh: there is more light).

By how much? Well, energy is conserved, so the total area under the curve has to double (there are two slits, after all).

Since the average of $sin^2(x)$ over a cycle is $\frac 1 2$, and there is twice as much light, you could guess the peak intensity needs to be 4 times brighter, and you would be correct.

The reason for this is that interference occurs because we add wave amplitudes, and when they are in-phase (out-of-phase), constructive (destructive) interference occurs. Constructive interference adds 2 amplitudes, $A$, and:

$$ A_{con} = A + A = 2A$$

and the intensity is the square of the amplitude:

$$ I_{con} = A_{con}^2 = 4A^2 = 4I $$

where $I$ is the single slit maximum.