Why are there interference patterns inside a diffraction envelope?

Question

When double-slit diffraction occurs, there are interference patterns inside, say, the central diffraction maxima (or envelope). I am trying to understand how these interference fringes are created.

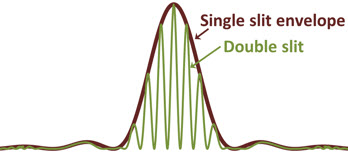
Here is what I know: each individual slit in the double-slit setup produces a diffraction pattern, and these two diffraction patterns overlap very closing. Assuming Fraunhofer diffraction (the diffraction pattern is viewed far from the diffracting slits), the waves coming from each slit into the central diffraction envelope will interference and produce a series of bright and dark fringes.

My question: can someone explain “easily” how these overlapping diffraction patterns produce interference fringes inside a diffraction envelope?

I feel comfortable mathematically integrating the fields using the Fresnel diffraction integral to arrive at the Fraunhofer condition. When I say easily, I am looking for a more physical explanation if possible.

Answer 1

In a simplistic model, you can view destructive interference for a two-slit situation as arising from one of two possible events: Either light from a single slit is destructively interfering (and hence light from the other slit will as well, since the off-set is usually ignored), or light leaving both slits interfere with each other. The smaller "inner" interference pattern is caused by interference between the slits, the second option above. This is contrast to the diffraction envelope which, as you stated, is caused by interference for a single slit.

For example, if light leaving the left-most edge of the left slit has a path length difference of $\lambda/2$ with respect tho the corresponding edge of the right slit, then light from these paths will completely destructively interfere. Furthermore, every point in one slit has a pair in the other slit that causes destructive interference (with the same path length difference).

You'll notice the first inner minimum occurs at a smaller angle than first single-slit minimum. This is consistent with $d\sin\theta=\lambda/2$ producing a smaller angle than $(a/2)\sin\theta=\lambda/2$ since the slit separation $d$ is larger than the slit width $a$. These equations yield the first "interference" and first "diffraction" minima for two slits, respectively.