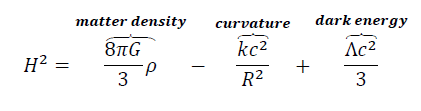Why are the radius of the observable universe and radius of curvature of dark energy almost the same?

Question

If you take the Einstein equation,

$R_{\mu\nu} - \frac12 Rg_{\mu\nu} = \frac{8\pi G}{c^4}T_{\mu\nu}$

and plug in the estimated vacuum energy of $10^{-9} J/m^3$ for $T_{\mu\mu}$, you get a spatial curvature of about

$R_{ii} \approx 2.07 \cdot 10^{52} m^{-2}$

If we supposed pure de-Sitter space, the spatial cross-sections would be spherical, right? And the curvature would be $1/r^2$, which would give a radius of curvature of

$r \approx 6.94 \cdot 10^{25} m$

Multiply that by $2\pi$ and the circumference would be pretty darn close to the estimated size of the observable universe of $8.8 \cdot 10^{26} m$.

But as I understand it, the "size of the observable universe" means something completely different: it's the radius from any point at which the entire light cone "disappears", that is, even the null direction that should be moving toward us is instead comoving with us, due to the (not necessarily accelerating?) expansion of the universe. Which is something I don't fully understand (without acceleration, ie. curvature, how can you get deviation of light cones over distance?), but anyway it certainly is not the radius of curvature.

So is it just a coincidence that these two numbers happen to be of the same order of magnitude? Or is there a connection I'm not grasping?

Answer 1

The Einstein equation is also the first Friedmann equation. So what the OP has done is write the de Sitter (dark energy only, no matter, flat universe) solution.

It is well known that the de Sitter characteristic length $l_\Lambda$ is related to the cosmological constant $\Lambda$:

\begin{equation} {\Lambda} = \frac{3}{l_\Lambda^2} \end{equation} That's the $1/r^2$

Now, working out the radius of the current observable universe does depend on what parameters you plug in (aka 'model dependent') but there is general acceptance the radius $R_{obs}\approx46Gly\approx 4.4\times10^{26}m$.

TLDR: So yeah, $2\pi l_\Lambda\approx2\times R_{obs}$. But the 'connection' ($\pi$) is just that the current recessional velocity of the particle horizon is $\approx3.47c$.