Describing forces in rolling

Question

Consider a wheel on a frictionless horizontal surface. If we apply a horizontal force (parallel to the surface and above the level of the center of mass), what happens to the wheel? Does it roll or slide forward or rotate only or does any other phenomenon happen? Please guide me. Also draw a free body diagram.

Note: This is a thought experiment. If the question is not satisfying, I am sorry for that and please guide me.

Answer 1

Consider the Free Body Diagram:

where:

• $F$ is a driving force
• $mg$ the weight of the wheel
• $F_N$ a reactive force, called the Normal force
• $F_f$ a friction force

We can now establish some force/torque balances.

In the vertical ($y$) direction, there no motion because with $\text{N2L}$:

$$\Sigma F_y=F_N-mg=0 \Rightarrow F_N=mg$$

The friction force is usually modeled as:

$$F_f=\mu F_N=\mu mg$$

As long as no slipping occurs, $\mu$ is the static friction coefficient.

Now, looking at the balance of torques about the CoG (marked as $+$) we have a net torque balance $\tau$:

$$\tau=F\lambda-F_f R=F\lambda-\mu mg R$$

As per $\text{N2L}$ (applied for rotation) this causes angular acceleration $\alpha$, in the clockwise direction:

$$\tau= I_w \alpha \Rightarrow \alpha=\frac{\tau}{I_w}$$

where $I_w$ is the inertial moment of the wheel.

Note that $\alpha=\frac{\text{d}\omega}{\text{d}t}$.

Without slipping/sliding, we can write $v=\omega R$ and also:

$$a=\alpha R$$

Or:

$$a=\frac{F\lambda-\mu mg R}{I_w}R$$

Answer 2

It should roll. Whether it will be rolling with sliding or pure rolling we don't know until we know what height the force was applied at. Breaking the motion into Translation and Rotation, we can write one force and torque equation each for both respectively as such-

$F=ma$

$ \tau=I\alpha=rF$

We can also calculate the condition on the height at which you must apply the force for pure rolling by equalling the net acceleration of the bottom most contact point to $0$. This point will have two accelerations, one from rotation ($=R\alpha$) and one from translation ($=a$). Notice their directions are opposite, so for net acceleration of zero they must be equal. So, we have

$F=ma$

$a=\frac{F}{m}$

$I\alpha=rF$

$\alpha=\frac{rF}{I}$

For pure rolling, $a=R\alpha$

Substituting and rearranging, we have

$r=\frac{I}{mR}$

Answer 3

Look neglecting friction makes it simple. We have to think only about the applied force for rotational motion as well as translational motion.

So since there is an external force , the body will have some translational motion in the forward direction. This will happen for sure and we can find the acceleration using

F=ma

Now we have to look for the rotational motion. If the force was applied exactly at the centre of mass of the body, there would be no rotation at all (cause is in your previous question).

But since the force is applied at some distance above the centre of mass there would be some Torque(same cause , as given in your question) and hence the body will rotate.

So in your case , there would be both rotation as well as translation or you can say that the body is rolling. For pure rolling I1mbo has given correct explanation.

Thanks for asking. Hope it helps.

Sorry for no fbd's.

Answer 4

The equation of motions are:

$$\sum_M F_y=m\frac{d}{dt}\,v-F=0$$

$$\sum_M \tau_x=I\,\frac{d}{dt}\,\omega-F\,d=0$$

Thus :

$$\dot{v}=\frac{F}{m}\tag 1$$ $$\dot{\omega}=-\frac{F\,d}{I}\tag 2$$

Roll condition:

$$v=\omega\,R$$

Slide condition :

$$v \lessgtr \omega\,R$$

$\Rightarrow$ $$\dot{v}\lessgtr \dot{\omega}\,R\tag 3$$

with equations (1) ,(2) and (3) you get

$$F\left(\frac{1}{m}+\frac{R\,d}{I}\right)\lesseqgtr 0\tag 4$$

where $=$ is for roll and > for silde

Thus:

$F=0$ for rolling

$\Rightarrow$

$$v=v_0\,t\quad,\omega=\frac{v_0}{R}\,t$$ where $v_0$ is the initial velocity .

and $F> 0$ for slide

Answer 5

Does it roll or slide forward

It depends on where the force is applied and the moment of inertia of the wheel, together which determine whether or not the conditions for rolling without slipping are met without the need for friction.

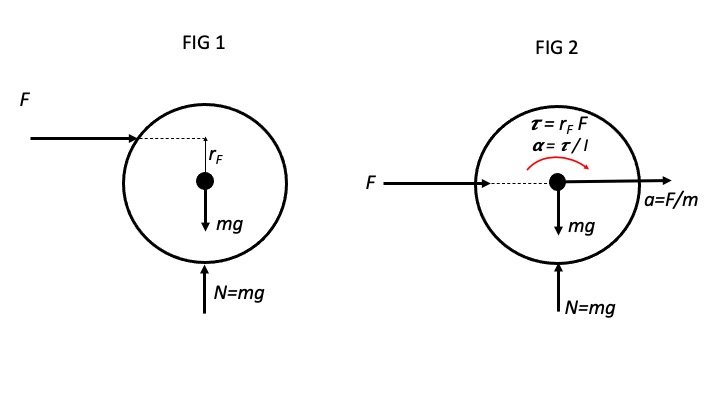
Fig 1 shows the forces acting on the wheel in the absence of any friction (static, kinetic, or rolling) or air drag, where the line of action of the force $F$ is an arbitrary distance $r_F$ above the center of mass of the wheel (assumed the geometric center). The weight of the wheel is balanced by the normal reaction force of the surface it is on. The only external horizontal force acting on the wheel is $F$ whose line of action is a distance $r_F$ from the center.

Fig 2 shows the equivalent force system where the line of action of $F$ is moved to the center of mass (COM) with a couple (torque) about the COM accounting for the original torque of $F$ about the COM. The wheel has a linear and angular acceleration as shown (where $I$ is the moment of inertia of the wheel about the COM).

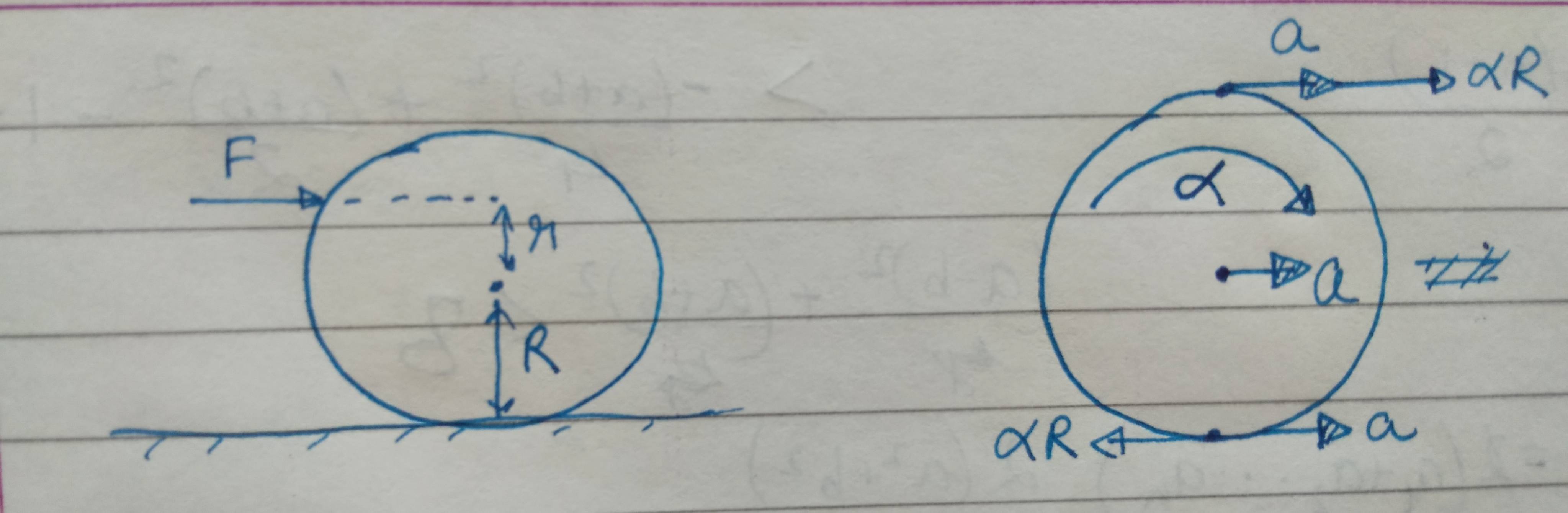
The condition for rolling without slipping is, where $r$ is the radius of the wheel

$$a=\alpha r\tag{1}$$

This is based on the distance travelled by a point on the surface of the wheel for a complete revolution has to equal the horizontal distance traveled by the COM in order for there to be no relative motion (slipping) between the wheel and the surface.

Then since

$$\alpha=\frac{\tau}{I}=\frac{r_{F}F}{I}\tag{2}$$

Where $I$ is the moment of inertia about the COM

and $$a=\frac{F}{m}\tag{3}$$

substituting equations (2) and (3) into (1) we have

$$\frac{F}{m}=\frac{rr_{F}F}{I}\tag{4}$$

The moment of inertia $I$ of the wheel will be $I=kmr^2$ where $k\le 1$ depending on the distribution of the mass of the wheel about its COM. thus equation (4) becomes

$$\frac{F}{m}=\frac{rr_{F}F}{kmr^2}\tag{5}$$

which simplifies to

$$\frac{r_{F}}{kr}=1\tag{6}$$

for a thin walled disk (hoop) $k=1$ and $r_{F}/r=1$. So if the force $F$ is applied to the rim of the hoop, the hoop will roll without slipping. For a solid disk $k=1/2$ and the disk will roll without slipping if the force is applied at half the radius from the COM. And so forth. Otherwise the wheel will slip while rolling.

Hope this helps.