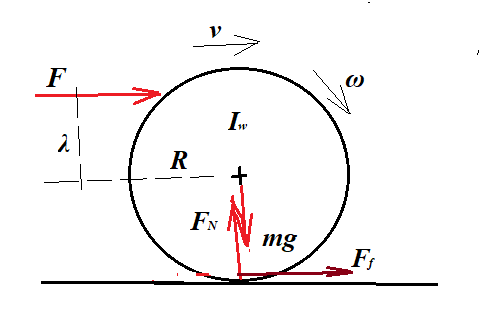
Consider the Free Body Diagram:
where:
- $F$ is a driving force
- $mg$ the weight of the wheel
- $F_N$ a reactive force, called the Normal force
- $F_f$ a friction force
We can now establish some force/torque balances.
In the vertical ($y$) direction, there no motion because with $\text{N2L}$:
$$\Sigma F_y=F_N-mg=0 \Rightarrow F_N=mg$$
The friction force is usually modeled as:
$$F_f=\mu F_N=\mu mg$$
As long as no slipping occurs, $\mu$ is the static friction coefficient.
Now, looking at the balance of torques about the CoG (marked as $+$) we have a net torque balance $\tau$:
$$\tau=F\lambda-F_f R=F\lambda-\mu mg R$$
As per $\text{N2L}$ (applied for rotation) this causes angular acceleration $\alpha$, in the clockwise direction:
$$\tau= I_w \alpha \Rightarrow \alpha=\frac{\tau}{I_w}$$
where $I_w$ is the inertial moment of the wheel.
Note that $\alpha=\frac{\text{d}\omega}{\text{d}t}$.
Without slipping/sliding, we can write $v=\omega R$ and also:
$$a=\alpha R$$
Or:
$$a=\frac{F\lambda-\mu mg R}{I_w}R$$