It should roll. Whether it will be rolling with sliding or pure rolling we don't know until we know what height the force was applied at. Breaking the motion into Translation and Rotation, we can write one force and torque equation each for both respectively as such-
$F=ma$
$ \tau=I\alpha=rF$
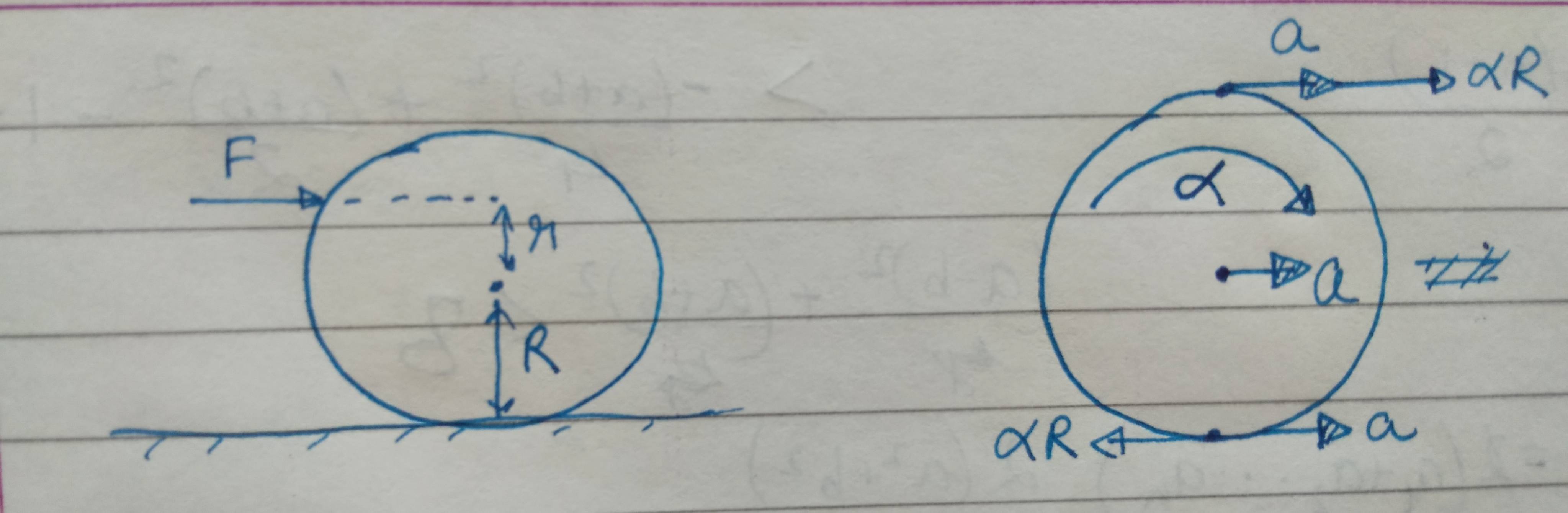
We can also calculate the condition on the height at which you must apply the force for pure rolling by equalling the net acceleration of the bottom most contact point to $0$. This point will have two accelerations, one from rotation ($=R\alpha$) and one from translation ($=a$). Notice their directions are opposite, so for net acceleration of zero they must be equal. So, we have
$F=ma$
$a=\frac{F}{m}$
$I\alpha=rF$
$\alpha=\frac{rF}{I}$
For pure rolling, $a=R\alpha$ Substituting
Substituting and rearranging, we have
$r=\frac{I}{mR}$
