It should roll.
Breaking the motion into Translation and Rotation, we can write one force and torque equation each for both respectively as such-
$F=ma$
$ \tau=I\alpha=rF$
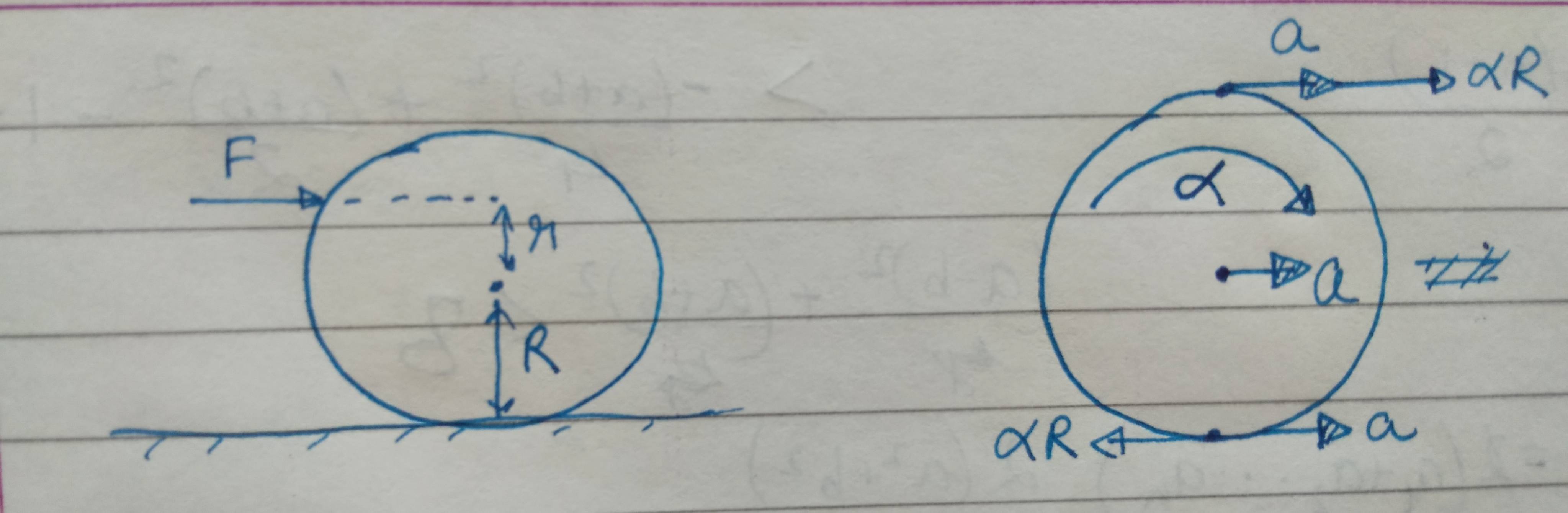
We can also calculate the condition on the height at which you must apply the force for pure rolling by equalling the net acceleration of the bottom most contact point to $0$. This point will have two accelerations, one from rotation ($=R\alpha$) and one from translation ($=a$). Notice their directions are opposite, so for net acceleration of zero they must be equal. So, we have
$F=ma$
$a=\frac{F}{m}$
$I\alpha=rF$
$\alpha=\frac{rF}{I}$
For pure rolling, $a=R\alpha$
Substituting and rearranging, we have $r=\frac{I}{mR}$