Curl of electric field due to dipole?

Question

The field lines of electric field of dipole do seem to curl if seen geometrically. If water flow lines were of such shape, a ball would have rotated in it, doesn't this interpretation of non-zero curl apply here? But we know that curl of electrostatic field is zero. So, how do we conceive it geometrically? I am convinced of it from all mathematical arguments, maybe a more visual meaning of curl would help?

Answer 1

Alternative definition

Whenever you try to find whether a field has a non zero curl, then imagine yourself moving a small charge (or whatever object upon which the field acts) in a very small loop (to be rigorous, it should be infinitesimal). If you end up doing some non zero work when you move the charge across the loop, then it means that the field has a non zero curl at that point ($\vec{\nabla} \times \mathbf E\neq 0$). But if you do no work when you move around a loop, then it means that the field does not have any curl at that point ($\vec{\nabla} \times \mathbf E=0$).

Case of a dipole

Let's say that you place a dipole and thus the dipole establishes its field in the surroundings. Now, if you take any infinitesimal circle and calculate the work done while going around it, you would see that for almost half part of the circle, the field is along the direction of motion, and for almost the other half, it is opposite to the direction of motion. This way, the net work done by the field cancels out which implies that the curl of the field is zero (everywhere).

Fallacy in your argument

You are perceiving the curl as the curving of field. But whenever you estimate the curl of the field by the curvature, you should always consider the a whole loop instead of just going in one direction. In this case, you saw that the field is curving when you go from a point A to point B, but then you should also take into account the curvature when you come back from point B to point A. And this case, both the values exactly cancel exch other and the curl therefore, is zero. Also, if your arguments were true, then every field whose curl would be zero should exist as straight lines and shouldn't curl anywhere, but this isn't true.

Answer 2

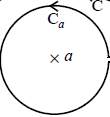
Perhaps you could think this a more visual representation of curl. Nothing like a dipole. Curl describes the flow of a field around a point.

To make sense of this one has to think of the limit as the size of the loop goes to zero at the point $a$ (curl is defined in terms of derivatives, meaning that this limit is implicit). Alternatively, think of the integral of curl over the area, as in Stoke's theorem. An example is the magnetic field around a current carrying wire, for which the curl is zero everywhere but at the wire.