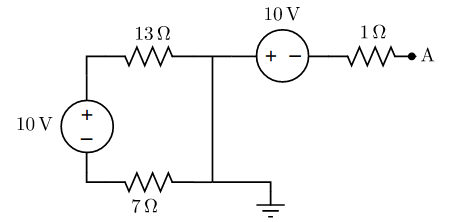
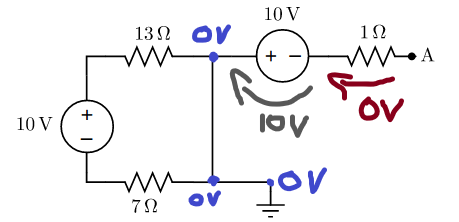
I am trying to calculate the voltage in A, in the following circuit:
That means I have to calculate the voltage at the ground end relative to A, or with other words: "go from ground to A and add or substract each voltage".
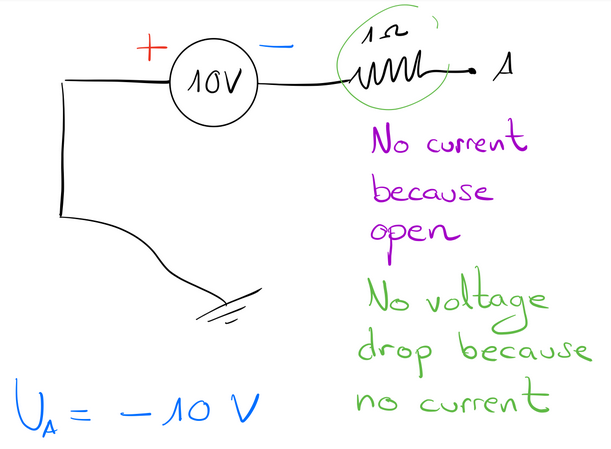
If the loop wasn't there, this is what I can say about $U_A$.
But there is my first confusion, since ground systems are made to close the circuit, and I don't know if that allows a current to actually flow between A and ground.
Or maybe I can consider the space bewteen A and ground like a huge resistance, so there really is no current going on there.
But even assuming that is correct, I don't know how to proceed with the loop, because:
- There will be current in the loop.
- Voltage in the loop must sum zero.
- Each resistance has a different value, so they will have a different voltage drop.
- I don't know how to calculate the current in the loop, so I don't know how to calculate the voltage in each resistance, and hence... I don't know if there is any voltage in the middle connection (which will affect $U_A$).
A teacher said that the cable with nothing in it, behaves like a shortcircuit, so I can just imagine it's not there. That would be very clear if there wasn't a voltage source in the loop.
But the fact that there is a voltage source in the loop makes it hard to believe that there won't be any current and voltage on that side of the circuit.
How exactly does the voltage source on the left side affect the cable that has nothing in it?
UPDATE
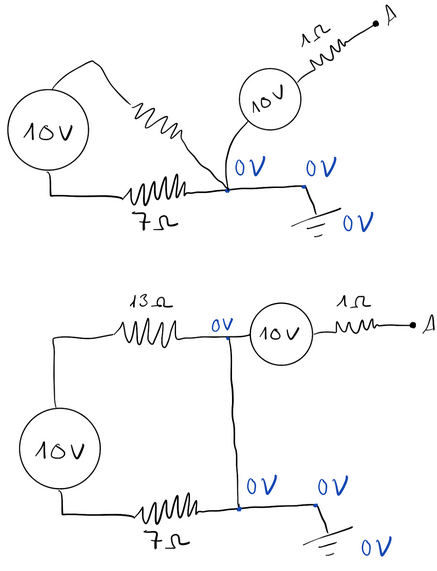
Thanks to uriyaba's and Ofek's answers I now understand that there is no voltage difference in the middle connection.
But Ofek's answer, even though it's very clear, is somehow "jumping over" the confusion. It calculates the current in the loop by assuming that there is no voltage in the center connection, so it is not actually mathematically justifying the fact that there is no voltage there, it's just assuming it.
If we apply Kirchhoff's second law in the loop, it would be an equation with two unknown parameters:
$10V − 13Ω⋅I −7Ω⋅I - x V = 0$
Where $x V$ is the unknown amount in the branch.
So this is not the way to go yet, to know what the value for x is.
There is something else that must come into the game.
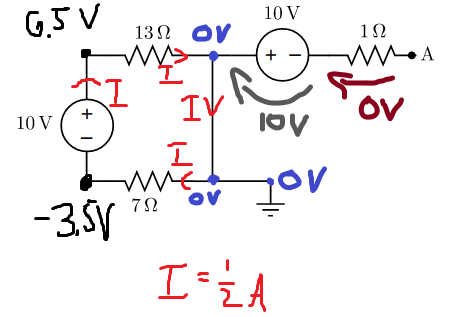
I tried to expand on uriyaba's answer and made this drawing:
But the 0 V confuse me.
If there really where 0 V in that branch, the loop would not have any current because charge carriers wouldn't have any "reason" or "motivation" to cross that connection.
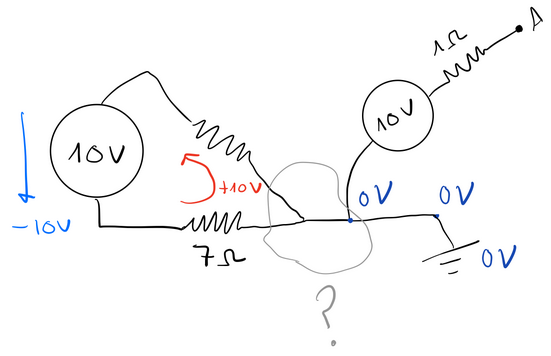
Of course, what is confusing me, is the representation of the circuit.
Maybe this makes it clearer, but I am still not getting what really goes on in that central area:
How can I have "yes voltage difference" and "no voltage difference" at the same spot?
What would a connection like this look like in reality?
If the loop was independent and not connected to anything, I wouldn't have any doubt. Any cable connecting the two resistors wouldn't have a voltage drop (ideally of course).
But the case is, it's in fact connected to another voltage source.