0
$\begingroup$

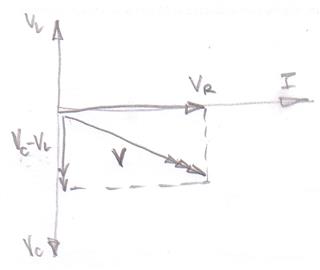
I have this homework problem in my book, it says:

In the circuit shown in the figure, the current flows in the circuit is 0.644A and the the RMS of the AC source is 100V, calculate the reading of the voltmeter.

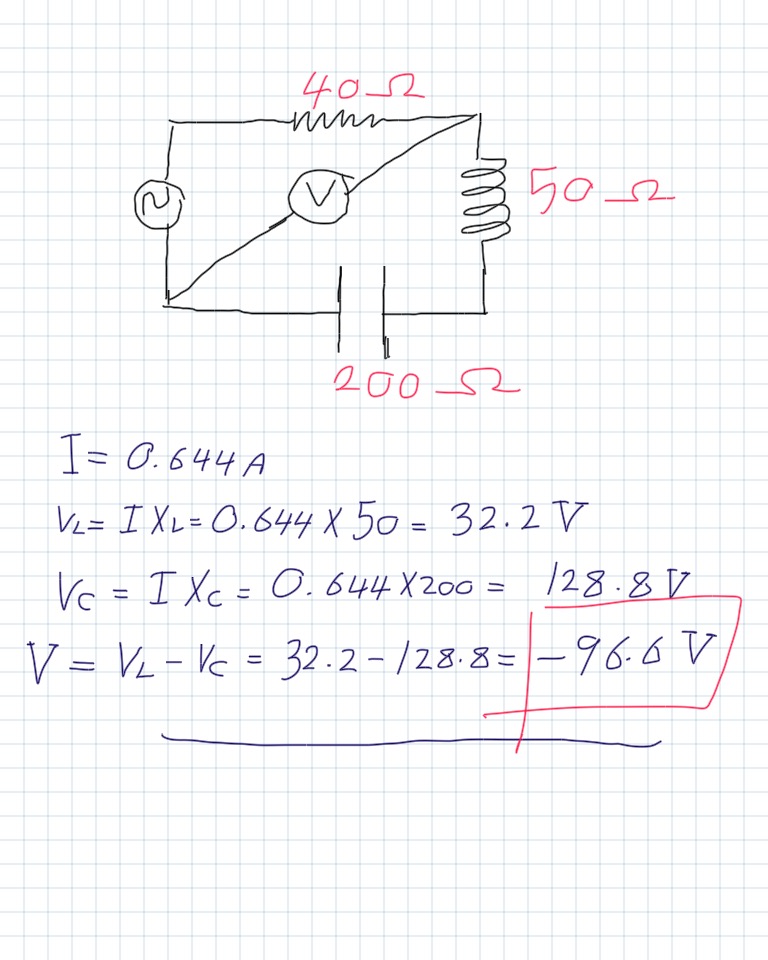
Here is the figure and my attempt to solve the problem, I had solved it with two methods, and I had different values in each one, and I don't know which one is correct. Method one: enter image description here

I calculated the voltage across the capacitor and the inductor branch and got a voltage of 96.6V. But I said to myself to try to calculate the voltage across the AC source and the ohmic resistance branch thinking that it must have the same value as they are connected to the same two points the cpacitor and the inductor are connected to. And I did this: enter image description here (Note that Veff is the RMS of the source) I thought that the branch of the AC source and the resistance has the same voltage as the branch of the capcitor and the inductor, but apparently it doesn't, so why? Did I do something wrong? Or what?

Improve this question
$\endgroup$
1
  • $\begingroup$ In your solution (2) you're assuming that $V_{eff}$ and $V_{R}$ have the same phase so that you can do a simple subtraction of their amplitudes. They're not in phase, so you can't simply subtract their amplitudes. The current through the resistor will be somewhat out of phase with the voltage of the AC voltage source due to the fact that the resistor is in a series circuit with an inductor and capacitor. With problems of this type you always have to remember that you're dealing with different voltages and currents that are generally out of phase with each other. $\endgroup$
    – user93237
    Commented May 18, 2017 at 20:57

1 Answer 1

Reset to default
0
$\begingroup$

The phasor diagram looks like this.

enter image description here

You quite corrected added the phasors $V_{\rm C}$ and $V_{\rm L}$ and found a voltage which was lagging the current $I$ in the circuit bu $90^\circ$.
In the second method you incorrectly subtracted the voltage across the resistor $V_{\rm R}$ from the supply voltage $V$ as you did not include the fact that they are not in phase.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Sorry for replying very late, but what should I do to correct it? I am still unclear. $\endgroup$
    – Asmaa
    Commented May 21, 2017 at 5:43
  • $\begingroup$ You want the voltage across the supply and the resistor which are $90^\circ$ out of phase with one another so use $\sqrt{V^2-V_{\rm R}}$ and you will get your first answer. $\endgroup$
    – Farcher
    Commented May 21, 2017 at 5:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.