I can't understand what is given here. How is the double slit pattern a interference a two single slit and double slit. If we say it is interference of two single slit then it makes sense. But the graph looks different from double slit pattern. Please explain
The "double-slit interference pattern," as defined in this passage, is what you get when you shine light on two slits of infinitesimal width. In reality, you can't ever make slits of infinitesimal width, so whenever you actually perform this experiment, you must account for the fact that your slits have finite width. Shining light through a slit of finite width gives a single-slit interference pattern. So the "actual double-slit interference pattern" (i.e. the pattern you will get in every double-slit experiment you could possibly perform) is a combination of the "double-slit interference pattern" (the ideal one, with infinitesimal slit widths) and two single-slit interference patterns.
Reading the words carefully, I think the text is right.
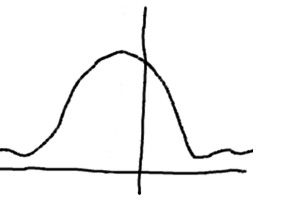
This is the diffraction pattern from the left slit:
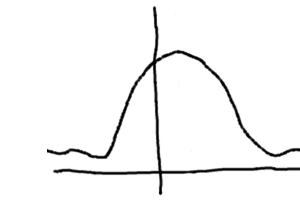
This is the diffraction pattern from the right slit:
right diffraction pattern
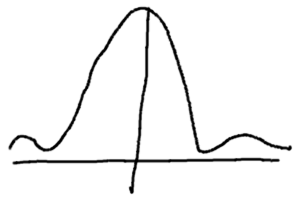
And this is the superposition of both diffraction patterns, just the sum of the previous two:
Up to here it is the classical expected behevior.
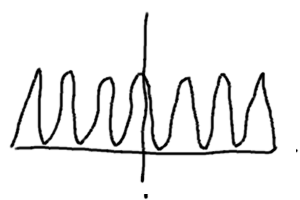
But then there is the double-slit interference pattern:
Combine these two last images (multiply them, I think) and you'll get the one from your book.
The last paragraph about $a$ being much smaller than $d$ is so that the interference pattern is much narrower than the diffraction pattern. If they are of the same magnitude (or $a$ greater than $d$) then the quantum effect is not clearly visible, which is the point of this experiment.
The double-slit interference pattern combines two distinct effects:
1.Interference from the two slits (Double-Slit Interference): This is the primary pattern caused by waves from the two slits interacting constructively and destructively. 2. Diffraction from each individual slit (Single-Slit Diffraction): Each slit acts as an individual source of diffraction, creating its own intensity envelope.
The resulting double-slit pattern is a combination of these effects, leading to a more complex intensity distribution. When light passes through a single slit, it diffracts, spreading out and creating a central bright fringe with dimmer fringes on either side. The result is a central bright region (the widest) and successive smaller, dimmer regions on either side.
With two slits, light waves from both slits interfere. If the path difference between the two waves is an integer multiple of the wavelength, there’s constructive interference (bright fringes). For a half-wavelength difference, there’s destructive interference (dark fringes).
In the double-slit experiment, the interference fringes are modulated by the single-slit diffraction envelope. This means that the fine structure (rapid bright and dark fringes) is due to the double-slit interference and the overall envelope (gradual fading of fringe intensity away from the center) is due to the single-slit diffraction.
Now coming to the question of why the Double-slit pattern Looks Different, If the slits are infinitely narrow, the single-slit diffraction pattern vanishes, leaving pure cosine^2 interference fringes. This would look like equally spaced bright and dark fringes with uniform intensity across the screen.
Real slits have finite width, so the single-slit diffraction creates an intensity envelope that modifies the pattern. The double-slit interference fringes are superimposed on this envelope, meaning some fringes are brighter or dimmer depending on their location within the diffraction envelope.
If you plot the double-slit pattern, you'll notice:
