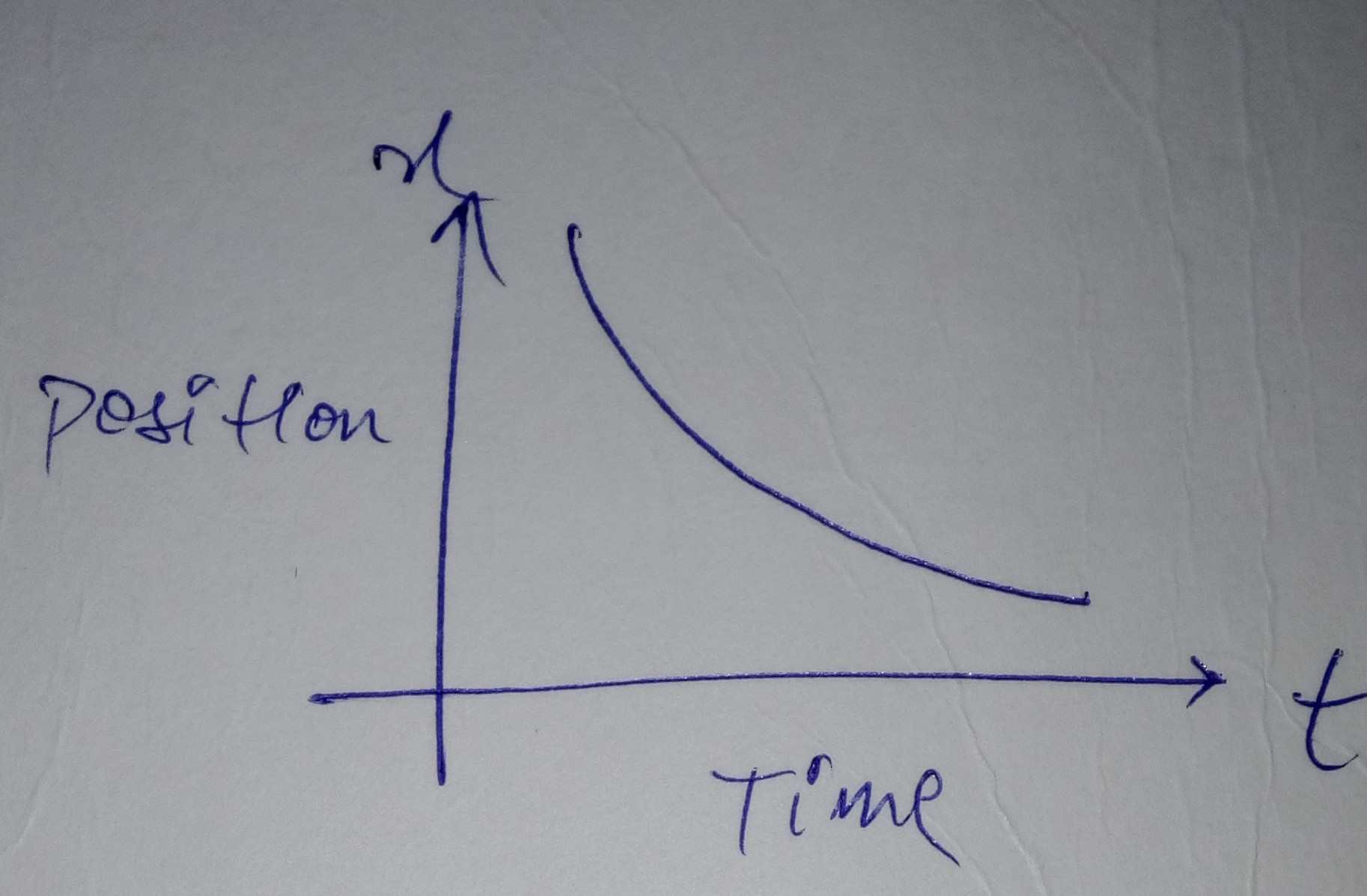
Please explain this graph to me as why acceleration is positive
$\begingroup$
$\endgroup$
3
-
$\begingroup$ There is a rule that tells us the function is convex if its second derivative is greater than zero. $a=\dfrac{d^2x}{dt^2}$ and it is greater than zero, so $x$ is convex. Another explanation: the acceleration is positive, then the velocity $v(t)$ is an ascending function; we could consider an elementary case, when $V=ax$, i.e. $a$ is constant. Then $x\sim Ca^2, C>0$, so the path would represent a part of parabola with "branches up". $\endgroup$– nicaelCommented Apr 13, 2018 at 9:40
-
$\begingroup$ @nicael I think your answer is fine and if I were you I wouldn't have deleted it. If you would like to undelete it I think it would make a useful contribution. This is an elementary question but I still think it's a valid question. We were all beginners at some point. $\endgroup$– John RennieCommented Apr 13, 2018 at 10:41
-
$\begingroup$ @John well, ok! $\endgroup$– nicaelCommented Apr 13, 2018 at 13:38
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
The velocity is the gradient of the line on your position:time graph. If I roughly estimate the gradient by eye I get something like this:
And the acceleration is the gradient of the velocity:time graph
answered Apr 13, 2018 at 8:22
-
3$\begingroup$ Alternatively, the velocity is increasing (becoming less negative) with time, so the acceleration must be positive. $\endgroup$– jimCommented Apr 13, 2018 at 9:46
-
$\begingroup$ Please tell me why velocity is increasing? $\endgroup$ Commented Apr 13, 2018 at 11:19
-
$\begingroup$ @brahamdeepsingh The velocity starts out as a negative number and it becomes less negative. That means the change in the velocity is a positive number i.e. the velocity is increasing. $\endgroup$ Commented Apr 13, 2018 at 11:21