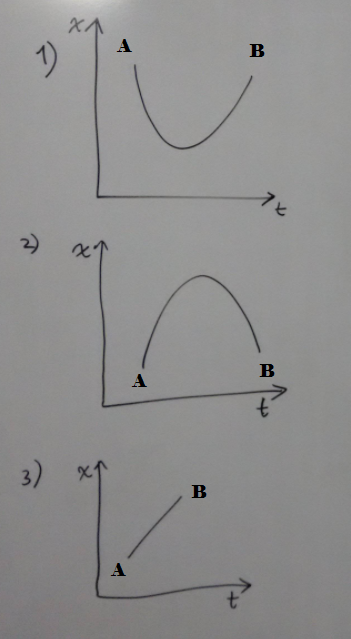
These three graphs are from my textbook. It states that the acceleration in 1) is positive, 2) is negative and 3) is zero and can be told by looking at the slope.
What I understand from the graph is in the picture above. How can I conclude the signs of acceleration from that information in graphs 1 and 2?
The acceleration is the rate of change of velocity (i.e., how fast it's changing in time).
A positive acceleration means increasing values of velocity, for example, as in your picture, that the velocity (slope) goes from negative to positive values. A negative $a$ means decreasing values for $v$.
When the velocity is neither growing, nor getting smaller, its rate of change is zero: $a=0$.
Graphically, when a curve in the $x$ vs. $t$ plot has its concavity pointing up, $a$ is positive; when it's pointing down, $a$ is negative.
In the first graph the velocity is changing from negative to positive with respect to time, i.e. $\frac{dv}{dt} > 0$. Acceleration $a = \frac{dv}{dt}$ and thus a is positive.
In the second graph it's just the opposite.
In the graph 1, body is moving with a constant acceleration, In the graph 2, body is moving with a constant retardation, and, In the graph 3, body is moving with a constant velocity.
On average, acceleration is related to the change in slope over time
$$ a_{\rm ave} = \frac{ {\rm slope}(B) - {\rm slope}(A) }{ {\rm time}(B) - {\rm time}(A) } $$
| Case | Slope at A | Slope at B | Acceleration Sign |
|---|---|---|---|
| 1) | negative | positive | positive |
| 2) | positive | negative | negative |
| 3) | positive | positive | zero |
