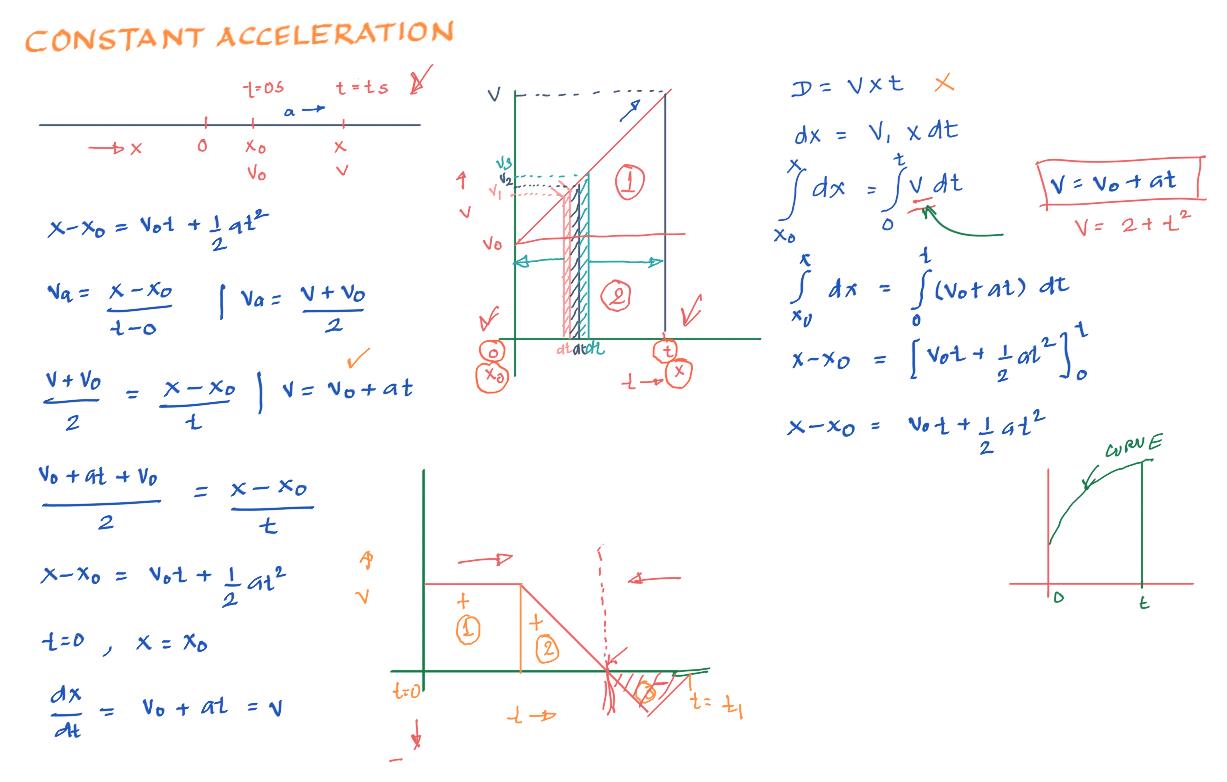
Wondering from the position vs time graph of an object moving with constant acceleration. How could you find the velocity? So the position vs time graph would be a parabola. I am thinking that the instantaneous velocity, $v_y$, may be found as the slope of the tangent to a displacement versus time graph at any point. Wondering if someone could help clarify this for me
$\begingroup$
$\endgroup$
3
-
$\begingroup$ You are correct. Finding the slope of a tangent line is one way of solving for instantaneous velocity at a given time, but if you are drawing the tangent line by hand it may not be totally accurate, but you have the right concept. Unless you can draw perfect tangent lines, a more accurate method would be to solve the derivative of the displacement-time function at the given time, but if you are content with a little bit of error and/or you don't know calculus, I would stick to the first method :) $\endgroup$– RyanCommented Oct 19, 2016 at 0:47
-
$\begingroup$ I understand the question to be, "how can I find the instantaneous velocity from the position vs. time graph of an object undergoing constant acceleration?" I gave the exact correct answer below, but it was downvoted without comment. I can spare the two points, but I hate to see visitors go away thinking that the correct answer is faulty. $\endgroup$– D. EnnisCommented Oct 19, 2016 at 10:05
-
$\begingroup$ bjp409 you arer asking a question for which you already found an answer... $\endgroup$– Žarko TomičićCommented Dec 29, 2017 at 9:30
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
For unidirectional uniform motion,average velocity,average speed,instantaneous velocity and instantaneous speed all are equal.
Things are not so complicated even if we are dealing with accelerated motion.Just find the point at which you want the instantaneous velocity and calculate its slope.it will give you instantaneous velocity.
answered Oct 19, 2016 at 6:56
$\begingroup$
$\endgroup$
Yes, finding velocity that way is correct.
Acceleration is constant $=a$.
Once integrate, velocity is linear
$$ v = v_0 + a\,t $$
Again integrate, position is parabolic
$$ x = x_0+ v_0 \,t + a t^2/2. $$
$\begingroup$
$\endgroup$
The instantaneous velocity at a point in time is equal to the average velocity over any time interval that the point is in the middle of. So if you want the instantaneous velocity at 5 seconds, find the slope of the line between points on the curve at 4 and 6 seconds, or 3 and 7 seconds, etc.
Note: Someone apparently downvoted the above answer without understanding, so I must have been too brief or unclear. I'll explain. The secant line to a continuous curve is defined as the line connecting any two points on the curve. In the interval between those two points for an increasing function, it can be seen that the slope of the tangent to any point changes from a value less than that of the secant line to a value greater than that of the secant line. So there must be a point where the slope of the tangent is the same as that of the secant line (in other words, they are parallel). This is the idea that leads to the derivative of a function in calculus. For a parabolic position vs. time curve, that point is located in the middle of the time interval. The slope of the tangent at that point, and the slope of the secant line that it's in the middle of, are equal to the instantaneous velocity.
$\begingroup$
$\endgroup$
Displacement is a product of velocity and total time of travel.
If you see a velocity time graph (please see the explanation i've written in page below), you’ll see that it is composed of several small rectangles under the curve that are nothing but products of small time intervals (often shown as “dt”) and the velocity at that time. If you sum up the area of these rectangles or integrate (integration is nothing but summation of area), you get the total displacement
You can also watch this video made by me for more clarity-
Position Vs. Time Graph for finding Displacement
