3
$\begingroup$

If you spin a single nucleus containing multiple nucleons fast enough it will fly apart.

Is there a speed limit to a spinning proton or electron assuming it's held at a fixed location with a strong magnetic field?

What speed would either have to attain before breaking up, assuming they can be broken up? What would they break up into?

Improve this question
$\endgroup$
3
  • $\begingroup$ That's a good (homework type) question, just balance the electric charge holding them together against their atomic masses and and you should get a good estimate, just an estimate though. This site is designed to provide hints and ideas, not (no offence meant to you) to solve problems. Think of them as a spinning barbell, with the bar as the charge between them, as a first approximate figure. $\endgroup$
    – user163104
    Commented Aug 13, 2017 at 17:07
  • 1
    $\begingroup$ Looking at the answers, I think that people misunderstand the question asked here. (Free) electrons indeed can have no angular momentum other than spin, since they are point particles. But protons can, since they are no point particles (see en.wikipedia.org/wiki/… ) So you could in principle rotate a proton and see what happens, same as you can do with molecules or nuclei. Don't ask me how to do it experimentally and which angular momenta are necessary to see any noticeable effect though, since strong interaction is... strong. $\endgroup$
    – Photon
    Commented Aug 13, 2017 at 19:45
  • 2
    $\begingroup$ @Photon excited nucleon states are very common in strong interaction physics. en.wikipedia.org/wiki/List_of_baryons look at the table of baryon resonances. Nearly every nucleon resonance that isn't 1/2 is rotating, and many of the 1/2 states are also rotating. $\endgroup$
    – Johnathan Gross
    Commented Aug 13, 2017 at 21:02

2 Answers 2

Reset to default
6
$\begingroup$

You can't spin up an electron. The only way that is possible is if as a string you can put angular momentum on it that way. The energy required to do that would be near Planck scale energy. So I will say this is not possible with the electron FAPP.

For the proton you can spin it up. This is the Regge trajectory that has the angular momentum $J$ as the abscissa and $M^2$as the ordinate. At the bottom here is the $940MeV$ particle, corresponding to the proton. The proton is made of three quarks and they have intrinsic spin $\frac{\hbar}{2}$ that adds up to the same. If I spin this up, we think of these three quarks as being like the masses on a bolos the Argentine gauchos use to rope cattle. The next higher state has a mass of $1680MeV$. This state is not stable and the additional $740MeV$ of rest mass can enter into the production of mesons. We may think of the proton with $udd$ quarks such that one of the $d$ quarks has its "gluon string" break so it becomes coupled to a $d$ anti-quark and the $d$ quark remains with the baryon. the $d,\bar d$ meson carries off this additional mass energy.

For a whole nucleus you have similar physics, though a bit more complicated. There are people who work with rotating nuclei.

enter image description here

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ The text on your figure (v1) is not legible. Could you possibly replace with a legible version? And while you're editing, could you add a link to the source for your figure? Thanks. $\endgroup$
    – rob ♦
    Commented Aug 14, 2017 at 4:17
  • $\begingroup$ 1. What is the first paragraph, in particular "as a string", supposed to mean, and why would the required energy be near the Planck scale? 2. You say that one can spin a proton, but you don't actually answer the question of if/when it will break apart at some sufficiently high angular momentum. $\endgroup$
    – ACuriousMind ♦
    Commented Aug 14, 2017 at 12:19
  • 1
    $\begingroup$ An electron as a string could be spun in much the same way a QCD string or bag can be spun. The energy scale is much larger. As for spinning a proton, I indicated exactly what the energy steps were where it 'breaks apart." $\endgroup$
    – Lawrence B. Crowell
    Commented Aug 14, 2017 at 18:20
1
$\begingroup$

How fast can you spin a proton or electron without breaking it?

Even in the link you give, spin is a quantum number which can increase if the energy input to the nucleus is increased, the individual nuclei going to higher energy bound states ( or quantum mechanically defined bands) in higher angular momentum quantum numbers.

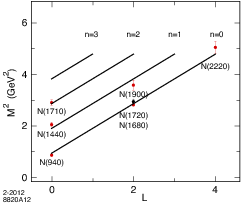
A proton is composed out of quarks. When enough energy is supplied to the proton the quarks settle at higher energy states with higher angular momentum. These are called baryonic resonances and are, as stated in the comments by Jonathan Gross, and can have spins higher than 1/2 of the proton neutron. The energies are of order of Mev, the delta resonance, spin 3/2, is about 200 MeV heavier than the proton and energy has to be supplied in the scattering process higher than that order of magnitude.

The electron is an elementary particle , a point particle , in the very well validated standard model of particle physics. It has an intrinsic spin but it is not a composite , but a point particle. It cannot be "broken up".

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.