You can't spin up an electron. The only way that is possible is if as a string you can put angular momentum on it that way. The energy required to do that would be near Planck scale energy. So I will say this is not possible with the electron FAPP.
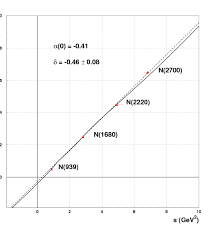
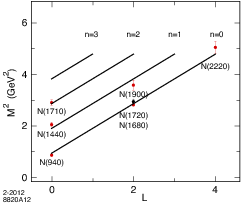
For the proton you can spin it up. This is the Regge trajectory that has the angular momentum $J$ as the abscissa and $M^2$as the ordinate. At the bottom here is the $939MeV$$940MeV$ particle, corresponding to the proton. The proton is made of three quarks and they have intrinsic spin $\frac{\hbar}{2}$ that adds up to the same. If I spin this up, we think of these three quarks as being like the masses on a bolos the Argentine gauchos use to rope cattle. The next higher state has a mass of $1680MeV$. This state is not stable and the additional $741MeV$$740MeV$ of rest mass can enter into the production of mesons. We may think of the proton with $udd$ quarks such that one of the $d$ quarks has its "gluon string" break so it becomes coupled to a $d$ anti-quark and the $d$ quark remains with the baryon. the $d,\bar d$ meson carries off this additional mass energy.
For a whole nucleus you have similar physics, though a bit more complicated. There are people who work with rotating nuclei.