About Torricelli's law derivation: $p_1=p_2$?

Question

When deriving Torricelli's law, pressure at points 'a' and 'b' are said be equal to atmospheric (Figure 1 below).

Torricelli's law became a specific case of the Bernoulli's principle, whose derivation uses the diagram below (Figure 2), and requires the pressure at point 2 be tangential to fluid velocity.

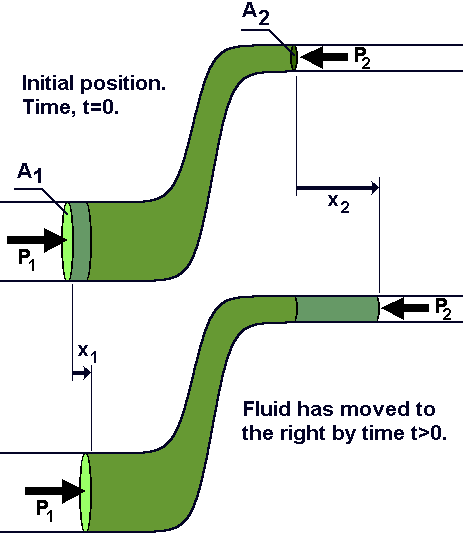
_{(source: 4physics.com)}

As a waterjet is formed in Torricelli's law experiment, atmospheric pressure is tangential only at the start (t=0), and then, at the very end of the waterjet when it's falling (describing a parabola), until it hits the "ground" (a table, the floor, etc.).

Hence, I would say that pressure at point b of Figure 1 cannot be the atmospheric, also because this point is in the start of a streamline (at t>0). But as this law has been discovered in 1643, many years have passed since then and there must be something I don't understand well enough about Pa=Pb. Thanks in advance.

Answer 1

At point B, the air pressure at the surface of the jet is acting normal to the jet. So, since pressure is continuous across the interface (neglecting surface tension), the water pressure just inside the surface must be 1 atm. And, if there is no radial motion of fluid, the pressure must be uniform across the cross section. But, by Pascal's law, pressure acts equally in all directions. So the pressure must be pushing back in the axial direction over the entire cross section with a value equal to 1 atm.