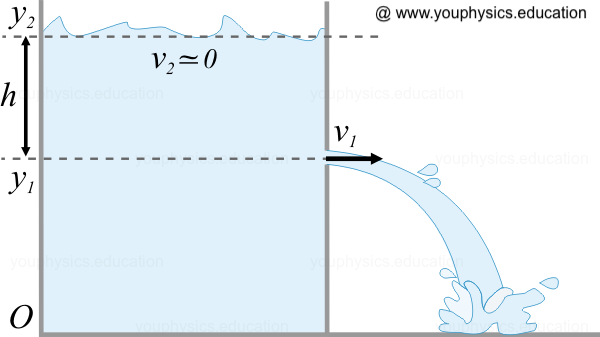
Torricelli's law follows from Bernoulli's principle for an incompressible fluid $\rho = const$
$$ \frac{\rho u_i^2}{2} + \rho g h_i + p_i = const $$
assuming that the area ratio between the container $A_1$ and the hole $A_2$ is large
$$ A_1 \gg A_2. $$
If you consider a streamline starting from a point 1 at the top part of the container, there is the pressure $p_1$, which corresponds to the ambient pressure and a velocity that results from the surface moving downwards $u_1$ as water is pouring out. At the point 2, the hole in the container, the potential energy has been converted to kinetic energy and the pressure corresponds to the ambient pressure of the surrounding fluid:
$$ \frac{\rho u_1^2}{2} + \rho g h_1 + p_1 = \frac{\rho u_2^2}{2} + \rho g h_2 + p_2 $$
$$ \frac{\rho u_1^2}{2} + \rho g \underbrace{(h_1 - h_2)}_{h} + p_1 = \frac{\rho u_2^2}{2} + p_2 $$
Assuming the change in pressure in the surrounding fluid can be neglected (of course if the outside was water as well then the velocity would clearly be zero as the ambient pressure would change with height as well) $p_1$ and $p_2$ can be assumed equal $p_1 \approx p_2$.
Furthermore we can consider the 1D continuity equation
$$ \dot{m_1} = \rho \dot{V_1} = \rho A_1 u_1 = \dot{m_2} = \rho \dot{V_2} = \rho A_2 u_2, $$
$$ A_1 u_1 = A_2 u_2. $$
Assuming that $A_1$ is significantly larger than $A_2$, $A_1 \gg A_2$ we can neglect the velocity at the top of the container $u_1 \approx 0$ and thus find Torricelli's law
$$ u_2 \approx \sqrt{2gh}. $$
1) As you can see there is no pressure term as you follow a particle from the top of the surface to the exit and on both sides there is assumed the same ambient pressure. If it would be a closed pressurised container you would end up with $u_2 \approx \sqrt{2gh + 2 \frac{(p_1 - p_2)}{\rho} }$ and there would be indeed a contribution from the higher pressure inside the tank.
2) Clearly the derivation of this idealised law is based on the assumption of a small hole but as long as one can still neglect the velocity at the top of the container $u_1 \approx 0$ the outcome would still be the same and not depend on the area. This is a result of mass continuity.
3) As already mentioned we have to consider the ambient pressure for both sides as it reflects an external "force". Consider that at the top of the container there would be a piston pushing the liquid down, so to say increasing the ambient pressure, the liquid would flow out faster, wouldn't it? If also the surrounding fluid was water there would be also a change in pressure with the height of the surrounding fluid and the ambient pressure at point 2 would be equal to $p_2 = p_1 + \rho g (h_1 - h_2) = p_1 + \rho g h$ and there would be no directed macroscopic motion through the hole.