If the potential drops across a resistor (=$V_d$) then shouldn't the potential difference be the $\epsilon-V_d$?

Question

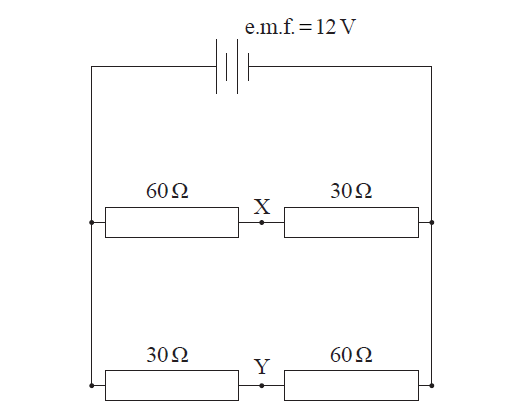
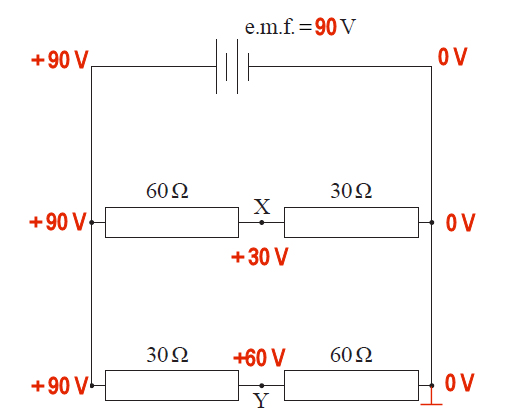
Consider the following circuit:

Suppose a current $I$ travels in both the branches, then as the current $I$ passes through the $60$ ohm resistor, there will be a drop in the potential of $60I$. Similarly, there will be a drop of $30I$ across the $30$ ohm resistor in the second branch. By drop in potential difference, I understand that we have lost that much quantity of p.d. and therefore, I believe that the potential at point X should be $12-60I$ and the potential at Y should be $12-30I$. However, in the several problems that I've tried, this is not the case. Please explain, why?

Please note that this is not a Homework Problem, but a specific example which I am using to understand a concept.

Answer 1

It is sometimes easier to visualise what is happening by using the idea of potential.
To do this make one point in the circuit 0 V.
This is a totally arbitrary choice.
It is the bottom right hand corner of your circuit.
Note to make the sums easier I have change the emf of the battery to 90 V so 2 A flows through the battery and 1 A through each of the resistance branches.

The potential difference across the top 30Ω resistor is $1 \times 30 = 30$ volts so the potential of node $X$ is $+30$ volts.
For the bottom 60Ω resistor the potential difference is is $1 \times 60 = 60$ volts so the potential of node $Y$ is $+60$ volts.

So the potential difference across $YX$ is $60 - 30 = 30$ with node $Y$ at the higher potential.

If a pair of resistors in one of the branches was interchanged the potential difference across $XY$ would be zero and this is the configuration for a balanced Wheatstone bridge.

Answer 2

I believe that the potential at point X should be 12−60I and the potential at Y should be 12−30I. However, in the several problems that I've tried, this is not the case. Please explain, why?

A proper way to write this is (in terms of the node voltages and branch currents) is

$$V_X = 12V - I_X \cdot 60 \Omega$$

$$V_Y = 12V - I_Y \cdot 30 \Omega$$

Where $I_X$ denotes the current through the top-most pair of resistors and $I_Y$ denotes the current through the bottom-most pair of resistors.

For the particular resistor values given, it is the case that $I_X = I_Y$:

$$I_X = \frac{12V}{60 \Omega + 30 \Omega} = I_Y$$

and so your equations will give the correct result. However, your equations will not give the correct result if, for example, the bottom-right resistor value were different since that would change $I_Y$ without changing $I_X$.

Without any other information about the other problems you've tried, I can only guess that your approach failed because the two branch currents are, in general, not equal.