0
$\begingroup$

Note that this isn't a homework problem, just a question the concept.

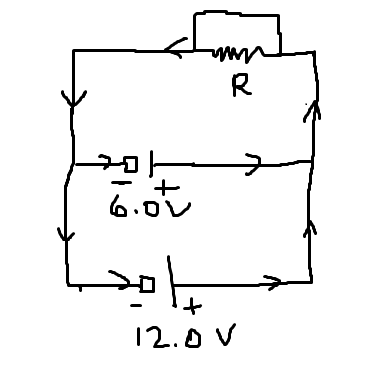
enter image description here

Let's take this quick circuit I drew up just now in MS paint. There are three different currents, one for the top, middle, and bottom branches. The bottom two branches have a voltage source on each, and there's a resistor in the top branch. So we have some set values for the current in each branch, found by using Kirchoff's rules.

Now I add an extra bit of wire at the top, around the resistor:

enter image description here

Is this what a short circuit is? What happens to the current at the top? Does it go completely around the resistor and through the added wire? Or does some of it still go through the resistor? Certainly something would have to change in order to find the currents again, using Kirchoff's rules.

People often say that current takes the path of least resistance, but it feels like that's not completely true.

Improve this question
$\endgroup$
2
  • $\begingroup$ "So we have some set values for the current in each branch, found by using Kirchoff's rules." - that's not true in this case. In the circuit you've drawn, KVL yields $12V=6V$ so the circuit does not have a solution. Add a resistance in series with one of the voltage sources and the circuit can be solved. As you reduce this resistance to zero, you'll find the current 'round the loop formed by the voltage sources goes to infinity. $\endgroup$
    – Alfred Centauri
    Commented Dec 10, 2016 at 2:17
  • $\begingroup$ Ah, I see. To be fair I just based this off something I remembered on a test but clearly the rest was important. What about if there was a resistance after the 12V source? What would happen with the current up there? $\endgroup$
    – hhh
    Commented Dec 10, 2016 at 2:50

1 Answer 1

Reset to default
1
$\begingroup$

I don't see why you need two batteries to ask your question. To work with a minimal example where you're question makes sense I suggest removing the 12 V battery from the circuit.

If we do, then you first diagram becomes a 6 V battery and a resistor. If we say $R=1 \Omega$ for concreteness, the top circuit has a current of 6 Amps.

In your second figure, again disconnecting the 12 V battery, there will be no current through R. It is indeed a short circuit in the sense that you have essentially connected the positive end of the battery to the negative end.

There's two quick calculations I might suggest trying to get some intuition.

The first is to take your second figure, disconnect the 12 V battery, and then put a resistor $R_2$ on the top path (the one that goes around R). Then compute the current through $R$. You will find that as you make $R_2$ smaller and smaller, the current through $R$ gets smaller and smaller as well (and eventually goes to zero).

The second is to compute the current through $R_2$. As $R_2$ gets smaller you'll find the current getting bigger and bigger, tending to infinity. That basically shows if you short circuit an ideal battery as you've drawn, you'll get infinite current. A real battery has a finite resistance (you can model that by putting a resistor r in series with the 6V battery). That will make the current finite. (Although if you try it in a lab your battery might explode).

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ In the real world, a 10ft long 20ga wire has a resistance of roughly 0.1 ohm. Very very small, but not zero. You can use that in a parallel resistance equation to see how the current splits between the "short" and the resistor. $\endgroup$
    – Cort Ammon
    Commented Mar 7, 2020 at 6:01

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.