1
$\begingroup$

Sorry if my grammar is a bit off. But I have few questions about space and its expansion.

If space expands faster and faster all the time, will there be a time when we cannot see further than "hubble sphere"?

Am I correct by thinking that we cannot ever reach the edge of the current hubble sphere due to not being able to go faster than a speed of light and because the edge will be expanded further away?

Improve this question
$\endgroup$
2

1 Answer 1

Reset to default
3
$\begingroup$

No, we will always be able to see farther than the Hubble sphere (in theory).

This spacetime diagram — taken from Pulsar's rendering of Davis & Lineweaver (2003)'s Figure 1, in this excellent answer — can help visualize it:

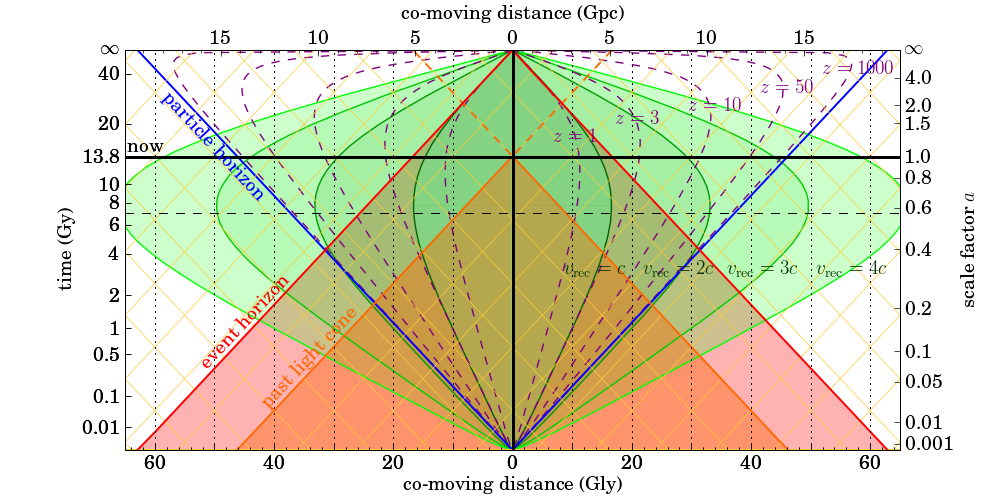
Spacetime

Coordinates

In this figure, time increases upward, we're the vertical line in the middle, Big Bang is the bottom line, and our current time is the black horizontal line. The $x$ axis shows distance from us in comoving coordinates, i.e. the coordinates that expand with the Universe, and in which galaxies lie approximately still. By definition, today comoving coordinates coincide with physical coordinates (i.e. the "real" distance you would measure if you stopped time and laid out rulers), but for instance 8 billion years (Gyr) ago (indicated on the left $y$ axis), when the scale factor $a$ was $0.5$ (indicated on the right $y$ axis), the distance between two given galaxies in physical coordinates would be half that in comoving coordinates.

Particle horizon

The farthest distance to which we can see is called the particle horizon, and is drawn in blue. It will always increase, since light from farther and farther away eventually will reach us (although light from these distances will be increasingly more redshifted and eventually will be unobservable in practise).

Hubble sphere

In contrast, the Hubble sphere — i.e. the distance at which space expands at the speed of light — doesn't increase much more than today in physical coordinates. In comoving coordinates, the Hubble sphere reached a maximum roughly 5 billion years ago, and is now decreasing (the innermost green solid line labeled $v_\mathrm{rec}=c$). In other words, galaxies closer and closer to us are receding at $v=c$, but not until they are farther away from us physically.

Can we reach the Hubble sphere?

As for your second question, the answer depends a bit on what you mean: By definition, the Hubble sphere is a certain distance from you, so taking your question literally, it's impossible to go to the Hubble sphere, since it will always be some distance from you. But I assume that you mean "Is it possible for us the send a space probe from Earth, out to Earth's Hubble sphere". The answer to this is "Yes". Although special relativity prohibits the space probe from going faster than $c$ locally, as seen from Earth the expansion of space "helps" the probe to go faster and faster, eventually surpassing $c$ (just like it helps galaxies going faster than $c$).

In the figure, the orange dashed line is our future light cone; events outside this region can never be affected by us. You see it incercepts the Hubble sphere approximately at $t=25\,\mathrm{Gyr}$, or $a=2$. This means that, if a space probe departs today and travels at almost the speed of light, it will reach our Hubble sphere in 10 billion years or so, at which time the Universe has doubled its size (in all three directions). A space probe traveling at more realistic velocities will reach the Hubble sphere later; the exact time can be seen from the figure using a wordline with a slope that is steeper than the 45º that light rays have.

Improve this answer
$\endgroup$
10
  • $\begingroup$ Just to be clear, when you say the particle horizon is "The farthest distance to which we can see", are you assuming that "we" are located at a point on the horizon rather than at the co-moving position of 0 on the horizontal axis? If instead we are located at co-moving position 0, it seems the line marked "past light cone" would be the furthest we can see now, and the line marked "event horizon" would show the furthest we will ever be able to see (our past light cone in the limit as time approaches infinity). $\endgroup$
    – Hypnosifl
    Commented Nov 24, 2021 at 4:18
  • $\begingroup$ @Hypnosifl The lines you mention are symmetric for exactly this reason: You are situated at the crossing point of the two black lines, {here,now}. Your "current" space — or just space — is everything along the horizontal line. You can see out to the point where the black and the blue lines cross, i.e. out to the particle horizon. The part of the black line inside this crossing is called the observable Universe. The same was true at earlier times, and will be true in the future; the observable Universe is always bounded by the particle horizon. [cont'd below] $\endgroup$
    – pela
    Commented Nov 24, 2021 at 12:22
  • $\begingroup$ [cont'd from above] However, you don't see everything in the observable Universe as it looks now. The time that you measure in a galaxy at a given distance from you, is given by your past cone. For instance, let's say that, today you observe a galaxy that is currently at a comoving distance (and hence a "real", physical distance, since physical distances and comoving distances are defined to be equal to each other today). In comoving coordinates, this galaxy moves through spacetime along a vertical line, which is black dotted in the diagram [cont'd below] $\endgroup$
    – pela
    Commented Nov 24, 2021 at 12:41
  • $\begingroup$ [cont'd from above] The time in this galaxy — i.e. the age of the Universe when it emitted the light you see today — is found by following your past light cone down to the point where it crossed the galaxy's dotted worldline. This crossing is seen from the $y$ axis to be at ~2.3 Gyr (also, the secondary $y$ axis shows you that the expansion parameter at that time was $a=0.26$, so you'll see the galaxy redshifted by an amount $z=1/a-1=2.8$). Using the particle horizon as an example, you see it 46 Glyr away, but following your past light cone shows you that you see it at a time $t=0$. $\endgroup$
    – pela
    Commented Nov 24, 2021 at 12:41
  • $\begingroup$ I suppose it doesn't matter whether we use the current comoving distance to the particle horizon or the maximum past extent of our past light cone to define the size of the "observable universe", since both values are identical (as is clear from the conformal diagram). I'd think it would be conceptually clearer to define the size of the "observable universe" in terms of the latter rather than the former, since what we can "observe" is usually defined in terms of our past light cone, but maybe there are some other conceptual/pedagogical reasons for preferring the particle horizon definition. $\endgroup$
    – Hypnosifl
    Commented Nov 24, 2021 at 21:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.