11
$\begingroup$

I was recently shown a pretty cool video about common cosmological misconceptions. It got me reviewing the different between event horizon (current distance within which we will see/interact), particle horizon (current distance within which we have seen/interacted, and the Hubble Sphere (distance at which things are currently receding at the speed of light).

I've found the following figure extremely helpful: enter image description here

But I'm very confused about the event horizon currently lying outside of the Hubble Sphere. If the universe is accelerating in its expansion, then it seems like everything outside of the Hubble Sphere at this moment - will never be in causal contact, never be within our light-cone, and thus be outside of our event horizon. Is this figure correct?

Improve this question
$\endgroup$
6
  • $\begingroup$ If the expansion is slower than the speed of light (and it is) then stuff outside the Hubble Sphere could still make it to within our light cone. $\endgroup$
    – Jiminion
    Commented Jun 19, 2014 at 2:35
  • 1
    $\begingroup$ Related : physics.stackexchange.com/questions/60519/… $\endgroup$
    – Trimok
    Commented Jun 19, 2014 at 8:44
  • 1
    $\begingroup$ Here's the brief explanation. A comoving observer at the Hubble radius would find that there is $3\times10^8$m more of proper distance between us after 1 second. A beam of light travelling away from us at the Hubble radius would find that after 1 second there is $6\times10^8$m more of proper distance between us. A beam travelling towards us would find that there is no change in proper distance. In the latter case, however, there is a decrease in the comoving distance between us. Also, the proper hubble radius increases with time. So now, the light beam starts to close the proper gap $\endgroup$
    – Jim
    Commented Jul 10, 2014 at 19:32
  • $\begingroup$ @Jiminion What do you mean by saying "the expansion is slower thanthe speed of light"? $\endgroup$
    – Thriveth
    Commented Sep 1, 2016 at 16:05
  • $\begingroup$ @Thriveth Hubble expansion is about 70 km/sec per Mpc. $\endgroup$
    – Jiminion
    Commented Sep 1, 2016 at 16:52

3 Answers 3

Reset to default
7
$\begingroup$

As Chris White points out, this is a subtle issue, so I'm eager to see some more answers - perhaps someone can some up with a good car analogy ;)

In the meantime, here's my best shot at an explanation:

First, accept that the existence of a preferred spatial slicing does not make FLRW spacetime into Minkowski spacetime: Proper distance at constant cosmological time is no substitute for special relativistic proper distance, and all caveats of general relativity still apply.

Now, consider comoving coordinates and pick any two points at rest as emitter and (eventual) absorber. No matter the initial proper distance or recession velocity, a photon will move steadily from emitter towards absorber, decreasing the comoving distance it still needs to travel. It will not stop or freeze at any particular distance, and this is even true for photons that get emitted from beyond the event horizon - they'll just take a longer-than-infinite amount of time to reach their destination...

The event horizon is basically the past light cone at $t=\infty$, made up from null geodesics and has physical significance. In contrast, the Hubble sphere is largely arbitrary: It's where a particular coordinate velocity - the recession velocity - reaches $c$. However, the speed of light only limits relative velocities, which need to be evaluated at the same event or via parallel transport. Going by Pulsar's figure, light that reaches us from the Hubble sphere right now has $1\lt z\lt3$, so as far as the photon is concerned, the relative velocity of emitter and absorber was about $(0.7\pm0.1)c$ - nothing to sneeze at, but near enough is not good enough.

Improve this answer
$\endgroup$
5
  • 2
    $\begingroup$ Still, one can ask why nothing special happens at the Hubble sphere. In physical coordinates, it seems natural to say that if E and A are moving apart at $c$, a photon cannot compensate. And in comoving coordinates, we all agree that there is a finite distance at which the photon's travel time from E to A is infinite - why is that distance strictly greater than the distance to the Hubble sphere? I'm not saying anything you've written is wrong, but this is a very subtle thing to explain satisfactorily. $\endgroup$
    – user10851
    Commented Jun 19, 2014 at 7:44
  • $\begingroup$ @ChrisWhite: see edit - it might be a bit more clear now $\endgroup$
    – Christoph
    Commented Jun 19, 2014 at 15:59
  • $\begingroup$ next item on the list: figure out if and why any sphere of constant $v_\text{rec}\gt c$ will intersect the event horizon $\endgroup$
    – Christoph
    Commented Jun 19, 2014 at 16:09
  • $\begingroup$ Thanks Christoph, really insightful answer (and edit)! but there's still definitely pieces missing :) The crux of my confusion is that a photon emitted at the Hubble Sphere now still sees a $v = c$ recession velocity... but I think it's starting to come together for me $\endgroup$
    – DilithiumMatrix
    Commented Jun 19, 2014 at 16:20
  • $\begingroup$ damn - I forgot that in Pulsar's figure, redshifts need to be projected onto the light rays to associate them with events - the actual redshift is not $3$, but somewhere between $1\dots3$ $\endgroup$
    – Christoph
    Commented Jun 19, 2014 at 18:00
5
$\begingroup$

The reference is Pulsar's answer, see detailed explanations and graphics.

@Christoph 's answer is correct, so it is just an other way to express the answer.

First, there is a notion of instantaneous proper distance (between us and some galaxy), where we may imagine that the expansion is stopped at time $t$, and that we measure the distance between us and the galaxy. This is a pseudo-distance, which is not really and practically measurable.

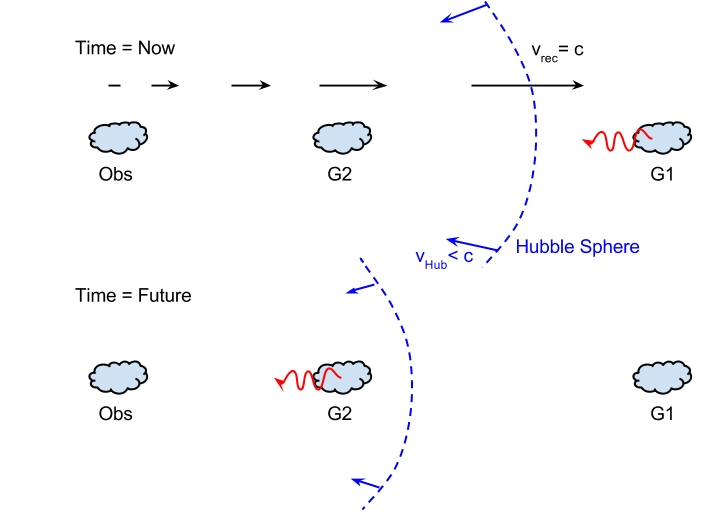
The Hubble distance is a proper distance corresponding to a recession velocity of $c$ at some time $t$. It depends only on characterics ($a(t)$, etc...) at the time $t$.

The difference between the cosmic event horizon proper distance (and also for the past light-cone proper distance) , compared to the Hubble distance (see in the reference the expressions of $D_\text{H}(t_{ob}),D_\text{lc}(t_\text{em},t_\text{ob}) ,D_\text{eh}(t_0$) for details), is that, for the cosmic event horizon proper distance and the past light cone distance, there is a physical signal (a photon). So there is an integration on different $a(t)$, this corresponds to the fact, that, while the photon itself has always a speed of $c$, in a local frame, the real distance between, for instance, $2$ intermediate galaxies on his travel, is increasing, due to the expansion of the universe. So we have to take in account all the values of $a(t)$ from the time where the photon is emitted until the time where the photon is observed.

The other fact is that it is exact that the Hubble distance lies completely inside the event horizon. The relation between the $2$ depends on the expression of the Hubble parameter $H(a(t))$, between $t$ and $+\infty$

Improve this answer
$\endgroup$
5
$\begingroup$

Here's my attempt at answering the question (read: thinking out loud).

In the past the universe was decelerating in its expansion.
Because of that, the Hubble-Sphere was actually expanding; i.e. you have to look father and farther away to find an object receding at the speed of light. Thus, if an object just a little ways outside the Hubble-sphere emits a photon, if you wait a while it will be within our Hubble-sphere, and we will eventually receive the photon. No problem.
But in a way, the Hubble sphere and photon were actually working together. What I mean is, the photon is traveling towards us, and the Hubble sphere is moving away from us -- bringing that struggling photon within its grasp.

Now, the universe is accelerating in its expansion.
That means the Hubble sphere is contracting. Something which was receding at sub-luminal velocities, can --- in the future --- recede superluminally. So a photon emitted just outside the Hubble sphere, at a galaxy G1, is actually fighting against the contracting of the Hubble sphere. What matters is how fast the Hubble sphere is contracting, versus how fast the photon is closing the distance to us (shoutout to @christoph's awesome post!).
Imagine a galaxy (G2) halfway between us and where the photon was emitted (G1, just outside of our Hubble sphere). G1 is within the Hubble sphere of G2, so it's easy to believe that the photon will reach G2 in finite time. What matters to us, is whether G2 will be within our Hubble sphere when the photon reaches it! Even though the Hubble sphere is contracting, the photon might be able to close the distance faster.
The Event Horizon is the distance from which a photon (emitted now) will never quite be able to enter our Hubble Sphere.

Maybe you're still thinking, "But if stuff at the Hubble Sphere is receding at the speed of light... how could a photon ever catch-up?" (At least I was). I think the kicker is that the Hubble sphere is not universally receding at the speed of light, but only apparently - relative to the observer at the full distance away.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.