How does the voltage across an inductor and a capacitor vary in series LCR circuit about resonance?

Question

I am aware that at resonance, the voltage across the inductor and the capacitor are equal in magnitude and opposite in phase. However, I want to know how the voltage across $L$ and $C$ vary if I vary the frequency on either side of the resonant frequency and if any relationship exists between the two (e.g. whether one decreases while the other increases with frequency below the resonant frequency and swaps their behaviour above the resonant frequenc).

Update: For and LCR circuit, we can write the following expressions for the voltages across the capacitor $V_c$ and the inductor $V_L$:

$V_c=\frac{-j}{\omega C}\frac{V}{R+j(\omega L - \frac{1}{\omega C})}$

and

$V_L=V\frac{ j\omega L }{R+j(\omega L - \frac{1}{\omega C})}$

($V$ is the rms volatge applied to the circuit and $j=\sqrt{-1}$)

And, the magnitudes of of $V_c$ and $V_L$ are:

$|{V_c}|=\frac{V}{\omega C\sqrt{R^2+(\omega L - \frac{1}{\omega C})^2}}$

and

$|V_L|=\frac{V \omega L}{\sqrt{R^2+(\omega L - \frac{1}{\omega C})^2}}$

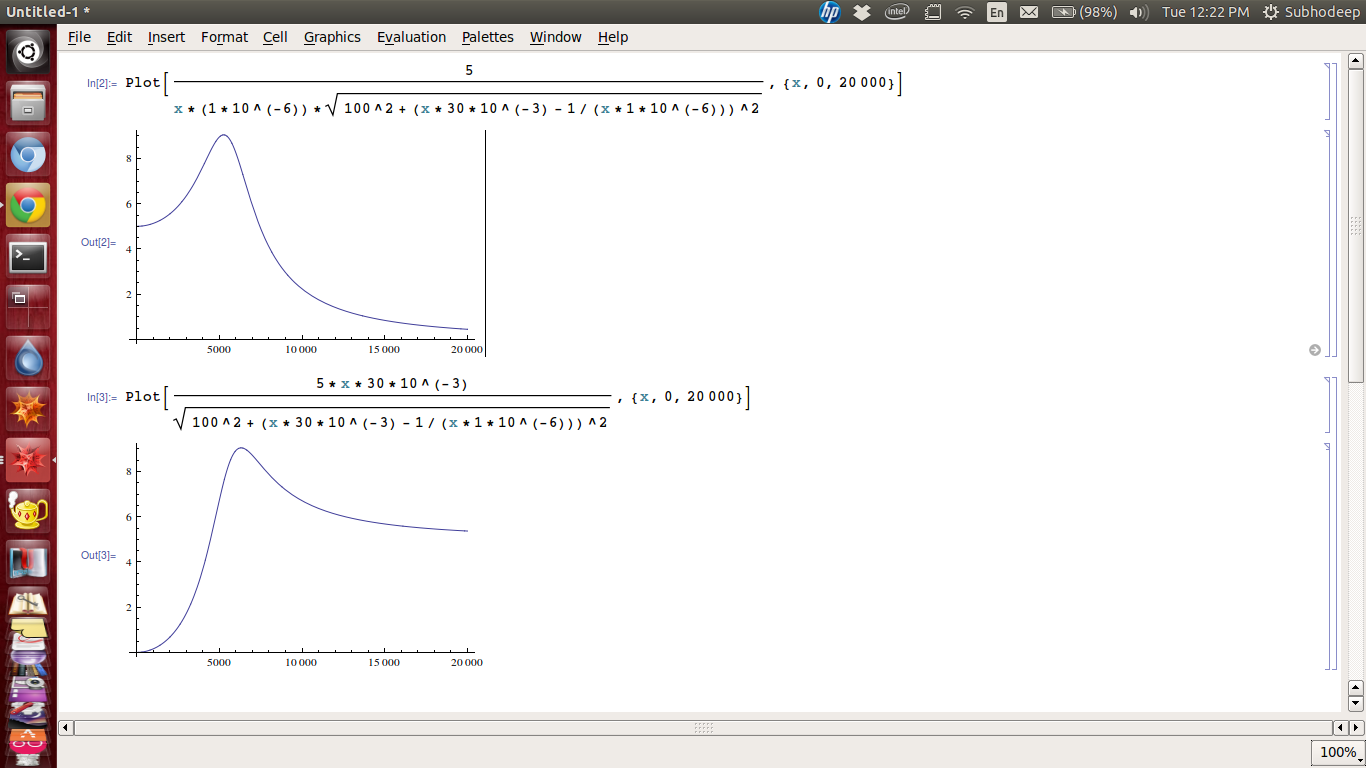
And I plotted $|V_c|$ and $|V_L|$ as functions of $\omega$ using Mathematica and here are the results:

The first plot shows the variation of $|V_c|$ with $\omega$ and the second plot shows the variation of $|V_L|$ with $\omega$. The voltages are along the $Y axis$ and $\omega$ is along the $X axis$.

(I used some standard values: $V=5\,\text{V}$, $R=100\,\Omega$, $C=1\,\mu\text{F}$ and $L=30\,\text{mH}$.)

Answer 1

note: I accidentally thought OP was asking about a series $LC$, not a series $LCR$. Including the $R$ changes the results here by making the infinities turn into large finite values.

Suppose you hook your series $LC$ circuit up to a voltage source with frequency dependent phasor $\tilde{V}_s(\omega)$.

Intuition

First let's guess what happens. At low frequency the inductor looks like a short circuit and the capacitor looks like an open, so the voltage across the inductor should be near zero and the voltage across the capacitor should be roughly $V_s$. At high frequency the inductor looks like an open and the capacitor looks like a short (opposite of low frequency case) so the voltage across the inductor should be roughly $V_s$ and the voltage across the capacitor should be roughly zero.

Near the resonance, the impedances of the inductor and capacitor cancel and the total impedance of the series circuit is very small. Therefore, near the resonance the total current $I$ through the circuit gets very large. The voltage across the inductor is given by $V_L = I_L \times Z_L$ where $I$ is the current through the inductor and $Z_L$ is the impedance of the inductor. Since we have a series circuit, $I_L = I$, so near the resonance where $I$ gets very large we expect $V_L$ to also get very large. The same reasoning applies to the capacitor.

Math

From the voltage divider equation you know that the voltage across the inductor is

$$V_L(\omega) = \tilde{V}_s(\omega) \frac{Z_L(\omega)}{Z_L(\omega) + Z_C(\omega)}$$

where $Z_L$ is the impedance of the inductor and $Z_C$ is the impedance of the capacitor. Putting in the usual impedance for capacitor and inductor gives

$$\tilde{V}_L(\omega) / \tilde{V}_s(\omega) = \frac{-\omega^2 / \omega_0^2}{1 - \omega^2 / \omega_0^2} $$

where $\omega_0 \equiv 1/\sqrt{LC}$ is the resonance frequency.

• As $\omega \rightarrow 0$, $\tilde{V}_L \rightarrow 0$.
• As $\omega \rightarrow \omega_0^-$ ($\omega$ approaches $\omega_0$ from the lower side), $\tilde{V}_L \rightarrow -\infty$.
• As $\omega \rightarrow \omega_0^+$, $\tilde{V_L} \rightarrow \infty$.
• As $\omega \rightarrow \infty$, $\tilde{V_L} \rightarrow \tilde{V}_s$.

By the same reasoning you get

$$\tilde{V}_C(\omega) / \tilde{V}_s(\omega) = \frac{1}{1 - \omega^2 / \omega_0^2} \, .$$

Here,

• As $\omega \rightarrow 0$, $\tilde{V}_C \rightarrow \tilde{V}_s$.
• As $\omega \rightarrow \omega_0^-$, $\tilde{V}_C \rightarrow \infty$.
• As $\omega \rightarrow \omega_0^+$, $\tilde{V}_C \rightarrow -\infty$.
• As $\omega \rightarrow \infty$, $\tilde{V}_C \rightarrow 0$.

Comparing the results for the capacitor and inductor you can see that their roles are exactly switched in all cases.

Answer 2

Say your series RLC circuit is excited by a constant current source producing current $I$ at angular frequency $\omega$. Since all 4 elements (R, L, C, and source) are in series, the current through any one of them is just the source current.

Then for each element, $V_n=IZ_n$.

For an inductor, the impedance Z is given by $Z=i\omega{}L$.

For a capacitor, the impedance is $Z=\dfrac{1}{i\omega{}C}$.

For a resistor, the impedance is just $Z=R$.

Answer 3

If you take the ratio$| v(l)|/|v(c)|$,you will get it is proportional to $\omega^2\cdot l\cdot c$, while $l\cdot c$ is inversely proportional to square of $\omega_0$ (resonant frequency); so, as long as $\omega$ is less than $\omega_0$, $|v(l)|<|v(c)|$ while, if $\omega$ is greater than $\omega_0$, the $|v(l)|>|v(c)|$.