Difference between stable manifold and basin of attraction?

Question

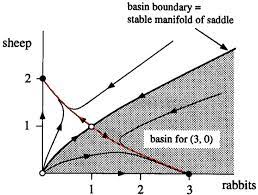
In 'Nonlinear Dynamics and Chaos' by S. Strogatz, a distinction is made between a stable manifold and basin of attraction of a fixed point in phase space:

Here, the stable manifold of a saddle point is a line, and the basin of attraction of a stable node is a plane. However, the definitions of the two terms are the same, namely:

The set of initial conditions $\bf x_0$ such that $\bf{x} \rightarrow \bf{x^{*}}$ as $t\rightarrow\infty$ for a fixed point $\bf{x^{*}}$.

Why is a distinction being made between the two terms?

Answer 1

To some extent it's indeed a convention/definition:

• intuitively, if a set has a region of typical initial conditions leading to it (say, a neighborhood, a finite phase space volume, etc.), and this region is by definition its "basin of attraction" and this attracting set is called an "attractor";
• whereas invariant sets (stable or not) can be calculated for any point, including non-attractive ones: and when a non-attracting set has a stable set associated with it and this stable set is a manifold, then we don't call this manifold its basin of attraction — since it's no attractor — but its stable manifold.

That's the basic gist of it, though of course there's more to it than that. For instance, you'll still distinguish the basin of attraction from the (stable) manifolds of a point attractor, since these manifolds are related to (to be more exact, they coincide locally with) the stable eigenvectors of the linearized system on this point.

Answer 2

Maybe the most straightforward distinction is:

• If the set in question is a true manifold, it’s a stable manifold. By true manifold, I mean that it has a dimension that is not equal to that of its embedding space.
• Otherwise it’s the basin of attraction of an attractor. This basin may also be or contain stable manifold(s), depending on the situation and exact definition.

The existence of a true (stable) manifold excludes the existence of an attractor since arbitrarily close to the attractor, you can find points on the manifold, next to which have to be points that do not converge to the attractor (otherwise the manifold would not be a true one).

While the above may present the two terms as a dichotomy, in the broader scope of dynamical systems, they are not. On the one hand, attractors also comprises things like limit cycles and chaotic attractors. On the other hand, stable manifolds also exist in Hamiltonian systems, which cannot have attractors at all.