Poincare-Bendixson Theorem Under Time Reversal

Question

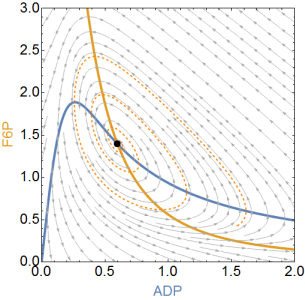
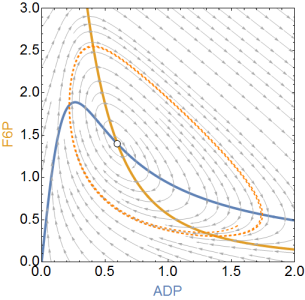
Strogatz's textbook "Nonlinear Dynamics and Chaos", Chapter 7 presents the Poincare-Bendixson theorem, which gives conditions under which one can conclude the existence of a closed orbit within some compact subset $R$ of the phase plane.

As presented in Strogatz, one of the hypotheses of the theorem is the existence of a trajectory $C$ which remains always within $R$ (the Wikipedia presentation makes this less obvious, but is equivalent). Strogatz thus presents a trick to find such a curve: we construct $R$ to be an annular region upon whose boundaries the flow points everywhere inward. Such a region must obviously contain a trajectory with the desired properties.

This, however, seems to assume that the limit cycle is stable (if it were unstable the trajectories would point everywhere outward, at best). I was wondering whether we can adapt the theorem to find unstable limit cycles as well, by considering the time reversed system. Essentially: does the existence of a stable limit cycle in a system imply the existence of an unstable limit cycle in its time-reversed counterpart?

Answer 1

In short: yes! :) I have done that before successfully.

Why does it work?

The autonomous dynamical system is defined as: $\dot{\bf{x}}=\bf{f}(\bf{x})$, with $\bf{x}$ $=(x,y)$ and $\bf{f}$ $=(f_1,f_2)$.

So the flow in phase space is $f_1(\bf{x})$ in "x"-direction and $f_2(\bf{x})$ in "y"-direction.

Reversing time ($t \rightarrow -t$) in an autonomous first order ODE gives:

$-dx/dt=f(x)$

$\Leftrightarrow dx/dt=-f(x)$

This will flip the phase flow direction into its opposite.

So in the simplest case of an attracting fixed point, it will repel trajectories under time reversal.

In the case of a limit cycle, let's use the view of Floquet theory and separate the components of the phase flow into periodic motion on the limit cycle with period T $x(t)=x(t+T)$ (Goldstone mode) and its othogonal deviations that point away from it $\Delta x(t)=x(t)-x_0$ ($x_0$ is a reference point on the limit cycle). In the case of an attracting limit cycle, $\Delta x(t)$ will decrease in every iteration. When we reverse time, the phase flow field flips so trajectories are pushed away from the limit cycle.

Here are the two versions of the phase plane dynamics of the Selkov model (if you read chapter 7 in Strogatz book, you are familiar with it). In the left picture exists an unstable limit cycle and in its center we find a stable fixed point - as expectable. In the right version, time is reversed and we find the stable limit cycle, whose existence we can prove by construction of a "trapping region" for Poincare-Bendixson, as Strogatz did in Example 7.3.2.

Blue and yellow lines are the nullclines(which do not change their position under time reversal). The orange dashed line shows a trajectory starting from the same point in both versions to illustrate phase space flow.