1
$\begingroup$

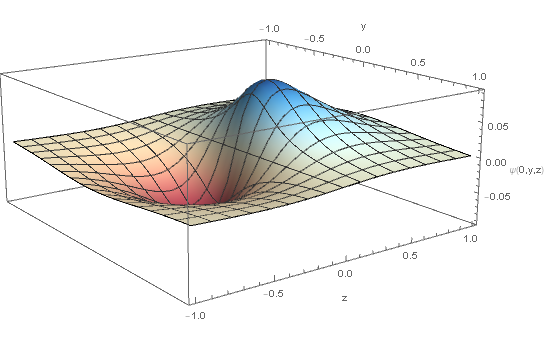
This question arose when I was told that there were positive and negative lobes in an orbital. I wanted to know on what basis this was proposed and hence I searched it on web and found out that it resembled the phases of the wave. But I always thought of orbital as a 2-d racing circuit for the electron, in which the electron would race around while bobbing up and down. Hence I wanted to know whether the p-orbital is the wave itself or is it like a racing circuit?

Here is my attempt to explain it visually! (Great graphics? Thank you!)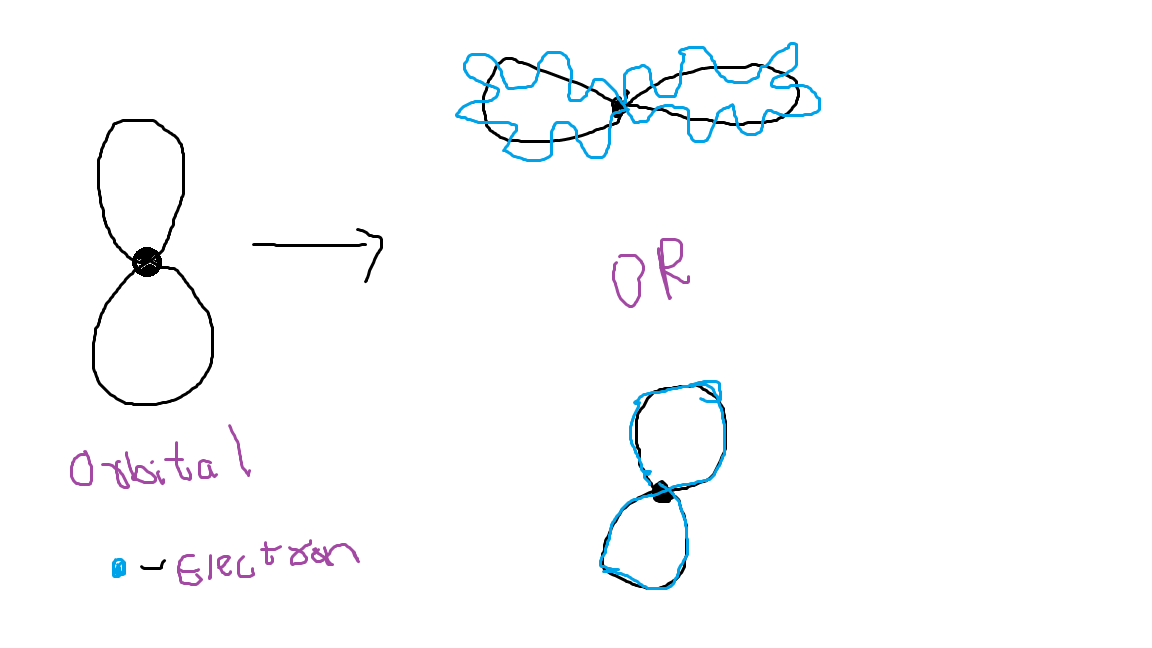enter image description here

Conclusion : Electrons don't have position. They are called particles only because they show nature of particles. Since they don't have position and are not particles, then there is actually no reason to visualize their path. They are neither particles nor waves but a mix of them. (If there is something wrong about the conclusion, then feel free to comment below)

Improve this question
$\endgroup$
3
  • $\begingroup$ I think your conclusion is correct but for the part: „They are waves“. Without knowing it for sure, I am pretty sure that the wave function (which comprises everything we can know about the electron) is not what makes the electron a wave. The electron is as much not a wave as it is not a particle. Depending on the experimental setup it shows aspects of a particle or aspects of a wave. $\endgroup$
    – Hartmut Braun
    Commented Aug 18, 2020 at 6:59
  • $\begingroup$ This explains that the orbit is a standing 3D wave of the electron about the nucleus: en.wikipedia.org/wiki/Atomic_orbital. Thus the electron does not orbit the nucleus like a planet (or particle). The wave function describes the probability of interacting with the electron at a particle point during measurement, not the probability that the electron is at a particular point. $\endgroup$
    – jpf
    Commented Aug 18, 2020 at 11:48
  • $\begingroup$ I think that should fix it @HartmutBraun $\endgroup$
    – Humble_Snowman
    Commented Sep 13, 2020 at 18:51

3 Answers 3

Reset to default
3
$\begingroup$

I wanted to know whether the p-orbital is the wave itself or is it like a racing circuit?

The p-orbital is the wave itself. It is a standing wave that reflects off of the confining potential well of the Coulomb attraction to the nucleus. If you take a cut through the $y,z$ plane, it looks like this:

Mathematica graphics

Mathematica source: Import["http://halirutan.github.io/Mathematica-SE-Tools/decode.m"]["https://i.sstatic.net/SbGmb.png"]

Here the vertical axis is the 'probability amplitude' (i.e. the wavefunction, $\psi(x,y,z)$).

You get the standard peanut shape if you compute the squared-modulus $|\psi(x,y,z)|^2$ and you plot a contour at a fixed value halfway between the minimum and the maximum, either in 2D or 3D.

The "racing circuit" you have drawn has no relation to reality. To the extent that it can be made to work is by having the "racing circuit" go in a straight line up and down the $z$ axis, with a wavelength equal to the length of the circuit. (But that is a fragile mental model, and it is extremely liable to cause additional errors and misconceptions, so use it as sparingly as possible.)

Improve this answer
$\endgroup$
12
  • $\begingroup$ So orbitals are waves?? Correct me if I am wrong but I have been taught that orbitals are the places where electrons reside, ie. maximum probability. So if they are the places with maximum probability of finding an electron then how can they be waves? $\endgroup$
    – Humble_Snowman
    Commented Aug 17, 2020 at 10:58
  • 2
    $\begingroup$ Language can be bent and re-used as required by the context. In 'grown-up' QM, orbitals are waves, period. (They come with uniquely-associated probability distributions and thus with locations of maximal probability, but what they are is waves.) The simplification you have been told is a useful teaching tool, but you need to treat it as such. $\endgroup$
    – Emilio Pisanty
    Commented Aug 17, 2020 at 11:00
  • $\begingroup$ So the pictures of the orbitals s, p and d were a lie? $\endgroup$
    – Humble_Snowman
    Commented Aug 17, 2020 at 11:16
  • $\begingroup$ No, pictures like this one are accurate representations of the probability densities ($|\psi(\mathbf r)|^2$) that arise from the wavefunctions of the orbitals ($\psi(\mathbf r)$). It's just important to pay attention to what's being claimed that they are. $\endgroup$
    – Emilio Pisanty
    Commented Aug 17, 2020 at 12:30
  • 1
    $\begingroup$ @Humble_Snowman they are the equivalent of drawing stick figures in place of a full human form. It’s not a lie, but it’s not the whole truth. $\endgroup$
    – cms
    Commented Aug 17, 2020 at 12:31
2
$\begingroup$

In quantum mechanics electron in general has no position. It is not that we do not know what is the position, it literally has no such property. Kind of like color has no taste. It makes no sense to talk about position of electron (in general), just like it makes no sense to talk about taste of color blue. So the picture of racing electrons is just wrong.

All we can say is what probability is to find electron at certain area. Orbital is simply put just wave function that encodes this probability if it would be filled by some electron.

The pictures of orbitals you can see are just areas of space, where the probability density of finding the electron there is bigger than certain number - if it would be filled.

Answering the comment:

"...electron has no position..." seems to contradict "...we can say the probability to find electron at certain area..."

it only seems that way. It has not position before the collapse of the wave function, but it has immediately after the collapse. The measurement forces the electron to take up the position.

Improve this answer
$\endgroup$
7
  • $\begingroup$ hmm.. so here is a doubt. If the pictures of orbitals I see are just areas of space where prob density of finding the electron are highest then what do the positive and negative lobes of the orbital mean in this context? $\endgroup$
    – Humble_Snowman
    Commented Aug 17, 2020 at 11:15
  • $\begingroup$ @Humble_Snowman As far as I know, these concern phase of the p-orbital when you have two atoms. It has no meaning for orbital by itself, Negative lobes simply mean, the two areas of two orbitals of these two atoms are out of phase. $\endgroup$
    – Umaxo
    Commented Aug 17, 2020 at 11:36
  • 1
    $\begingroup$ @Humble_Snowman The wave function can have positive and negative values, but either way when you take the square modulus you get a real positive number. $\endgroup$
    – Charlie
    Commented Aug 17, 2020 at 11:36
  • $\begingroup$ "...electron has no position..." seems to contradict "...we can say the probability to find electron at certain area..." $\endgroup$
    – jpf
    Commented Aug 17, 2020 at 11:39
  • 1
    $\begingroup$ @jpf it only seems that way. It has not position before the collapse of the wave function, but it has immediately after the collapse. The measurement forces the electron to take up the position. $\endgroup$
    – Umaxo
    Commented Aug 17, 2020 at 11:44
-1
$\begingroup$

We don't have a model of an electron but we know that its charge is essentially concentrated in a point. Its dynamics is however described by a wave, the wave function, which is a solution to Schrodinger's wave equation. For an electron in the coulomb potential of a nucleus this equation has discrete solutions, orbitals, that describe bound states.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.