Some doubts related to interference of light by air wedge

Question

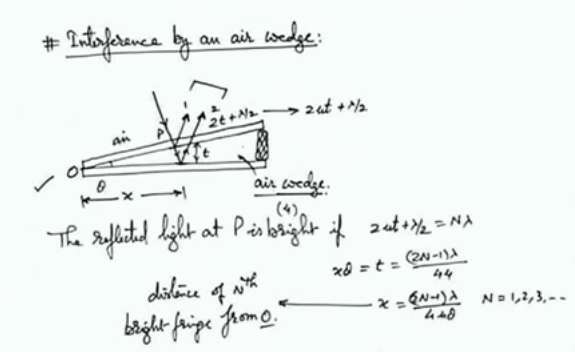
We were taught "Interference by an Air Wedge" today in class. I have attached the image of diagram we were taught and the related formulae. However, I have still some lingering doubts about this topic.

In the air wedge, why don't we consider the rays reflected from the upper part of top plate and bottom part of lower plate ?

When I asked this question in class my teacher said something along the lines of "Due to thin plates for the two waves from upper and lower part phase difference will be π due to which destructive interference take place and hence it is better to ignore these..."

Truly speaking, I could not understand what my teacher meant. Why should those two waves destructively interfere ? Rather I feel that if those two waves were considered along with the assumption that the plates are very thin then the two wave reflected from the upper plates' surfaces surfaces would mutually cancel out due to path difference of $\dfrac{\lambda}{2}$. And, similarly the two waves reflected from either surface of the lower plate would also mutually cancel out due to path difference of $\dfrac{\lambda}{2}$.

So, even after taking the assumption that the thickness of the plates tends to $0$, we see that the waves should also cancel out each other. I don't understand why it is correct to consider only $2$ of the reflected $4$ waves and calculate for bright and dark fringes.

Answer 1

Your concern is valid, but it turns out that accounting for all reflections doesn't essentially change the result.

Let the amplitude of an electromagnetic wave be multiplied by $R_1$ when reflected from air off glass, and $R_2$ when reflected from glass off air. Similarly, let the transmitted amplitudes be multiplied by $T_1$ and $T_2$. These numbers can be found using the Fresnel equations.

Now, the amplitudes of the electric field due to the four reflections are

• top of top panel: $R_1$
• bottom of top panel: $T_1 R_2$
• top of bottom panel: $T_1 T_2 R_1 \times (\text{phase factor})$
• bottom of bottom panel: $T_1 T_2 T_1 R_2 \times (\text{phase factor})$.

where the phase factors are due to traveling the distance $2t$. (The $\pi$ phase factor from some of the reflections is accounted for in the definition of the $R_i$ and $T_i$, some of which are negative.)

If we only include the reflections off the tops of both panels, the total amplitude is $$R_1 + (\text{phase factor}) T_1 T_2 R_1$$ where the phase factor depends on the size of the air wedge. Now, if we include all four reflections, we get a total amplitude of $$(1+T_1 R_2/R_1)\left(R_1 + (\text{phase factor}) T_1 T_2 R_1\right)$$ which is identical up to a constant. Therefore we can ignore two of the reflections, and the result will be essentially the same -- the peaks will be in the same places, even though the overall amplitude will be wrong.

To summarize:

• Your teacher made a small mistake drawing the figure; we should only be considering the reflections from the tops of both wedges, or the bottoms of both, not from one top and one bottom.
• The reason that the reflected waves from the top and bottom of a glass wedge don't cancel out is because $T_1 R_2 \neq R_1$. You are right that there will be destructive interference, but they won't cancel out completely, because their amplitudes aren't the same.
• As you might have noticed, there are actually infinitely many reflections in the problem. However, these all just modify the reflected amplitude by a constant, so we again ignore them.