2
$\begingroup$

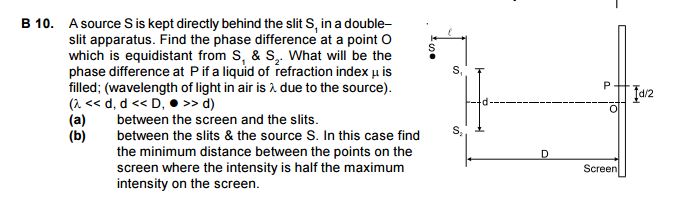
I have attached the question as a picture. I simply don't understand where to begin this question. It is given that $l \gg d$. Why shouldn't the phase difference at $O$ be zero? What exactly is different about this set-up as compared to the 'normal' set-up of Young's Double Slit experiment?

Improve this question
$\endgroup$
6
  • $\begingroup$ Nothing in the question suggests that the phase difference at $O$ is anything but zero... just follow the physics you know. Note that if there is a medium with refractive index >1 between the slits and the screen, the wavelength of the light becomes shorter. That's all you need to know... $\endgroup$
    – Floris
    Commented Jan 14, 2016 at 14:04
  • $\begingroup$ @Floris Well nothing except the answer... The answer given isn't zero. $\endgroup$
    – Gummy bears
    Commented Jan 14, 2016 at 14:08
  • $\begingroup$ Ah - I just noticed that the source is not centered on the line. But the height of the source (offset) is not given... $\endgroup$
    – Floris
    Commented Jan 14, 2016 at 14:09
  • 1
    $\begingroup$ That's not how the diagram is drawn, but you are right, that's what the text says. I hate it when questions are deliberately obfuscated like that. It doesn't aid in understanding. $\endgroup$
    – Floris
    Commented Jan 14, 2016 at 14:15
  • 1
    $\begingroup$ Which makes the height offset $\frac{d}{2}$ $\endgroup$
    – Floris
    Commented Jan 14, 2016 at 14:23

3 Answers 3

Reset to default
2
$\begingroup$

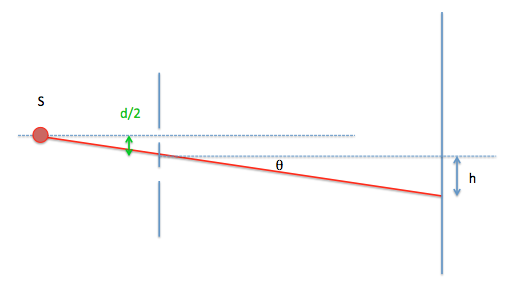
The drawing is a little bit confusing - but the key is that the source $S$ is a relatively long way from the slits (compared to their spacing), and at a height of $\frac{d}{2}$ (from "directly behind $S_1$"). This means you can draw a diagram to calculate the relative path distance between S and each of the two slits. The difference in path length results in a phase difference before the light arrives at the slits - and that phase difference is maintained as you travel from slits to screen.

One way to look at it is to say that the "zero" of the diffraction pattern will be directly in line with the line connecting $S$ to the middle of the slits. If there was just a single large hole between the slits, you would have no problem seeing that this is the case:

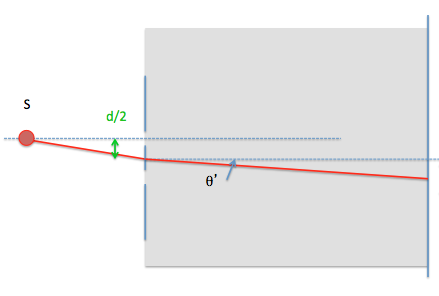
enter image description here

When you add a refractive index, the wavelength of the light is shorter (by a factor $\frac{1}{n}$). It's interesting to note that you can find the "zero" of the diffraction pattern (regardless of whether the refractive medium is in front of the slits or behind) by repeating the above argument: pretend there are no slits but just a single hole, and imagine where the light would go. Snell's law will quickly give you the answer.

enter image description here

I will leave the rest up to you - our homework policy asks us not to give complete answers to this kind of question, but just to explain some of the principles.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Well won't the path difference simply be $\sqrt{l^2 + d^2} - l$? But unfortunately, that's not the answer given... Or am I doing something wrong? $\endgroup$
    – Gummy bears
    Commented Jan 14, 2016 at 15:09
  • $\begingroup$ If the light arriving at a distance $h$ below $O$ has zero phase difference, then the path length difference $\Delta$ at $O$ (for small angles $\theta$) is found from (similar triangles) $\frac{\Delta}{d} = \frac{h}{D} = \frac{d}{2\ell}$. In this, I assume that $\sin\theta = \tan\theta$ which is an OK assumption for small angles. Note that your expression simplifies to the same thing if you do an expansion (noting that $d\ll l$, write $\ell\left(\sqrt{1+\frac{d^2}{\ell^2}}-1\right)\approx \ell(1+\frac{d^2}{2\ell^2} - 1) = \frac{d^2}{2\ell}$ which is what I got the other way... $\endgroup$
    – Floris
    Commented Jan 14, 2016 at 15:15
  • $\begingroup$ I'm not seeing it... Which two triangles are we talking about? $\endgroup$
    – Gummy bears
    Commented Jan 14, 2016 at 15:19
  • $\begingroup$ Triangle made by $h$ and $D$, triangle made by $\frac{d}{2}$ and $\ell$, and triangle made by path difference $\Delta$ and the slit spacing $d$ all have the same angle $\theta$. $\endgroup$
    – Floris
    Commented Jan 14, 2016 at 15:21
  • $\begingroup$ Aha... Now I see it... yes... Thanks for the help! And sorry for being so stupid :) $\endgroup$
    – Gummy bears
    Commented Jan 14, 2016 at 15:29
0
$\begingroup$
  1. A phase difference may also be zero.
  2. Whenever the difference of the distances the beams travel is not a multiple of the wavelength, there is a non-zero phase difference - as simple as that. So if the source is not equidistant from both slits, and the point of measurement is equidistant from the slits, mean you already have a pretty good chance of non-zero phase difference.
Improve this answer
$\endgroup$
0
$\begingroup$

The text states that the source $S$ is placed just behind the slit $S_1$, so it looks to me that the sources $S_1$ and $S_2$ already are out of phase by a factor $\exp(i k d)$. Thus the phase difference in $O$ is $\Phi_O = k d$.

This is different from the usual experiment where $S$ is placed at equal distance from $S_1$ and $S_2$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ What is $k$? And where did you get this answer? $\endgroup$
    – Gummy bears
    Commented Jan 14, 2016 at 14:13
  • $\begingroup$ $k$ is the wavevector : $k = \frac{2 \pi}{\lambda}$, $\lambda$ being the wavelenght of the source. Assume $S$ is at the same position as $S_1$ (as it is directly behind it). If we set the original phase of the light in $S$ to be zero, the phase in $S_1$ is thus zero as well, and the phase in $S_2$ is $k*d$, as the light travelled on a distance $d$ from $S_1$ to $S_2$. $\endgroup$
    – Dimitri
    Commented Jan 14, 2016 at 14:19
  • 1
    $\begingroup$ @D.Labat be careful not to give away too much. We don't want to answer the question for him. $\endgroup$
    – garyp
    Commented Jan 14, 2016 at 14:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.