1
$\begingroup$

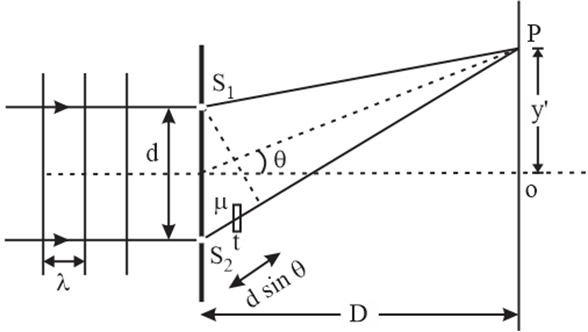
Consider the experimental setup for Young's Double Slit Experiment:

enter image description here

Here there is an angle between the directions of propagation of waves ,i.e., $\angle S_1PS_2$ - the angle between the electric and magnetic field vectors at that point other than the phase difference.

My question is:

Why is this angle never taken into account while analysing this experiment or the diffraction experiments?

Source of the image: https://www.embibe.com/study/effect-of-placing-thin-transparent-film-in-ydse-in-front-of-slits-concept

Improve this question
$\endgroup$
3
  • $\begingroup$ It's already been accounted. We use $\theta$ instead of $\angle S_1PS_2$ because it's easier to find path difference. $\endgroup$
    – Ken
    Commented Nov 12, 2020 at 5:05
  • $\begingroup$ @Ken I meant the angle between the electric and magnetic field vectors at that point other than the phase difference. $\endgroup$
    – Tony Stark
    Commented Nov 12, 2020 at 5:09
  • 1
    $\begingroup$ As distance to screen is very large, the angle will be very small, and Hance the angle between the electric and magnetic field vectors is close to 0. So, the approximation of neglecting that is acceptable. There are many other approximations, that have more significance than this. $\endgroup$
    – Manish S
    Commented Nov 12, 2020 at 6:29

1 Answer 1

Reset to default
2
$\begingroup$

Typically we assume that the distance to the screen is much larger than the width of the slits or the separation of the slits. The light that emerges from the two slits at an angle of theta are almost parallel. Thus, theoretically and approximately there is no angle $S_1 P S_2$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.