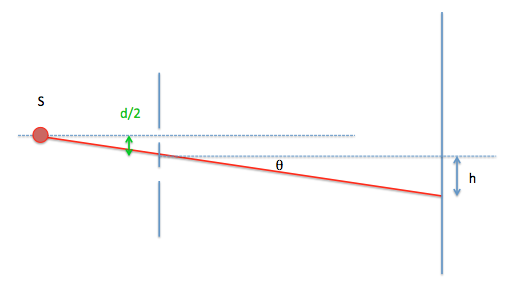
The drawing is a little bit confusing - but the key is that the source $S$ is a relatively long way from the slits (compared to their spacing), and at a height of $\frac{d}{2}$ (from "directly behind $S_1$"). This means you can draw a diagram to calculate the relative path distance between S and each of the two slits. The difference in path length results in a phase difference before the light arrives at the slits - and that phase difference is maintained as you travel from slits to screen.
One way to look at it is to say that the "zero" of the diffraction pattern will be directly in line with the line connecting $S$ to the middle of the slits. If there was just a single large hole between the slits, you would have no problem seeing that this is the case.:
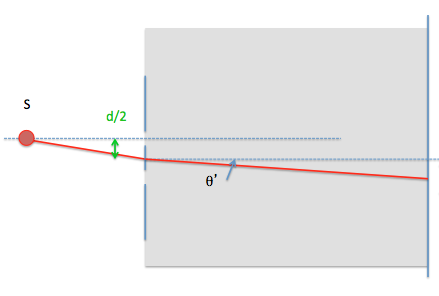
When you add a refractive index, the wavelength of the light is shorter (by a factor $\frac{1}{n}$). It's interesting to note that you can find the "zero" of the diffraction pattern (regardless of whether the refractive medium is in front of the slits or behind) by repeating the above argument: pretend there are no slits but just a single hole, and imagine where the light would go. Snell's law will quickly give you the answer.
I will leave the rest up to you - our homework policy asks us not to give complete answers to this kind of question, but just to explain some of the principles.