3
$\begingroup$

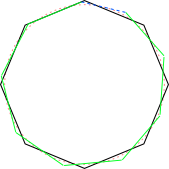
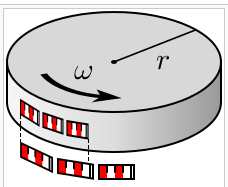
According to Einstein it should be greater than $2 \pi R$ for a co-rotating observer, i.e. $L' = \gamma L$ where $L = 2 \pi R$ in a non-rotating frame and $\gamma$ is the usual Lorentz factor, which would make the spatial geometry hyperbolic - from wikipedia:

Imagine a circle drawn about the origin in the x'y' plane of K' and a diameter of this circle. Imagine, further, that we have given a large number of rigid rods, all equal to each other. We suppose these laid in series along the periphery and the diameter of the circle, at rest relatively to K'. If U is the number of these rods along the periphery, D the number along the diameter, then, if K' does not rotate relatively to K, we shall have $U/D=\pi$. But if K' rotates we get a different result. Suppose that at a definite time t, of K we determine the ends of all the rods. With respect to K all the rods upon the periphery experience the Lorentz contraction, but the rods upon the diameter do not experience this contraction (along their lengths!). It therefore follows that $U/D>\pi$.

On the other hand, there's a nice explanation here on SE that I find intuitively appealing but it concludes the opposite: $L' = L/\gamma$.

So which shall it be?

Due to obvious reasons I put more weight on the first possibility, although I don't actually understand the reasoning, so if someone could describe it in more detail it'd be great.

There's also this paper I've been looking at, which arrives at Einstein's result in a general relativistic framework, but I'd rather just stay at measuring rods and the like if it's possible...

Improve this question
$\endgroup$
4

4 Answers 4

Reset to default
6
$\begingroup$

You can get the result you want by looking at the "circumference" in different ways. In the circle's rest system, there is no ambiguity of circumference. It is the sum of length's of the rods laid out along the circle. This is the total length of black rods in the following picture:

Black rods are inertial, green rods are rotating

Now if you rotate very fast w.r.t. the circle, the rods will seem shorter. This is depicted by the green lines (they actually do not stay straight, but will bend a little, however for the sake of the argument, let's assume they stay nearly straight). The leftmost rod is placed where it was before. The next rod is directly adjacent to that, there no gap that suddenly appears, if you start to rotate. So the second rod is a little off w.r.t. its non-rotating counterpart (keep in mind, that this is just a "snapshot").

If you continue to lay down rods that way, you will run out of green rods before you complete the circle. The missing part is the dashed blue line.

Now you can say: "Well, completing a full circumference means to go around and counting rods. If you count eight rods, you completed the circle." By this argument, the circumference gets smaller, as in the linked SE post.

You could also say: "F**k the number of rods... I have plenty! I will lay down rods until I complete the circle." Then you would count how many rods are needed. Clearly more than before. So, you argue that the circumference has gotten longer. This is what happened in the wikipedia article.

You could say: "Well I want a very rigid definition of circumference! I will just do X and everyone will agree that it measures the circumference by any standard and unambiguously!" You would be wrong.

Rotating systems have a strange property: If you placed many clocks around the circumference of a rotating circle, you cannot synchronize all of them. You could start with one clock, synchronize it with the next and so on. Then, if you come back to your first clock you will see a time difference between the first and the last one, even though you set them all to the same time!

This is why I put the word "snapshot" in quotation marks. There is no "snapshot" of a rotating disk, because there is no way to define simultaneity along the whole circumference. And that is why you can define the circumference however the hell you like.

Improve this answer
$\endgroup$
4
  • $\begingroup$ +1 for the comment on lack of Einstein synchronization of clocks in the rotating frame but there is still a very natural notion of circumference that can be defined. It is that which comes from integrating the "spatial" metric on the quotient manifold obtained by taking Minkowski space-time in the rotating coordinates and quotienting out the events that lie on the same orbit of the time-like Killing field defining the congruence of observers on the disk. The integration through this "spatial" metric gives the hyperbolic geometry of Einstein C.f. arxiv.org/pdf/gr-qc/0309020v1.pdf $\endgroup$
    – FenderLesPaul
    Commented Nov 13, 2014 at 19:31
  • $\begingroup$ @FenderLesPaul: Thanks for the link! It is a bit more than I'm prepared to chew right now, but it's nice to know there is a rigorous way to get the desired result. Will study it in detail... one day. $\endgroup$
    – Rok
    Commented Nov 13, 2014 at 22:29
  • $\begingroup$ @M.Herzkamp: Nice answer! The last paragraph is especialy helpful. :) $\endgroup$
    – Rok
    Commented Nov 13, 2014 at 22:30
  • $\begingroup$ @Rok, here's a much shorter, much more intuitive, and less technical paper: arxiv.org/pdf/1109.2488v2.pdf $\endgroup$
    – FenderLesPaul
    Commented Nov 15, 2014 at 4:00
3
$\begingroup$

You have to realize that a rotating frame of reference is not an inertial frame - and because of this, you can't use the non-rotating frame solution. There is acceleration... so the two frames are not equivalent. The observer at the center of the rotating frame does not experience acceleration, while the one on the circle does. So it's OK that they measure things differently - as indeed they do, as per the linked answer you quoted.

Improve this answer
$\endgroup$
2
  • $\begingroup$ This has nothing to do with GR; it's a purely SR problem, acceleration doesn't change that. $\endgroup$
    – FenderLesPaul
    Commented Nov 13, 2014 at 19:27
  • $\begingroup$ @FenderLesPaul - point about SR vs GR taken. Edited. Thanks for pointing that out. $\endgroup$
    – Floris
    Commented Nov 13, 2014 at 20:10
0
$\begingroup$

In the first case the reference frame is fixed to the stationary center of disk rotation with velocity $\omega$. enter image description here

Then the magnitude of the velocity of any point in the circumference of the disk is $\omega R$. So the circumference will undergo Lorentz contraction by factor $\gamma ={\sqrt{1-{\frac{{{\omega}^2 R}^2}{c^2}}}}$ So here the length moving with respect to the reference frame of observer.

In the 2nd case the polygon is on the earth, reference frame, and it is observed from the moving spaceship i.e. from a moving frame. That is these two case is reciprocal to each other and hence the observed length is also difference. For first case: $L'=\gamma L$ and 2nd case $L'={\frac{L}{\gamma}}$ And both are write because observer and object place are different.

Improve this answer
$\endgroup$
0
$\begingroup$

According to Einstein [the circumference] should be greater than $2 \pi R$ for a co-rotating observer … On the other hand, there's a nice explanation here on SE … but it concludes the opposite: $L' = L/\gamma$.

The Lorentz factor ($\gamma$) starts from $1$ at rest and decreases towards zero the faster you go (reaching $0$ only at the speed of light, so never). Being at the denominator in the equation, the final circumference will be greater the smaller the Lorentz factor is, i.e. the faster the body spins.

\begin{equation}L^\prime = \frac{L}{\gamma} = \frac{2\pi R}{\sqrt{1-\frac{v^2}{c^2}}}\end{equation}

Or, alternatively,

\begin{equation}L^\prime = \frac{L}{\gamma} = \frac{2\pi R}{\sqrt{1-\frac{R^2\omega^2}{c^2}}}\end{equation}

PSR J1748−2446ad is the fastest neutron star known; if you walked around its equator you would walk three kilometres more than you had predicted by staring at it from Earth.

This implies the impossibility of rigid rotation. Neutron stars are not rigid, and so they are free to deform to fit for the larger circumference.

More on this topic:

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.